proofs

164 Pigeon-hole and double counting
For the proof we set N = {0, 1, . . ., n} and R = {0, 1, . . ., n − 1}. Consider
the map f : N → R, where f(m) is the remainder of a 1 + . . . + a m
upon division by n. Since |N| = n + 1 > n = |R|, it follows that there are
two sums a 1 + . . . + a k , a 1 + . . . + a l (k < l) with the same remainder,
where the first sum may be the empty sum denoted by 0. It follows that
l∑
i=k+1
a i =
l∑
a i −
i=1
k∑
i=1
a i
has remainder 0 — end of proof.
□
Let us turn to the second principle: counting in two ways. By this we mean
the following.
Double counting.
Suppose that we are given two finite sets R and C and a subset
S ⊆ R ×C. Whenever (p, q) ∈ S, then we say p and q are incident.
If r p denotes the number of elements that are incident to p ∈ R,
and c q denotes the number of elements that are incident to q ∈ C,
then ∑
c q . (3)
p∈R
r p = |S| = ∑ q∈C
Again, there is nothing to prove. The first sum classifies the pairs in S
according to the first entry, while the second sum classifies the same pairs
according to the second entry.
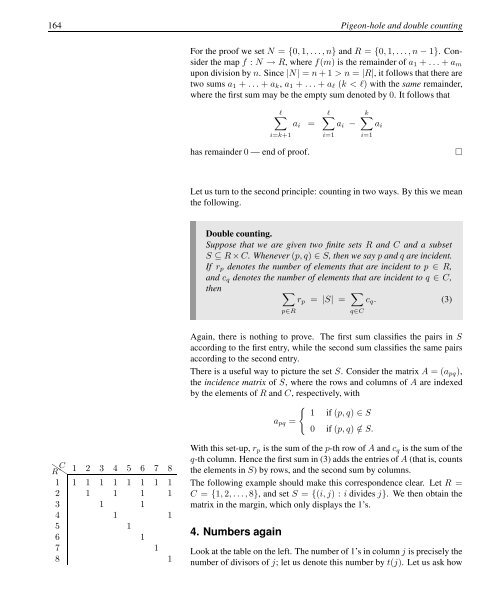
There is a useful way to picture the set S. Consider the matrix A = (a pq ),
the incidence matrix of S, where the rows and columns of A are indexed
by the elements of R and C, respectively, with
{
1 if (p, q) ∈ S
a pq =
0 if (p, q) /∈ S.
◗ R◗
1 2 3 4 5 6 7 8
1 1 1 1 1 1 1 1 1
2 1 1 1 1
3 1 1
4 1 1
5 1
6 1
7 1
8 1
With this set-up, r p is the sum of the p-th row of A and c q is the sum of the
q-th column. Hence the first sum in (3) adds the entries of A (that is, counts
the elements in S) by rows, and the second sum by columns.
The following example should make this correspondence clear. Let R =
C = {1, 2, . . .,8}, and set S = {(i, j) : i divides j}. We then obtain the
matrix in the margin, which only displays the 1’s.
4. Numbers again
Look at the table on the left. The number of 1’s in column j is precisely the
number of divisors of j; let us denote this number by t(j). Let us ask how