proofs

86 Touching simplices
l
l
l ′
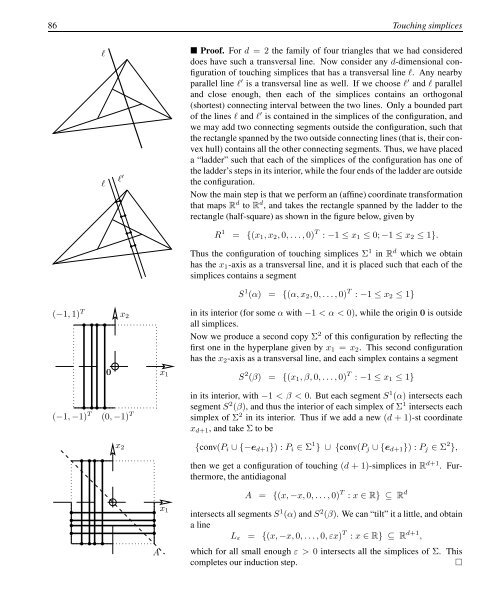
Proof. For d = 2 the family of four triangles that we had considered
does have such a transversal line. Now consider any d-dimensional configuration
of touching simplices that has a transversal line l. Any nearby
parallel line l ′ is a transversal line as well. If we choose l ′ and l parallel
and close enough, then each of the simplices contains an orthogonal
(shortest) connecting interval between the two lines. Only a bounded part
of the lines l and l ′ is contained in the simplices of the configuration, and
we may add two connecting segments outside the configuration, such that
the rectangle spanned by the two outside connecting lines (that is, their convex
hull) contains all the other connecting segments. Thus, we have placed
a “ladder” such that each of the simplices of the configuration has one of
the ladder’s steps in its interior, while the four ends of the ladder are outside
the configuration.
Now the main step is that we perform an (affine) coordinate transformation
that maps R d to R d , and takes the rectangle spanned by the ladder to the
rectangle (half-square) as shown in the figure below, given by
(−1, 1) T x 2 in its interior (for some α with −1 < α < 0), while the origin 0 is outside
R 1 = {(x 1 , x 2 , 0, . . .,0) T : −1 ≤ x 1 ≤ 0; −1 ≤ x 2 ≤ 1}.
Thus the configuration of touching simplices Σ 1 in R d which we obtain
has the x 1 -axis as a transversal line, and it is placed such that each of the
simplices contains a segment
S 1 (α) = {(α, x 2 , 0, . . .,0) T : −1 ≤ x 2 ≤ 1}
all simplices.
Now we produce a second copy Σ 2 of this configuration by reflecting the
first one in the hyperplane given by x 1 = x 2 . This second configuration
has the x 2 -axis as a transversal line, and each simplex contains a segment
0 x 1
S 2 (β) = {(x 1 , β, 0, . . .,0) T : −1 ≤ x 1 ≤ 1}
(−1, −1) T
(0, −1) T
x 2
in its interior, with −1 < β < 0. But each segment S 1 (α) intersects each
segment S 2 (β), and thus the interior of each simplex of Σ 1 intersects each
simplex of Σ 2 in its interior. Thus if we add a new (d + 1)-st coordinate
x d+1 , and take Σ to be
{conv(P i ∪ {−e d+1 }) : P i ∈ Σ 1 } ∪ {conv(P j ∪ {e d+1 }) : P j ∈ Σ 2 },
A = {(x, −x, 0, . . . ,0) T : x ∈ R} ⊆ R d
A
then we get a configuration of touching (d + 1)-simplices in R d+1 . Fur-
thermore, the antidiagonal
x 1
intersects all segments S 1 (α) and S 2 (β). We can “tilt” it a little, and obtain
a line
L ε = {(x, −x, 0, . . .,0, εx) T : x ∈ R} ⊆ R d+1 ,
which for all small enough ε > 0 intersects all the simplices of Σ. This
completes our induction step.
□