proofs

64 Lines in the plane, and decompositions of graphs
However, it was shown by Coxeter that the order axioms will suffice for
a proof of the Sylvester–Gallai theorem. Thus one can devise a proof that
does not use any metric properties — see also the proof that we will give in
Chapter 12, using Euler’s formula.
The Sylvester–Gallai theorem directly implies another famous result on
points and lines in the plane, due to Paul Erdős and Nicolaas G. de Bruijn.
But in this case the result holds more generally for arbitrary point-line
systems, as was observed already by Erdős and de Bruijn. We will discuss
the more general result in a moment.
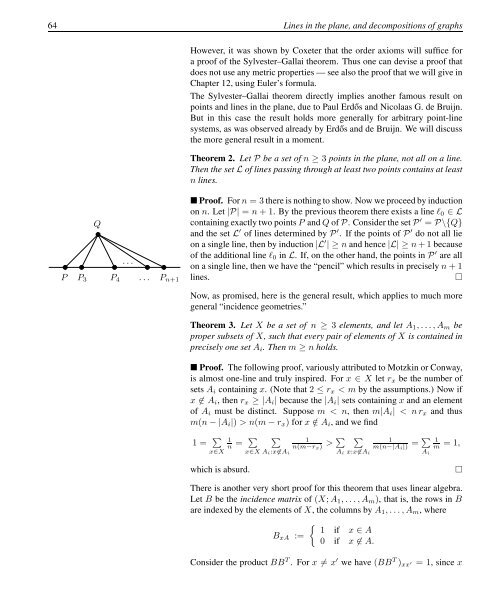
Theorem 2. Let P be a set of n ≥ 3 points in the plane, not all on a line.
Then the set L of lines passing through at least two points contains at least
n lines.
Q
. . .
P P 3 P 4 . . .
P n+1
Proof. For n = 3 there is nothing to show. Now we proceed by induction
on n. Let |P| = n + 1. By the previous theorem there exists a line l 0 ∈ L
containing exactly two points P and Q of P. Consider the set P ′ = P\{Q}
and the set L ′ of lines determined by P ′ . If the points of P ′ do not all lie
on a single line, then by induction |L ′ | ≥ n and hence |L| ≥ n +1 because
of the additional line l 0 in L. If, on the other hand, the points in P ′ are all
on a single line, then we have the “pencil” which results in precisely n + 1
lines.
□
Now, as promised, here is the general result, which applies to much more
general “incidence geometries.”
Theorem 3. Let X be a set of n ≥ 3 elements, and let A 1 , . . .,A m be
proper subsets of X, such that every pair of elements of X is contained in
precisely one set A i . Then m ≥ n holds.
Proof. The following proof, variously attributed to Motzkin or Conway,
is almost one-line and truly inspired. For x ∈ X let r x be the number of
sets A i containing x. (Note that 2 ≤ r x < m by the assumptions.) Now if
x ∉ A i , then r x ≥ |A i | because the |A i | sets containing x and an element
of A i must be distinct. Suppose m < n, then m|A i | < n r x and thus
m(n − |A i |) > n(m − r x ) for x /∈ A i , and we find
1 = ∑
x∈X
1
n = ∑
x∈X
∑
1
n(m−r > ∑ x)
A i:x∉A i A i
∑
1
m(n−|A = ∑ 1
i|)
x:x∉A i A i
m = 1,
which is absurd.
□
There is another very short proof for this theorem that uses linear algebra.
Let B be the incidence matrix of (X; A 1 , . . . , A m ), that is, the rows in B
are indexed by the elements of X, the columns by A 1 , . . . , A m , where
B xA :=
{ 1 if x ∈ A
0 if x ∉ A.
Consider the product BB T . For x ≠ x ′ we have (BB T ) xx ′ = 1, since x