proofs

Cauchy’s rigidity theorem 83
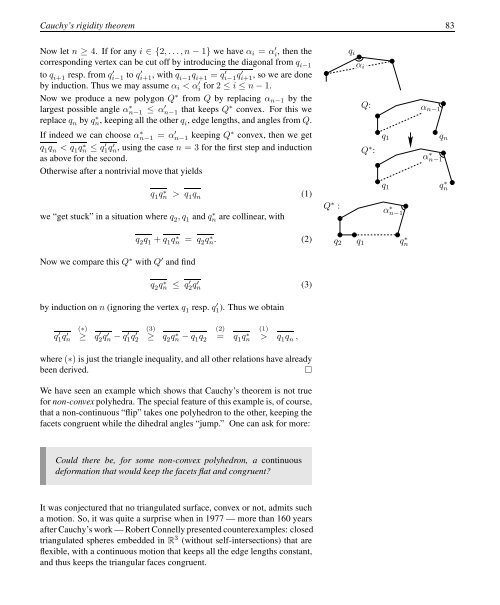
Now let n ≥ 4. If for any i ∈ {2, . . ., n − 1} we have α i = α ′ i , then the
q
corresponding vertex can be cut off by introducing the diagonal from q i−1
to q i+1 resp. from q i−1 ′ to q′ i+1 , with q i−1 q i+1 = q′ i−1 q′ i+1 , so we are done
by induction. Thus we may assume α i < α ′ i for 2 ≤ i ≤ n − 1.
Now we produce a new polygon Q ∗ from Q by replacing α n−1 by the
largest possible angle α ∗ n−1 ≤ α′ n−1 that keeps Q∗ convex. For this we
replace q n by qn, ∗ keeping all the other q i , edge lengths, and angles from Q.
i
α i
Q:
α n−1
If indeed we can choose α ∗ n−1 = α ′ n−1 keeping Q ∗ convex, then we get
q 1 q n < q 1 qn ∗ ≤ q′ 1 q′ n , using the case n = 3 for the first step and induction
as above for the second.
Otherwise after a nontrivial move that yields
Q ∗ :
q 1
α ∗ n−1
q n
q 1 q ∗ n > q 1 q n (1)
we “get stuck” in a situation where q 2 , q 1 and qn ∗ are collinear, with
Q ∗ :
q 1
α ∗ n−1
q ∗ n
q 2 q 1 + q 1 qn ∗ = q 2 q∗ n . (2) q 2
Now we compare this Q ∗ with Q ′ and find
q 2 qn ∗ ≤ q 2 ′ q′ n (3)
by induction on n (ignoring the vertex q 1 resp. q 1 ′ ). Thus we obtain
q 1
q ∗ n
q ′ 1 q′ n
(∗)
≥ q ′ 2 q′ n − q ′ 1 q′ 2
(3)
≥ q 2 qn ∗ (2)
− q 1 q 2 = q 1 qn
∗
(1)
> q 1 q n ,
where (∗) is just the triangle inequality, and all other relations have already
been derived.
□
We have seen an example which shows that Cauchy’s theorem is not true
for non-convex polyhedra. The special feature of this example is, of course,
that a non-continuous “flip” takes one polyhedron to the other, keeping the
facets congruent while the dihedral angles “jump.” One can ask for more:
Could there be, for some non-convex polyhedron, a continuous
deformation that would keep the facets flat and congruent?
It was conjectured that no triangulated surface, convex or not, admits such
a motion. So, it was quite a surprise when in 1977 — more than 160 years
after Cauchy’s work — Robert Connelly presented counterexamples: closed
triangulated spheres embedded in R 3 (without self-intersections) that are
flexible, with a continuous motion that keeps all the edge lengths constant,
and thus keeps the triangular faces congruent.