Connes-Chern Character for Manifolds with Boundary and ETA ...
Connes-Chern Character for Manifolds with Boundary and ETA ...
Connes-Chern Character for Manifolds with Boundary and ETA ...
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
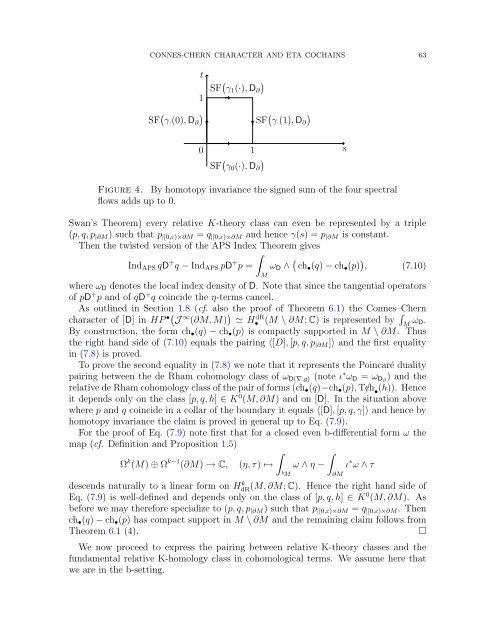
CONNES-CHERN CHARACTER AND <strong>ETA</strong> COCHAINS 63<br />
t<br />
1<br />
SF ( γ·(0), D ∂<br />
)<br />
SF ( γ 1 (·), D ∂<br />
)<br />
SF ( γ·(1), D ∂<br />
)<br />
0 1<br />
SF ( )<br />
γ 0 (·), D ∂<br />
s<br />
Figure 4. By homotopy invariance the signed sum of the four spectral<br />
flows adds up to 0.<br />
Swan’s Theorem) every relative K-theory class can even be represented by a triple<br />
(p, q, p |∂M ) such that p |[0,ε)×∂M = q |[0,ε)×∂M <strong>and</strong> hence γ(s) = p |∂M is constant.<br />
Then the twisted version of the APS Index Theorem gives<br />
∫<br />
Ind APS qD + q − Ind APS pD + p = ω D ∧ ( ch • (q) − ch • (p) ) , (7.10)<br />
where ω D denotes the local index density of D. Note that since the tangential operators<br />
of pD + p <strong>and</strong> of qD + q coincide the η-terms cancel.<br />
As outlined in Section 1.8 (cf. also the proof of Theorem 6.1) the <strong>Connes</strong>–<strong>Chern</strong><br />
character of [D] in HP •( J ∞ (∂M, M) ) ≃ H• dR (M \ ∂M; C) is represented by ∫ ω M D.<br />
By construction, the <strong>for</strong>m ch • (q) − ch • (p) is compactly supported in M \ ∂M. Thus<br />
the right h<strong>and</strong> side of (7.10) equals the pairing 〈[D], [p, q, p |∂M ]〉 <strong>and</strong> the first equality<br />
in (7.8) is proved.<br />
To prove the second equality in (7.8) we note that it represents the Poincaré duality<br />
pairing between the de Rham cohomology class of ω D(∇,g) (note ι ∗ ω D = ω D∂ ) <strong>and</strong> the<br />
relative de Rham cohomology class of the pair of <strong>for</strong>ms (ch • (q)−ch • (p), T/ch •<br />
(h)). Hence<br />
it depends only on the class [p, q, h] ∈ K 0 (M, ∂M) <strong>and</strong> on [D]. In the situation above<br />
where p <strong>and</strong> q coincide in a collar of the boundary it equals 〈[D], [p, q, γ]〉 <strong>and</strong> hence by<br />
homotopy invariance the claim is proved in general up to Eq. (7.9).<br />
For the proof of Eq. (7.9) note first that <strong>for</strong> a closed even b-differential <strong>for</strong>m ω the<br />
map (cf. Definition <strong>and</strong> Proposition 1.5)<br />
∫ ∫<br />
Ω k (M) ⊕ Ω k−1 (∂M) → C, (η, τ) ↦→ ω ∧ η − ι ∗ ω ∧ τ<br />
descends naturally to a linear <strong>for</strong>m on HdR k (M, ∂M; C). Hence the right h<strong>and</strong> side of<br />
Eq. (7.9) is well-defined <strong>and</strong> depends only on the class of [p, q, h] ∈ K 0 (M, ∂M). As<br />
be<strong>for</strong>e we may there<strong>for</strong>e specialize to (p, q, p |∂M ) such that p |[0,ε)×∂M = q |[0,ε)×∂M . Then<br />
ch • (q) − ch • (p) has compact support in M \ ∂M <strong>and</strong> the remaining claim follows from<br />
Theorem 6.1 (4).<br />
□<br />
We now proceed to express the pairing between relative K-theory classes <strong>and</strong> the<br />
fundamental relative K-homology class in cohomological terms. We assume here that<br />
we are in the b-setting.<br />
M<br />
b M<br />
∂M