Introduction to Krylov subspace methods - IMAGe

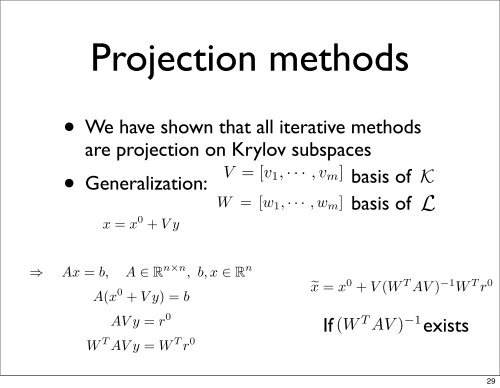
(√w understand that CG/SDesc./Richardson are projection meth-2 λ 2 λ − 2 κ + 1T k+1 (1 + 2η) ≥ 1 λ max + λ√ min2‖x k+1 λmax − √ = 1 max − (p.86 λ min in [2]) We2nowκ +understand1thatκ − 1√ CG/SDesc./Richarλ min 2 κ + − 1 x‖ A ≤ 2 √(√ ods on ) (√ )Projectionk+1a Krylov space. In general, (p.86 in let[2]) V = We [vκ −methods1 , now · · · , vundm ] bκ − 1k+1‖x k+1 − x‖ A ≤ 21‖x k+1 √ {v ‖x− x‖ A ≤ 2 (√ −√ x 0 i } m i=1 are a basis ‖ A . of ) space ‖x K − and onx 0 k+1 5.3a‖ W KrylovA .= [w Proje1, space. · · · , w m ] Iκ + 1‖x k+1 space − x‖ L. + 5.3 A ≤Then κ − 12 √if the approximateProjection‖x {v− i } x m0 solutionmethods‖ A . is written asi=1 are a basis of spκ + 1n methodsspace L. Thenrojection methods(p.86 x = x 0 if the app+ in V y[2]) WProjection • We have methods shown (p.86that in [2]) all iterative We now methods understand that C[2]) We noware understand projection ods that CG/SDesc./Richardson Krylov a Krylov subspaces space.odsInare general,on a Kryloace. In general, let V = [v projection let Vmin [2]) We now understand 1 , · · · , vthat m ] be an n ×CG/SDesc./Richardson ⇒m matrixAx =whereb, are A projection meKrylov•space. Generalization:In general, {v ilet } [v ] be an n × m matrix wn a Krylov space. In general, let i=1 V = are [v 1 , a· · basis · , v m ] be of {van space i } m ∈ R n×n , b, x ∈of space K and W = [w 1 , · · · , w m ] a (A-orthogonal) basis ofA(x 0 n + of × i=1 Vmy) Kmatrix = are and ba Wwharee approximatea basis of space K and [w 1 , ] a (A-orthogonal) ba=1 are a basis solution of space is written K space and asW L. = Then [w 1, · · · if , wthe m ] a basis approximate space (A-orthogonal) AVofy = L. r 0 Then solut basis ⇒Then if the approximate x = x 0 + V y solution is written asW T AV y = W T r 0x =x = x 0 + V y⇒ Ax = b, A ∈IfR(W n×n T ,AVb, x) −1 ∈ Rexists, nA(x 0 ∴ ˜x = x 0 + V (W T AV ) −1 W T r 0+ V y) = b⇒⇒ Ax Ax = b, b, A ∈ R n×n , b, x ∈ R nAV y = r 0n ⇒ Ax = b, AConditions forW T AV y = W T rA(x 0 A(x 0 0 existence of (W IfT (W AV T ) −1 AV : ) −1 exists,+ V y) = bA(x∴0 +(i) A is positive AV y = definite rAV 0 and L = Ke L. Then if the approximate solution is written asts,AV 29