differential equation

More documents

Recommendations

Info

468 DIFFERENTIAL EQUATIONS For line 6, x 1 = 2.0 y P1 = y 0 + h(y ′ ) 0 = 5.85212176 + 0.2(2.54787824) = 6.361697408 y C1 = y 0 + 1 2 h[(y′ ) 0 + (3 + 3x 1 − y P1 )] = 5.85212176 + 1 2 (0.2)[2.54787824 = 6.370739843 + (3 + 3(2.0) − 6.361697408)] Problem 6. Using the integrating factor method the solution of the differential equation = 3(1 + x) − y of Problem 5 is dy dx y = 3x + e 1 − x . When x = 1.6, compare the accuracy, correct to 3 decimal places, of the Euler and the Euler-Cauchy methods. When x = 1.6, y = 3x + e 1−x = 3(1.6) + e 1−1.6 = 4.8 + e −0.6 = 5.348811636. From Table 49.1, page 461, by Euler’s method, when x = 1.6, y = 5.312 % error in the Euler method ( ) 5.348811636 − 5.312 = × 100% 5.348811636 = 0.688% From Table 49.11 of Problem 5, by the Euler-Cauchy method, when x = 1.6, y = 5.351368 % error in the Euler-Cauchy method ( ) 5.348811636 − 5.351368 = × 100% 5.348811636 = −0.048% The Euler-Cauchy method is seen to be more accurate than the Euler method when x = 1.6. Now try the following exercise. 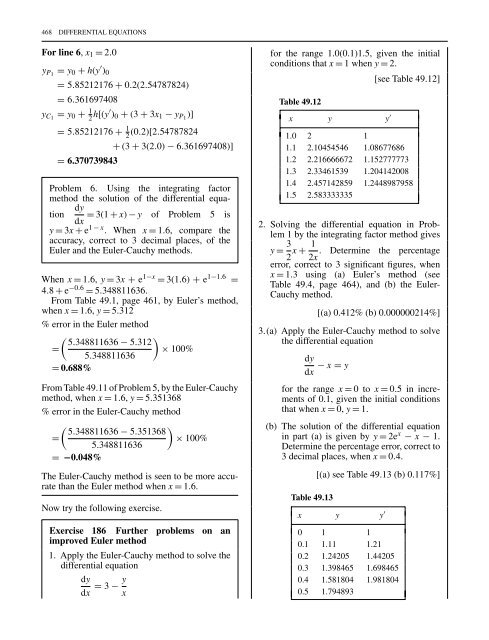Exercise 186 Further problems on an improved Euler method 1. Apply the Euler-Cauchy method to solve the differential equation dy dx = 3 − y x for the range 1.0(0.1)1.5, given the initial conditions that x = 1 when y = 2. [see Table 49.12] Table 49.12 x y y ′ 1.0 2 1 1.1 2.10454546 1.08677686 1.2 2.216666672 1.152777773 1.3 2.33461539 1.204142008 1.4 2.457142859 1.2448987958 1.5 2.583333335 2. Solving the differential equation in Problem 1 by the integrating factor method gives y = 3 2 x + 1 . Determine the percentage 2x error, correct to 3 significant figures, when x = 1.3 using (a) Euler’s method (see Table 49.4, page 464), and (b) the Euler- Cauchy method. [(a) 0.412% (b) 0.000000214%] 3.(a) Apply the Euler-Cauchy method to solve the differential equation dy dx − x = y for the range x = 0tox = 0.5 in increments of 0.1, given the initial conditions that when x = 0, y = 1. (b) The solution of the differential equation in part (a) is given by y = 2e x − x − 1. Determine the percentage error, correct to 3 decimal places, when x = 0.4. Table 49.13 [(a) see Table 49.13 (b) 0.117%] x y y ′ 0 1 1 0.1 1.11 1.21 0.2 1.24205 1.44205 0.3 1.398465 1.698465 0.4 1.581804 1.981804 0.5 1.794893
NUMERICAL METHODS FOR FIRST ORDER DIFFERENTIAL EQUATIONS 469 4. Obtain a numerical solution of the differential equation 1 dy x dx + 2y = 1 using the Euler-Cauchy method in the range x = 0(0.2)1.0, given the initial conditions that x = 0 when y = 1. Table 49.14 x y y ′ [see Table 49.14] 0 1 0 0.2 0.99 −0.196 0.4 0.958336 −0.3666688 0.6 0.875468851 −0.450562623 0.8 0.784755575 −0.45560892 1.0 0.700467925 49.5 The Runge-Kutta method The Runge-Kutta method for solving first order differential equations is widely used and provides a high degree of accuracy. Again, as with the two previous methods, the Runge-Kutta method is a step-by-step process where results are tabulated for a range of values of x. Although several intermediate calculations are needed at each stage, the method is fairly straightforward. The 7 step procedure for the Runge-Kutta method, without proof, is as follows: To solve the differential equation dy = f (x, y)given dx the initial condition y = y 0 at x = x 0 for a range of values of x = x 0 (h)x n : 1. Identify x 0 , y 0 and h, and values of x 1 , x 2 , x 3 , .... 2. Evaluate k 1 = f(x n , y n ) starting with n = 0 ( 3. Evaluate k 2 = f x n + h 2 , y n + h ) 2 k 1 4. Evaluate k 3 = f ( x n + h 2 , y n + h ) 2 k 2 5. Evaluate k 4 = f (x n + h, y n + hk 3 ) 6. Use the values determined from steps 2 to 5 to evaluate: y n+1 = y n + h 6 {k 1 + 2k 2 + 2k 3 + k 4 } 7. Repeat steps 2 to 6 for n = 1, 2, 3, ... Thus, step 1 is given, and steps 2 to 5 are intermediate steps leading to step 6. It is usually most convenient to construct a table of values. The Runge-Kutta method is demonstrated in the following worked problems. Problem 7. Use the Runge-Kutta method to solve the differential equation: dy dx = y − x in the range 0(0.1)0.5, given the initial conditions that at x = 0, y = 2. Using the above procedure: 1. x 0 = 0, y 0 = 2 and since h = 0.1, and the range is from x = 0tox = 0.5, then x 1 = 0.1, x 2 = 0.2, x 3 = 0.3, x 4 = 0.4, and x 5 = 0.5 Let n = 0 to determine y 1 : 2. k 1 = f (x 0 , y 0 ) = f (0, 2); since dy = y − x, f (0, 2) = 2 − 0 = 2 dx ( 3. k 2 = f x 0 + h 2 , y 0 + h ) 2 k 1 ( = f 0 + 0.1 ) 0.1 ,2+ 2 2 (2) = f (0.05, 2.1) = 2.1 − 0.05 = 2.05 ( 4. k 3 = f x 0 + h 2 , y 0 + h ) 2 k 2 ( = f 0 + 0.1 ) 0.1 ,2+ 2 2 (2.05) = f (0.05, 2.1025) = 2.1025 − 0.05 = 2.0525 5. k 4 = f (x 0 + h, y 0 + hk 3 ) = f (0 + 0.1, 2 + 0.1(2.0525)) = f (0.1, 2.20525) = 2.20525 − 0.1 = 2.10525 I
Page 1 and 2: Differential equations 46 Solution
Page 3 and 4: SOLUTION OF FIRST ORDER DIFFERENTIA
Page 5 and 6: α is the temperature coefficient o
Page 7 and 8: SOLUTION OF FIRST ORDER DIFFERENTIA
Page 9 and 10: Differential equations 47 Homogeneo
Page 11 and 12: HOMOGENEOUS FIRST ORDER DIFFERENTIA
Page 13 and 14: Differential equations 48 Linear fi
Page 15 and 16: Hence from equation (4): ye x = xe
Page 17 and 18: LINEAR FIRST ORDER DIFFERENTIAL EQU
Page 19 and 20: NUMERICAL METHODS FOR FIRST ORDER D
Page 25: NUMERICAL METHODS FOR FIRST ORDER D
Page 33 and 34: Differential equations 50 Second or
Page 35 and 36: SECOND ORDER DIFFERENTIAL EQUATIONS
Page 37 and 38: When thus t = 0, dV dt = 3ω, 3ω =
Page 39 and 40: Differential equations 51 Second or
Page 49 and 50: Differential equations 52 Power ser
Page 51 and 52: POWER SERIES METHODS OF SOLVING ORD
Page 71 and 72: AN INTRODUCTION TO PARTIAL DIFFEREN
Page 77 and 78:
AN INTRODUCTION TO PARTIAL DIFFEREN
Page 79 and 80:
Page 81 and 82:
Page 83:
Differential equations Assignment 1
show all

differential equation

Create successful ePaper yourself

Delete template?

Save as template?