differential equation
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
446 DIFFERENTIAL EQUATIONS<br />
Find the <strong>equation</strong> of the curve if it passes<br />
through the point ( 1, 1 )<br />
3 .<br />
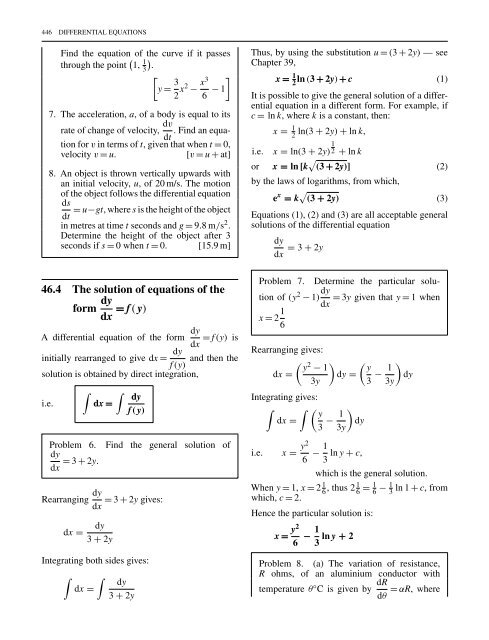
[<br />
y = 3 ]<br />
2 x2 − x3<br />
6 − 1<br />
7. The acceleration, a, of a body is equal to its<br />
rate of change of velocity, dv . Find an <strong>equation</strong><br />
for v in terms of t, given that when t = 0,<br />
dt<br />
velocity v = u.<br />
[v = u + at]<br />
8. An object is thrown vertically upwards with<br />
an initial velocity, u, of 20 m/s. The motion<br />
of the object follows the <strong>differential</strong> <strong>equation</strong><br />
ds<br />
= u−gt, where s is the height of the object<br />
dt<br />
in metres at time t seconds and g = 9.8m/s 2 .<br />
Determine the height of the object after 3<br />
seconds if s = 0 when t = 0. [15.9 m]<br />
46.4 The solution of <strong>equation</strong>s of the<br />
form dy<br />
dx = f ( y)<br />
A <strong>differential</strong> <strong>equation</strong> of the form dy = f (y) is<br />
dx<br />
initially rearranged to give dx = dy and then the<br />
f (y)<br />
solution is obtained by direct integration,<br />
i.e.<br />
∫<br />
∫<br />
dx =<br />
dy<br />
f ( y)<br />
Problem 6. Find the general solution of<br />
dy<br />
dx = 3 + 2y.<br />
Rearranging dy = 3 + 2y gives:<br />
dx<br />
dx =<br />
dy<br />
3 + 2y<br />
Integrating both sides gives:<br />
∫<br />
∫<br />
dx =<br />
dy<br />
3 + 2y<br />
Thus, by using the substitution u = (3 + 2y) — see<br />
Chapter 39,<br />
x =<br />
2 1 ln (3 + 2y) + c (1)<br />
It is possible to give the general solution of a <strong>differential</strong><br />
<strong>equation</strong> in a different form. For example, if<br />
c = ln k, where k is a constant, then:<br />
x =<br />
2 1 ln(3 + 2y) + ln k,<br />
i.e. x = ln(3 + 2y) 2 1 + ln k<br />
or x = ln [k √ (3 + 2y)] (2)<br />
by the laws of logarithms, from which,<br />
e x = k √ (3 + 2y) (3)<br />
Equations (1), (2) and (3) are all acceptable general<br />
solutions of the <strong>differential</strong> <strong>equation</strong><br />
dy<br />
dx = 3 + 2y<br />
Problem 7. Determine the particular solution<br />
of (y 2 − 1) dy = 3y given that y = 1 when<br />
dx<br />
x = 2 1 6<br />
Rearranging gives:<br />
( y 2 − 1<br />
dx =<br />
3y<br />
Integrating gives:<br />
∫<br />
dx =<br />
) ( y<br />
dy =<br />
3 − 1 )<br />
dy<br />
3y<br />
∫ ( y<br />
3 − 1 3y<br />
)<br />
dy<br />
i.e. x = y2<br />
6 − 1 ln y + c,<br />
3<br />
which is the general solution.<br />
When y = 1, x = 2 1 6 , thus 2 1 6 = 1 6 − 1 3<br />
ln 1 + c, from<br />
which, c = 2.<br />
Hence the particular solution is:<br />
x = y2<br />
6 − 1 3 ln y + 2<br />
Problem 8. (a) The variation of resistance,<br />
R ohms, of an aluminium conductor with<br />
temperature θ ◦ C is given by dR = αR, where<br />
dθ