General Computer Science 320201 GenCS I & II Lecture ... - Kwarc

tationally loaded). We just assume the contrary, i.e. that there is a minimal polynomial p that
contains a non-prime-implicant monomial Mk, then we can decrease the cost of the of p while still
inducing the given function f. So p was not minimal which shows the assertion.
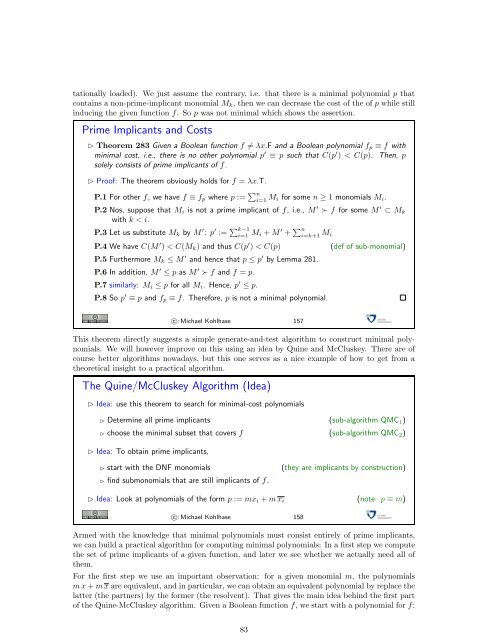
Prime Implicants and Costs
Theorem 283 Given a Boolean function f = λx.F and a Boolean polynomial fp ≡ f with
minimal cost, i.e., there is no other polynomial p ′ ≡ p such that C(p ′ ) < C(p). Then, p
solely consists of prime implicants of f.
Proof: The theorem obviously holds for f = λx.T.
P.1 For other f, we have f ≡ fp where p := n
i=1 Mi for some n ≥ 1 monomials Mi.
P.2 Nos, suppose that Mi is not a prime implicant of f, i.e., M ′ ≻ f for some M ′ ⊂ Mk
with k < i.
P.3 Let us substitute Mk by M ′ : p ′ := k−1
i=1 Mi + M ′ + n
i=k+1 Mi
P.4 We have C(M ′ ) < C(Mk) and thus C(p ′ ) < C(p) (def of sub-monomial)
P.5 Furthermore Mk ≤ M ′ and hence that p ≤ p ′ by Lemma 281.
P.6 In addition, M ′ ≤ p as M ′ ≻ f and f = p.
P.7 similarly: Mi ≤ p for all Mi. Hence, p ′ ≤ p.
P.8 So p ′ ≡ p and fp ≡ f. Therefore, p is not a minimal polynomial.
c○: Michael Kohlhase 157
This theorem directly suggests a simple generate-and-test algorithm to construct minimal polynomials.
We will however improve on this using an idea by Quine and McCluskey. There are of
course better algorithms nowadays, but this one serves as a nice example of how to get from a
theoretical insight to a practical algorithm.
The Quine/McCluskey Algorithm (Idea)
Idea: use this theorem to search for minimal-cost polynomials
Determine all prime implicants (sub-algorithm QMC 1)
choose the minimal subset that covers f (sub-algorithm QMC 2)
Idea: To obtain prime implicants,
start with the DNF monomials (they are implicants by construction)
find submonomials that are still implicants of f.
Idea: Look at polynomials of the form p := mxi + m xi
c○: Michael Kohlhase 158
(note: p ≡ m)
Armed with the knowledge that minimal polynomials must consist entirely of prime implicants,
we can build a practical algorithm for computing minimal polynomials: In a first step we compute
the set of prime implicants of a given function, and later we see whether we actually need all of
them.
For the first step we use an important observation: for a given monomial m, the polynomials
m x + m x are equivalent, and in particular, we can obtain an equivalent polynomial by replace the
latter (the partners) by the former (the resolvent). That gives the main idea behind the first part
of the Quine-McCluskey algorithm. Given a Boolean function f, we start with a polynomial for f:
83