A particle-in-Burgers model: theory and numerics - Laboratoire de ...
A particle-in-Burgers model: theory and numerics - Laboratoire de ...
A particle-in-Burgers model: theory and numerics - Laboratoire de ...
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
Mo<strong>de</strong>l <strong>and</strong> motivation Auxiliary steps Results h = 0: coupl<strong>in</strong>g h = 0: <strong>de</strong>f<strong>in</strong>ition, uniqueness h = 0: <strong>numerics</strong>, existence The coupled problem<br />
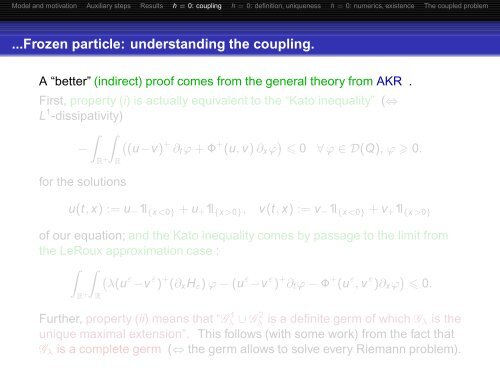
...Frozen <strong>particle</strong>: un<strong>de</strong>rst<strong>and</strong><strong>in</strong>g the coupl<strong>in</strong>g.<br />
A “better” (<strong>in</strong>direct) proof comes from the general <strong>theory</strong> from AKR .<br />
First, property (i) is actually equivalent to the “Kato <strong>in</strong>equality” (⇔<br />
L 1 -dissipativity)<br />
<br />
+ + <br />
− (u−v) ∂tϕ+Φ (u, v)∂xϕ 0 ∀ϕ ∈ D(Q), ϕ 0.<br />
R +<br />
R<br />
for the solutions<br />
u(t, x) := u−1l{x0}, v(t, x) := v−1l{x0}<br />
of our equation; <strong>and</strong> the Kato <strong>in</strong>equality comes by passage to the limit from<br />
the LeRoux approximation case :<br />
<br />
ε ε + ε ε + + ε ε<br />
λ(u −v ) (∂xHε)ϕ−(u −v ) ∂tϕ−Φ (u , v )∂xϕ 0.<br />
R +<br />
R<br />
Further, property (ii) means that “G 1 λ ∪ G 2 λ is a <strong>de</strong>f<strong>in</strong>ite germ of which Gλ is the<br />
unique maximal extension”. This follows (with some work) from the fact that<br />
Gλ is a complete germ (⇔ the germ allows to solve every Riemann problem).