Advanced Abstract Algebra - Maharshi Dayanand University, Rohtak

70
ADVANCED ABSTRACT ALGEBRA
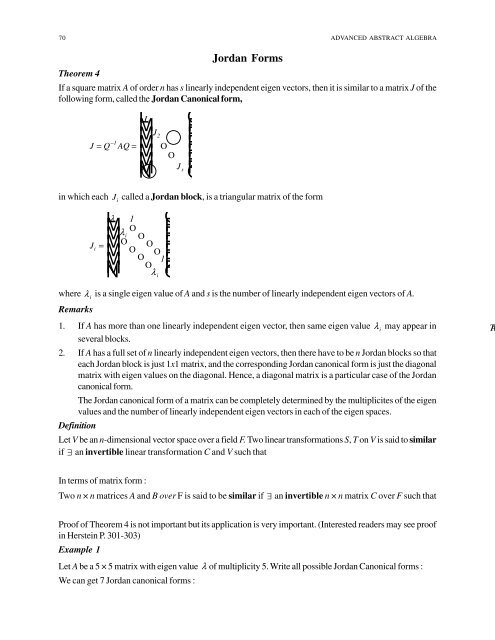
Theorem 4
NM
J s
QP
Jordan Forms
If a square matrix A of order n has s linearly independent eigen vectors, then it is similar to a matrix J of the
following form, called the Jordan Canonical form,
LJ
1
M
O
J
P
2
−1
J = Q AQ = Ο
Ο
in which each J i
called a Jordan block, is a triangular matrix of the form
J i
=
L
NM
λ i
Mλ
i
1
Ο
Ο
Ο Ο Ο Ο Ο 1 Ο
λ
i
O
QP
where λ i
is a single eigen value of A and s is the number of linearly independent eigen vectors of A.
Remarks
1. If A has more than one linearly independent eigen vector, then same eigen value λ i
may appear in
several blocks.
2. If A has a full set of n linearly independent eigen vectors, then there have to be n Jordan blocks so that
each Jordan block is just 1x1 matrix, and the corresponding Jordan canonical form is just the diagonal
matrix with eigen values on the diagonal. Hence, a diagonal matrix is a particular case of the Jordan
canonical form.
The Jordan canonical form of a matrix can be completely determined by the multiplicites of the eigen
values and the number of linearly independent eigen vectors in each of the eigen spaces.
Definition
Let V be an n-dimensional vector space over a field F. Two linear transformations S, T on V is said to similar
if ∃ an invertible linear transformation C and V such that
TA
In terms of matrix form :
Two n × n matrices A and B over F is said to be similar if ∃ an invertible n × n matrix C over F such that
Proof of Theorem 4 is not important but its application is very important. (Interested readers may see proof
in Herstein P. 301-303)
Example 1
Let A be a 5 × 5 matrix with eigen value λ of multiplicity 5. Write all possible Jordan Canonical forms :
We can get 7 Jordan canonical forms :