Symmetric Monoidal Categories for Operads - Index of

11.4 The Model Category of Σ∗-Objects 173
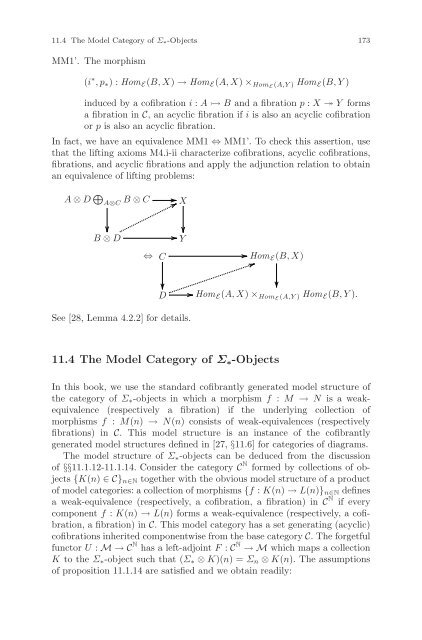
MM1’. The morphism
(i ∗ ,p∗) :HomE(B,X) → HomE(A, X) × HomE (A,Y ) HomE(B,Y )
induced by a cofibration i : A ↣ B and a fibration p : X ↠ Y forms
a fibration in C, an acyclic fibration if i is also an acyclic cofibration
or p is also an acyclic fibration.
In fact, we have an equivalence MM1 ⇔ MM1’. To check this assertion, use
that the lifting axioms M4.i-ii characterize cofibrations, acyclic cofibrations,
fibrations, and acyclic fibrations and apply the adjunction relation to obtain
an equivalence of lifting problems:
A ⊗ D �
A⊗C B ⊗ C X
B ⊗ D Y
⇔ C HomE(B,X)
D
See [28, Lemma 4.2.2] for details.
HomE(A, X) × HomE (A,Y ) HomE(B,Y ).
11.4 The Model Category of Σ∗-Objects
In this book, we use the standard cofibrantly generated model structure of
the category of Σ∗-objects in which a morphism f : M → N is a weakequivalence
(respectively a fibration) if the underlying collection of
morphisms f : M(n) → N(n) consists of weak-equivalences (respectively
fibrations) in C. This model structure is an instance of the cofibrantly
generated model structures defined in [27, §11.6] for categories of diagrams.
The model structure of Σ∗-objects can be deduced from the discussion
of §§11.1.12-11.1.14. Consider the category C N formed by collections of objects
{K(n) ∈C}n∈N together with the obvious model structure of a product
of model categories: a collection of morphisms {f : K(n) → L(n)}n∈N defines
a weak-equivalence (respectively, a cofibration, a fibration) in C N if every
component f : K(n) → L(n) forms a weak-equivalence (respectively, a cofibration,
a fibration) in C. This model category has a set generating (acyclic)
cofibrations inherited componentwise from the base category C. The forgetful
functor U : M→C N has a left-adjoint F : C N →Mwhich maps a collection
K to the Σ∗-object such that (Σ∗ ⊗ K)(n) =Σn ⊗ K(n). The assumptions
of proposition 11.1.14 are satisfied and we obtain readily: