Abel's theorem in problems and solutions - School of Mathematics
Abel's theorem in problems and solutions - School of Mathematics
Abel's theorem in problems and solutions - School of Mathematics
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
Solutions 207<br />
mov<strong>in</strong>g along a curve which does not pass through the po<strong>in</strong>ts<br />
<strong>and</strong> Moreover, every passage from one sheet to another, whilst<br />
cross<strong>in</strong>g a cut, co<strong>in</strong>cides with the passage <strong>in</strong>dicated on the scheme at that<br />
branch po<strong>in</strong>t from which this cut starts (cf., note 1 §2.10). Consequently<br />
the connection <strong>of</strong> the sheets at the branch po<strong>in</strong>ts must be made so as<br />
to obta<strong>in</strong> a connected scheme. S<strong>in</strong>ce po<strong>in</strong>ts different from <strong>and</strong><br />
are not branch po<strong>in</strong>ts (cf., 345), <strong>and</strong> at each one <strong>of</strong> the po<strong>in</strong>ts<br />
<strong>and</strong> only two sheets can jo<strong>in</strong> (cf., 346), <strong>in</strong> order to<br />
obta<strong>in</strong> a connected scheme we must put one arrow <strong>in</strong> correspondence with<br />
each one <strong>of</strong> the po<strong>in</strong>ts <strong>and</strong> i.e., all <strong>of</strong> these four po<strong>in</strong>ts<br />
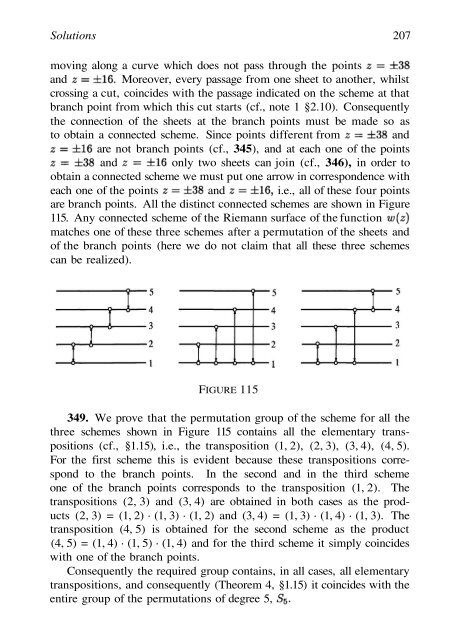
are branch po<strong>in</strong>ts. All the dist<strong>in</strong>ct connected schemes are shown <strong>in</strong> Figure<br />
115. Any connected scheme <strong>of</strong> the Riemann surface <strong>of</strong> the function<br />
matches one <strong>of</strong> these three schemes after a permutation <strong>of</strong> the sheets <strong>and</strong><br />
<strong>of</strong> the branch po<strong>in</strong>ts (here we do not claim that all these three schemes<br />
can be realized).<br />
FIGURE 115<br />
349. We prove that the permutation group <strong>of</strong> the scheme for all the<br />
three schemes shown <strong>in</strong> Figure 115 conta<strong>in</strong>s all the elementary transpositions<br />
(cf., §1.15), i.e., the transposition (1, 2), (2, 3), (3, 4), (4, 5).<br />
For the first scheme this is evident because these transpositions correspond<br />
to the branch po<strong>in</strong>ts. In the second <strong>and</strong> <strong>in</strong> the third scheme<br />
one <strong>of</strong> the branch po<strong>in</strong>ts corresponds to the transposition (1, 2). The<br />
transpositions (2, 3) <strong>and</strong> (3, 4) are obta<strong>in</strong>ed <strong>in</strong> both cases as the products<br />
(2, 3) = (1, 2) · (1, 3) · (1, 2) <strong>and</strong> (3, 4) = (1, 3) · (1, 4) · (1, 3). The<br />
transposition (4, 5) is obta<strong>in</strong>ed for the second scheme as the product<br />
(4, 5) = (1, 4) · (1, 5) · (1, 4) <strong>and</strong> for the third scheme it simply co<strong>in</strong>cides<br />
with one <strong>of</strong> the branch po<strong>in</strong>ts.<br />
Consequently the required group conta<strong>in</strong>s, <strong>in</strong> all cases, all elementary<br />
transpositions, <strong>and</strong> consequently (Theorem 4, §1.15) it co<strong>in</strong>cides with the<br />
entire group <strong>of</strong> the permutations <strong>of</strong> degree 5,