Abel's theorem in problems and solutions - School of Mathematics

78 Chapter 2
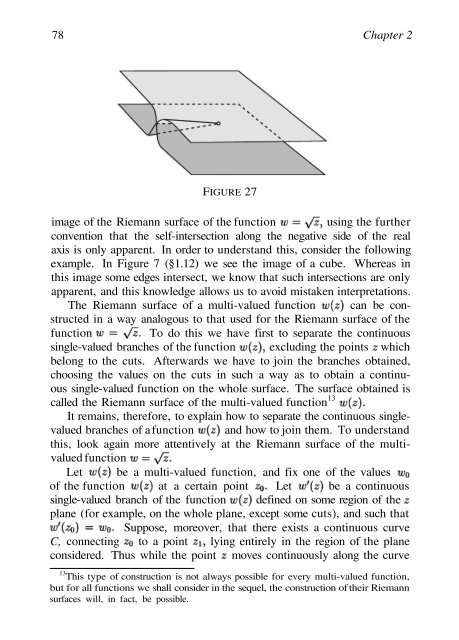
FIGURE 27
image of the Riemann surface of the function using the further
convention that the self-intersection along the negative side of the real
axis is only apparent. In order to understand this, consider the following
example. In Figure 7 (§1.12) we see the image of a cube. Whereas in
this image some edges intersect, we know that such intersections are only
apparent, and this knowledge allows us to avoid mistaken interpretations.
The Riemann surface of a multi-valued function can be constructed
in a way analogous to that used for the Riemann surface of the
function To do this we have first to separate the continuous
single-valued branches of the function excluding the points which
belong to the cuts. Afterwards we have to join the branches obtained,
choosing the values on the cuts in such a way as to obtain a continuous
single-valued function on the whole surface. The surface obtained is
called the Riemann surface of the multi-valued function 13
It remains, therefore, to explain how to separate the continuous singlevalued
branches of a function and how to join them. To understand
this, look again more attentively at the Riemann surface of the multivalued
function
Let be a multi-valued function, and fix one of the values
of the function at a certain point Let be a continuous
single-valued branch of the function defined on some region of the
plane (for example, on the whole plane, except some cuts), and such that
Suppose, moreover, that there exists a continuous curve
C, connecting to a point lying entirely in the region of the plane
considered. Thus while the point moves continuously along the curve
13 This type of construction is not always possible for every multi-valued function,
but for all functions we shall consider in the sequel, the construction of their Riemann
surfaces will, in fact, be possible.