Quantum Information Theory with Gaussian Systems

ρ2
p
α
ρ1
q
2.2 Gaussian states
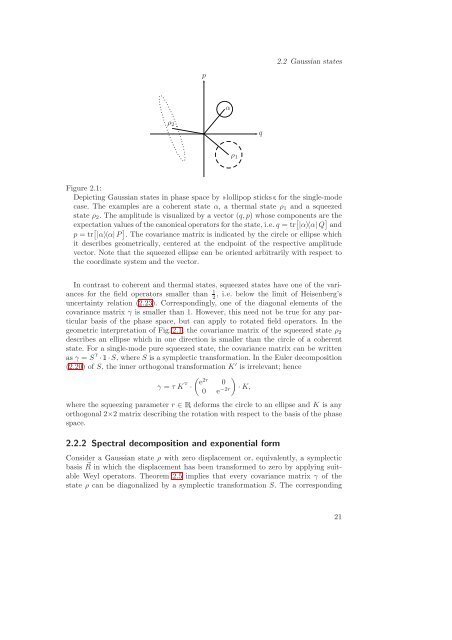
Figure 2.1:
Depicting Gaussian states in phase space bylollipop sticksfor the single-mode
case. The examples are a coherent state α, a thermal state ρ1 and a squeezed
state ρ2. The amplitude is visualized by a vector (q, p) whose components are the
expectation values of the canonical operators for the state, i.e. q = tr |α〉〈α| Q and
p = tr |α〉〈α| P . The covariance matrix is indicated by the circle or ellipse which
it describes geometrically, centered at the endpoint of the respective amplitude
vector. Note that the squeezed ellipse can be oriented arbitrarily with respect to
the coordinate system and the vector.
In contrast to coherent and thermal states, squeezed states have one of the variances
for the field operators smaller than 1
2 , i.e. below the limit of Heisenberg’s
uncertainty relation (2.23). Correspondingly, one of the diagonal elements of the
covariance matrix γ is smaller than 1. However, this need not be true for any particular
basis of the phase space, but can apply to rotated field operators. In the
geometric interpretation of Fig. 2.1, the covariance matrix of the squeezed state ρ2
describes an ellipse which in one direction is smaller than the circle of a coherent
state. For a single-mode pure squeezed state, the covariance matrix can be written
as γ = S T · ·S, where S is a symplectic transformation. In the Euler decomposition
(2.20) of S, the inner orthogonal transformation K ′ is irrelevant; hence
γ = τ K T
2r e 0
·
0 e−2r
· K,
where the squeezing parameter r ∈Êdeforms the circle to an ellipse and K is any
orthogonal 2×2 matrix describing the rotation with respect to the basis of the phase
space.
2.2.2 Spectral decomposition and exponential form
Consider a Gaussian state ρ with zero displacement or, equivalently, a symplectic
basis R in which the displacement has been transformed to zero by applying suitable
Weyl operators. Theorem 2.5 implies that every covariance matrix γ of the
state ρ can be diagonalized by a symplectic transformation S. The corresponding
21