Quantum Information Theory with Gaussian Systems
Quantum Information Theory with Gaussian Systems
Quantum Information Theory with Gaussian Systems
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
Im<br />
<br />
e iα(k)<br />
e−iα(k) <br />
Re<br />
4.2 Reversible <strong>Gaussian</strong> qca<br />
Im<br />
Re<br />
ea(k) e−a(k) <br />
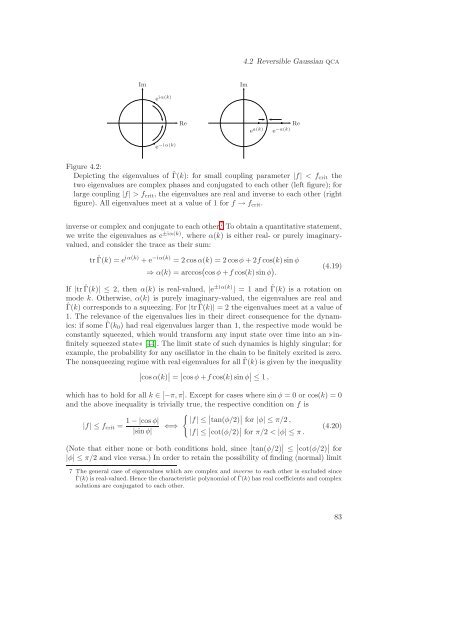
Figure 4.2:<br />
Depicting the eigenvalues of ˆ Γ(k): for small coupling parameter |f| < fcrit the<br />
two eigenvalues are complex phases and conjugated to each other (left figure); for<br />
large coupling |f| > fcrit, the eigenvalues are real and inverse to each other (right<br />
figure). All eigenvalues meet at a value of 1 for f → fcrit.<br />
inverse or complex and conjugate to each other. 7 To obtain a quantitative statement,<br />
we write the eigenvalues as e ±iα(k) , where α(k) is either real- or purely imaginaryvalued,<br />
and consider the trace as their sum:<br />
tr ˆ Γ(k) = e iα(k) + e −iα(k) = 2 cosα(k) = 2 cosφ + 2f cos(k)sin φ<br />
⇒ α(k) = arccos cosφ +f cos(k)sin φ .<br />
(4.19)<br />
If |tr ˆ Γ(k)| ≤ 2, then α(k) is real-valued, |e ±iα(k) | = 1 and ˆ Γ(k) is a rotation on<br />
mode k. Otherwise, α(k) is purely imaginary-valued, the eigenvalues are real and<br />
ˆΓ(k) corresponds to a squeezing. For |tr ˆ Γ(k)| = 2 the eigenvalues meet at a value of<br />
1. The relevance of the eigenvalues lies in their direct consequence for the dynamics:<br />
if some ˆ Γ(k0) had real eigenvalues larger than 1, the respective mode would be<br />
constantly squeezed, which would transform any input state over time into aninfinitely<br />
squeezed state[44]. The limit state of such dynamics is highly singular; for<br />
example, the probability for any oscillator in the chain to be finitely excited is zero.<br />
The nonsqueezing regime <strong>with</strong> real eigenvalues for all ˆ Γ(k) is given by the inequality<br />
<br />
cosα(k) = cosφ +f cos(k)sin φ ≤ 1 ,<br />
which has to hold for all k ∈ [−π, π]. Except for cases where sin φ = 0 or cos(k) = 0<br />
and the above inequality is trivially true, the respective condition on f is<br />
1 − |cosφ|<br />
|f| ≤ fcrit =<br />
|sinφ|<br />
⇐⇒<br />
<br />
|f| ≤ tan(φ/2) for |φ| ≤ π/2 ,<br />
|f| ≤ (4.20)<br />
cot(φ/2) for π/2 < |φ| ≤ π .<br />
(Note that either none or both conditions hold, since tan(φ/2) ≤ cot(φ/2) for<br />
|φ| ≤ π/2 and vice versa.) In order to retain the possibility of finding (normal) limit<br />
7 The general case of eigenvalues which are complex and inverse to each other is excluded since<br />
ˆΓ(k) is real-valued. Hence the characteristic polynomial of ˆ Γ(k) has real coefficients and complex<br />
solutions are conjugated to each other.<br />
83