Random Processes in Hyperbolic Spaces Hyperbolic Brownian ...
Random Processes in Hyperbolic Spaces Hyperbolic Brownian ...
Random Processes in Hyperbolic Spaces Hyperbolic Brownian ...
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
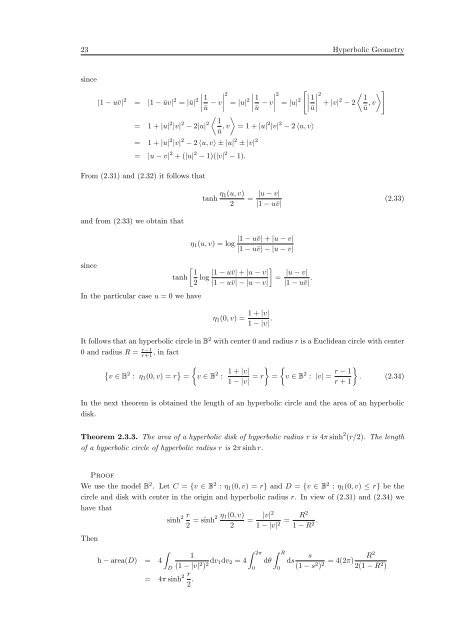
23 <strong>Hyperbolic</strong> Geometry<br />
s<strong>in</strong>ce<br />
|1 − u¯v| 2 = |1 − ūv| 2 = |ū| 2<br />
2<br />
<br />
<br />
1 <br />
− v<br />
ū = |u| 2<br />
2<br />
<br />
<br />
1 <br />
− v<br />
ū = |u| 2<br />
<br />
1 <br />
<br />
ū<br />
= 1 + |u| 2 |v| 2 − 2|u| 2<br />
<br />
1<br />
, v = 1 + |u|<br />
ū 2 |v| 2 − 2 〈u, v〉<br />
= 1 + |u| 2 |v| 2 − 2 〈u, v〉 ± |u| 2 ± |v| 2<br />
= |u − v| 2 + (|u| 2 − 1)(|v| 2 − 1).<br />
From (2.31) and (2.32) it follows that<br />
and from (2.33) we obta<strong>in</strong> that<br />
s<strong>in</strong>ce<br />
In the particular case u = 0 we have<br />
tanh η1(u, v)<br />
2<br />
η1(u, v) = log<br />
= |u − v|<br />
|1 − u¯v|<br />
|1 − u¯v| + |u − v|<br />
|1 − u¯v| − |u − v|<br />
<br />
1 |1 − u¯v| + |u − v|<br />
tanh log =<br />
2 |1 − u¯v| − |u − v|<br />
|u − v|<br />
|1 − u¯v| .<br />
η1(0, v) =<br />
1 + |v|<br />
1 − |v| .<br />
2<br />
+ |v| 2 <br />
1<br />
− 2 , v<br />
ū <br />
(2.33)<br />
It follows that an hyperbolic circle <strong>in</strong> B 2 with center 0 and radius r is a Euclidean circle with center<br />
0 and radius R = r−1<br />
r+1 , <strong>in</strong> fact<br />
v ∈ B 2 : η1(0, v) = r =<br />
<br />
v ∈ B 2 :<br />
<br />
1 + |v|<br />
= r = v ∈ B<br />
1 − |v| 2 <br />
r − 1<br />
: |v| = . (2.34)<br />
r + 1<br />
In the next theorem is obta<strong>in</strong>ed the length of an hyperbolic circle and the area of an hyperbolic<br />
disk.<br />
Theorem 2.3.3. The area of a hyperbolic disk of hyperbolic radius r is 4π s<strong>in</strong>h 2 (r/2). The length<br />
of a hyperbolic circle of hyperbolic radius r is 2π s<strong>in</strong>h r.<br />
Proof<br />
We use the model B2 . Let C = {v ∈ B2 : η1(0, v) = r} and D = {v ∈ B2 : η1(0, v) ≤ r} be the<br />
circle and disk with center <strong>in</strong> the orig<strong>in</strong> and hyperbolic radius r. In view of (2.31) and (2.34) we<br />
have that<br />
s<strong>in</strong>h 2 r<br />
2 = s<strong>in</strong>h2 η1(0, v)<br />
=<br />
2<br />
|v|2 R2<br />
= .<br />
1 − |v| 2 1 − R2 Then<br />
<br />
h − area(D) = 4<br />
D<br />
= 4π s<strong>in</strong>h 2 r<br />
2 .<br />
1<br />
(1 − |v| 2 ) 2 dv1dv2 = 4<br />
2π<br />
0<br />
dθ<br />
R<br />
0<br />
s<br />
ds<br />
(1 − s2 = 4(2π)<br />
) 2 2(1 − R2 )<br />
R 2