shot noise in mesoscopic conductors - Low Temperature Laboratory

More documents

Recommendations

Info

112 Ya.M. Blanter, M. Bu( ttiker / Physics Reports 336 (2000) 1}166 Fig. 31. Voltage dependence of the Fano factor (202) for the same set of parameters as Fig. 30 (solid line); Fano factor (78) for a charge neutral quantum well (dashed line). Poissonian. Jahan and Anwar [236], who also found super-Poissonian noise enhancement, included self-consistent e!ect at the level of the stationary transmission probabilities, but also did not take partition noise into account. As we already mentioned, an explanation of the super- Poissonian noise in terms of the potential #uctuations was given by Iannaccone et al. [240], and the analytical theory of this enhancement, identifying the relevant energy scales, was proposed in Refs. [241,226]. Experimentally, enhancement of noise in quantum wells, as the voltage approaches the range of negative di!erential resistance, was observed already in the early experiments by Li et al. [64] and by Brown [231]. The super-Poissonian shot noise in the negative di!erential resistance range was observed by Iannaccone et al. [240]. Kuznetsov et al. [232] have presented a detailed investigation of the noise oscillations from sub-Poissonian to super-Poissonian values of a resonant quantum well in the presence of a parallel magnetic "eld. The magnetic "eld leads to multiple voltage ranges of negative di!erential resistance and permits a clear demonstration of the e!ect. Their results are shown in Fig. 32. To conclude this section, we discuss the following issue. To obtain the super-Poissonian noise enhancement, we needed multi-stable behavior of the I}< curve; in turn, the multi-stability in quantum wells is induced by charging e!ects. It is easy to see, however, that the charging (or, generally, interaction) e!ects are not required to cause the multi-stability. Thus, if instead of a voltage-controlled experiment, we discuss a current-controlled experiment, the I}< characteristics for the uncharged quantum well (Fig. 30) are multi-stable for any external current. For the case of an arbitrary load line there typically exists a "nite range of external parameters where multi-stable behavior is developed. Furthermore, the quantum wells are not the only systems with multi-stability; as one well-known example we mention Esaki diodes, where the multi-stability is caused by the structure of the energy bands [243].
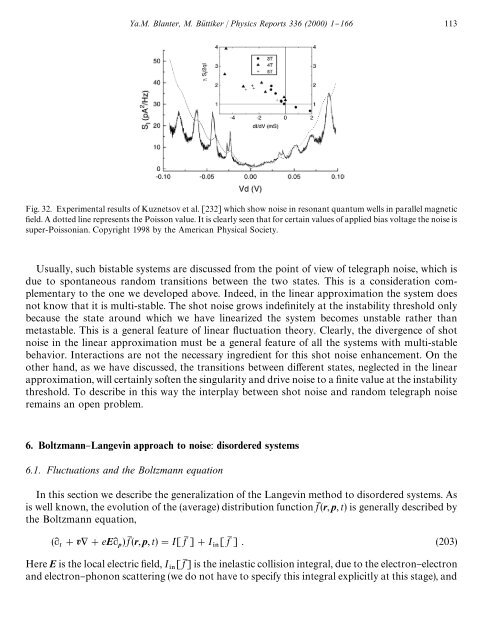
Fig. 32. Experimental results of Kuznetsov et al. [232] which show noise in resonant quantum wells in parallel magnetic "eld. A dotted line represents the Poisson value. It is clearly seen that for certain values of applied bias voltage the noise is super-Poissonian. Copyright 1998 by the American Physical Society. Usually, such bistable systems are discussed from the point of view of telegraph noise, which is due to spontaneous random transitions between the two states. This is a consideration complementary to the one we developed above. Indeed, in the linear approximation the system does not know that it is multi-stable. The shot noise grows inde"nitely at the instability threshold only because the state around which we have linearized the system becomes unstable rather than metastable. This is a general feature of linear #uctuation theory. Clearly, the divergence of shot noise in the linear approximation must be a general feature of all the systems with multi-stable behavior. Interactions are not the necessary ingredient for this shot noise enhancement. On the other hand, as we have discussed, the transitions between di!erent states, neglected in the linear approximation, will certainly soften the singularity and drive noise to a "nite value at the instability threshold. To describe in this way the interplay between shot noise and random telegraph noise remains an open problem. 6. Boltzmann}Langevin approach to noise: disordered systems 6.1. Fluctuations and the Boltzmann equation Ya.M. Blanter, M. Bu( ttiker / Physics Reports 336 (2000) 1}166 113 In this section we describe the generalization of the Langevin method to disordered systems. As is well known, the evolution of the (average) distribution function fM (r, p, t) is generally described by the Boltzmann equation, (R #*#eER p) fM (r, p, t)"I[ fM ]#I [ fM ] . (203) Here E is the local electric "eld, I [ fM ] is the inelastic collision integral, due to the electron}electron and electron}phonon scattering (we do not have to specify this integral explicitly at this stage), and
Page 1 and 2:
Ya.M. Blanter, M. BuK ttiker / Phys
Page 3 and 4:
8. Concluding remarks, future prosp
Page 5 and 6:
text; they are usually extensions o
Page 7 and 8:
such reviews are not readily availa
Page 9 and 10:
Ya.M. Blanter, M. Bu( ttiker / Phys
Page 11 and 12:
The above considerations are, of co
Page 13 and 14:
Page 15 and 16:
Page 17 and 18:
and the initial state of our two-pa
Page 19 and 20:
Page 21 and 22:
Page 23 and 24:
2.3.3. Multi-terminal case We consi
Page 25 and 26:
Note that in the absence of time-de
Page 27 and 28:
Page 29 and 30:
equilibrium and shot noise. For low
Page 31 and 32:
Page 33 and 34:
Page 35 and 36:
Page 37 and 38:
Page 39 and 40:
and S" e A e< dE ¹(E )[1!¹
Page 41 and 42:
arrier, situated in the region !w/2
Page 43 and 44:
Page 45 and 46:
Page 47 and 48:
we include weak localization e!ects
Page 49 and 50:
Page 51 and 52:
Page 53 and 54:
Page 55 and 56:
Page 57 and 58:
Page 59 and 60:
Edge channels in the quantum Hall e
Page 61 and 62: Fig. 19. (a) Geometry of a ring thr
Page 63 and 64: a manifestation of interference e!e
Page 65 and 66: Ya.M. Blanter, M. Bu( ttiker / Phys
Page 69 and 70: Physically, this corresponds to ele
Page 73 and 74: Our starting point is Eq. (51), whi
Page 77 and 78: introducing the transmission coe$ci
Page 79 and 80: investigated experimentally. A self
Page 85 and 86: and the non-equilibrium resistance
Page 87 and 88: the sample. These electrons are des
Page 93 and 94: with D ,¹ (2!¹ ). Performing t
Page 97 and 98: For completeness, we mention that i
Page 107 and 108: We note here, however, that there i
Page 111: The noise power (201) is strongly v
Page 115 and 116: where Ya.M. Blanter, M. Bu( ttiker
Page 117 and 118: where the quantity is expressed th
Page 119 and 120: 6.3. Interaction ewects Ya.M. Blant
Page 123 and 124: one "rst goes from the non-interact
Page 125 and 126: non-thermalized electrons, the high
Page 129 and 130: Fig. 34. Geometry of the chaotic ca
Page 133 and 134: which describes strictly ballistic
Page 141 and 142: approximately e/C. Between the peak
Page 149 and 150: Boltzmann}Langevin approach (see Se
Page 151 and 152: thermal to shot noise. It is, howev
Page 153 and 154: Note added in proof Ya.M. Blanter,
Page 155 and 156: and Lee et al. [340] obtain in this
Page 163 and 164:
Page 165 and 166:
show all

shot noise in mesoscopic conductors - Low Temperature Laboratory

Create successful ePaper yourself

Delete template?

Save as template?