You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
Plasma Phys. Control. Fusion 53 (2011) 093001<br />
Topical Review<br />
The first and second models are given in detail in [97] in which <strong>the</strong> transport coefficients in<br />
a magnetized plasma <strong>of</strong> Marshall [99] were employed. An asymmetry in heat flow is introduced<br />
by <strong>the</strong> inclusion <strong>of</strong> <strong>the</strong> enthalpy flow in <strong>the</strong> heat-flow equation and <strong>the</strong> <strong>the</strong>rmoelectric field in<br />
Ohm’s law, which are<br />
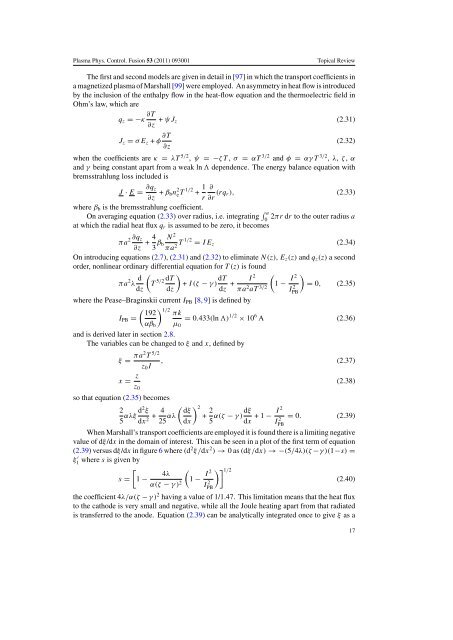
q z =−κ ∂T<br />
∂z + ψJ z (2.31)<br />
J z = σE z + φ ∂T<br />
(2.32)<br />
∂z<br />
when <strong>the</strong> coefficients are κ = λT 5/2 , ψ =−ζT, σ = αT 3/2 and φ = αγT 3/2 , λ, ζ , α<br />
and γ being constant apart from a weak ln dependence. The energy balance equation with<br />
bremsstrahlung loss included is<br />
J · E = ∂q z<br />
∂z + β bn 2 e T 1/2 + 1 ∂<br />
r ∂r (rq r), (2.33)<br />
where β b is <strong>the</strong> bremsstrahlung coefficient.<br />
On averaging equation (2.33) over radius, i.e. integrating ∫ a<br />
0<br />
2πr dr to <strong>the</strong> outer radius a<br />
at which <strong>the</strong> radial heat flux q r is assumed to be zero, it becomes<br />
N 2<br />
πa 2 ∂q z<br />
∂z + 4 3 β b<br />
πa T 1/2 = IE 2 z (2.34)<br />
On introducing equations (2.7), (2.31) and (2.32) to eliminate N(z), E z (z) and q z (z) a second<br />
order, nonlinear ordinary differential equation for T(z)is found<br />
πa 2 λ d ( )<br />
5/2<br />
dT<br />
T + I(ζ − γ) dT<br />
dz dz<br />
dz + I 2 (<br />
1 − I 2 )<br />
πa 2 aT 3/2 IPB<br />
2 = 0, (2.35)<br />
where <strong>the</strong> Pease–Braginskii current I PB [8, 9] is defined by<br />
( ) 192 1/2<br />
πk<br />
I PB =<br />
= 0.433(ln ) 1/2 × 10 6 A (2.36)<br />
αβ b µ 0<br />
and is derived later in section 2.8.<br />
The variables can be changed to ξ and x, defined by<br />
ξ = πa2 T 5/2<br />
, (2.37)<br />
z 0 I<br />
x = z (2.38)<br />
z 0<br />
so that equation (2.35) becomes<br />
2<br />
5 αλξ d2 ξ<br />
dx 2 + 4 25 αλ ( dξ<br />
dx<br />
) 2<br />
+ 2 α(ζ − γ)dξ<br />
5 dx +1− I 2<br />
IPB<br />
2 = 0. (2.39)<br />
When Marshall’s transport coefficients are employed it is found <strong>the</strong>re is a limiting negative<br />
value <strong>of</strong> dξ/dx in <strong>the</strong> domain <strong>of</strong> interest. This can be seen in a plot <strong>of</strong> <strong>the</strong> first term <strong>of</strong> equation<br />
(2.39) versus dξ/dx in figure 6 where (d 2 ξ/dx 2 ) → 0as(dξ/dx) →−(5/4λ)(ζ −γ)(1−s) =<br />
ξ ′ 1<br />
where s is given by<br />
[<br />
(<br />
4λ<br />
s = 1 −<br />
1 − I 2 )] 1/2<br />
α(ζ − γ) 2 IPB<br />
2 (2.40)<br />
<strong>the</strong> coefficient 4λ/α(ζ − γ) 2 having a value <strong>of</strong> 1/1.47. This limitation means that <strong>the</strong> heat flux<br />
to <strong>the</strong> cathode is very small and negative, while all <strong>the</strong> Joule heating apart from that radiated<br />
is transferred to <strong>the</strong> anode. Equation (2.39) can be analytically integrated once to give ξ as a<br />
17