Exercícios de Cálculo Integral e Diferencial I

ii)
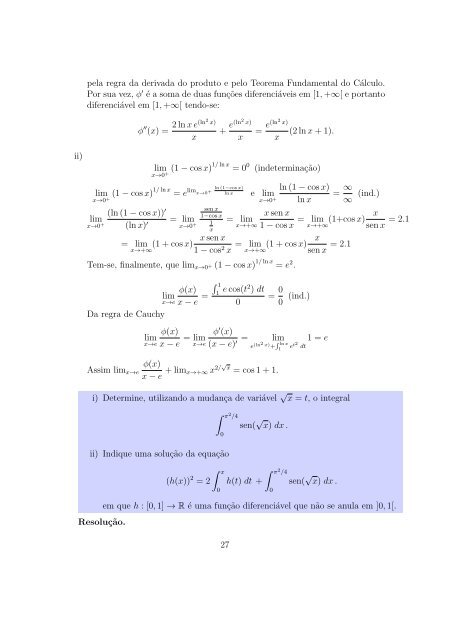
pela regra da derivada do produto e pelo Teorema Fundamental do Cálculo.
Por sua vez, φ ′ é a soma de duas funções diferenciáveis em [1, +∞[ e portanto
diferenciável em [1, +∞[ tendo-se:
φ ′′ (x) = 2 ln x e(ln2 x)
x
+ e(ln2 x)
x = e(ln2 x)
(2 ln x + 1).
x
lim
x→0 + (1 − cos x)1/ ln x = 0 0 (indeterminação)
lim
x→0 + (1 − cos x)1/ ln x = e lim ln (1−cos x)
x→0 + ln x e lim
x→0 +
ln (1 − cos x)
lim
x→0 +
(ln (1 − cos x)) ′
(ln x) ′
= lim
x→0 +
sen x
1−cos x
1
x
x sen x
= lim (1 + cos x)
x→+∞ 1 − cos2 x
x sen x
= lim
x→+∞ 1 − cos x
ln x
x
= lim (1 + cos x)
x→+∞ sen x
Tem-se, finalmente, que limx→0 + (1 − cos x)1/ ln x = e 2 .
φ(x)
lim
x→e x − e =
Da regra de Cauchy
Assim limx→e
φ(x)
lim
x→e x − e
= lim
x→e
1
1 e cos(t2 ) dt
0
φ ′ (x)
(x − e)
′ = lim
= 0
0 (ind.)
e (ln2
x) ln x
+ 1
φ(x)
x − e + limx→+∞ x 2/√ x = cos 1 + 1.
= ∞
∞ (ind.)
x
= lim (1+cos x)
x→+∞ sen x
et2 1 = e
dt
= 2.1
i) Determine, utilizando a mudança de variável √ x = t, o integral
π 2 /4
ii) Indique uma solução da equação
Resolução.
(h(x)) 2 = 2
0
x
0
sen( √ x) dx .
h(t) dt +
π 2 /4
0
sen( √ x) dx .
em que h : [0, 1] → R é uma função diferenciável que não se anula em ]0, 1[.
27
= 2.1