Contents - Student subdomain for University of Bath

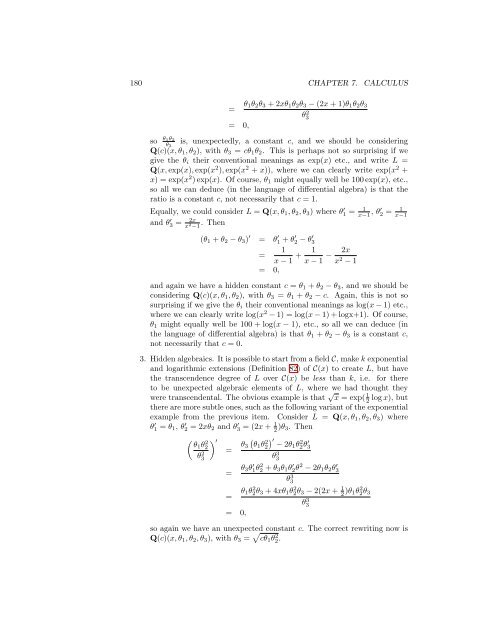
180 CHAPTER 7. CALCULUS
so θ1θ2
θ 3
= θ 1θ 2 θ 3 + 2xθ 1 θ 2 θ 3 − (2x + 1)θ 1 θ 2 θ 3
θ 2 3
= 0,
is, unexpectedly, a constant c, and we should be considering
Q(c)(x, θ 1 , θ 2 ), with θ 3 = cθ 1 θ 2 . This is perhaps not so surprising if we
give the θ i their conventional meanings as exp(x) etc., and write L =
Q(x, exp(x), exp(x 2 ), exp(x 2 + x)), where we can clearly write exp(x 2 +
x) = exp(x 2 ) exp(x). Of course, θ 1 might equally well be 100 exp(x), etc.,
so all we can deduce (in the language of differential algebra) is that the
ratio is a constant c, not necessarily that c = 1.
Equally, we could consider L = Q(x, θ 1 , θ 2 , θ 3 ) where θ 1 ′ = 1
x−1 , θ′ 2 = 1
and θ 3 ′ =
2x
x 2 −1 . Then
x−1
(θ 1 + θ 2 − θ 3 ) ′ = θ 1 ′ + θ 2 ′ − θ 3
′
=
1
x − 1 + 1
x − 1 − 2x
x 2 − 1
= 0,
and again we have a hidden constant c = θ 1 + θ 2 − θ 3 , and we should be
considering Q(c)(x, θ 1 , θ 2 ), with θ 3 = θ 1 + θ 2 − c. Again, this is not so
surprising if we give the θ i their conventional meanings as log(x − 1) etc.,
where we can clearly write log(x 2 − 1) = log(x − 1) + logx+1). Of course,
θ 1 might equally well be 100 + log(x − 1), etc., so all we can deduce (in
the language of differential algebra) is that θ 1 + θ 2 − θ 3 is a constant c,
not necessarily that c = 0.
3. Hidden algebraics. It is possible to start from a field C, make k exponential
and logarithmic extensions (Definition 82) of C(x) to create L, but have
the transcendence degree of L over C(x) be less than k, i.e. for there
to be unexpected algebraic elements of L, where we had thought they
were transcendental. The obvious example is that √ x = exp( 1 2
log x), but
there are more subtle ones, such as the following variant of the exponential
example from the previous item. Consider L = Q(x, θ 1 , θ 2 , θ 3 ) where
θ 1 ′ = θ 1 , θ 2 ′ = 2xθ 2 and θ 3 ′ = (2x + 1 2 )θ 3. Then
(
θ1 θ2
2 ) ′
= θ ( )
3 θ1 θ2
2 ′
− 2θ1 θ2θ 2 3
′
θ 2 3
θ 3 3
= θ 3θ ′ 1θ 2 2 + θ 3 θ 1 θ ′ 2θ 2 − 2θ 1 θ 2 θ ′ 3
θ 3 3
= θ 1θ 2 2θ 3 + 4xθ 1 θ 2 2θ 3 − 2(2x + 1 2 )θ 1θ 2 2θ 3
θ 3 3
= 0,
so again we have an unexpected constant c. The correct rewriting now is
Q(c)(x, θ 1 , θ 2 , θ 3 ), with θ 3 = √ cθ 1 θ 2 2 .