DBI Analysis of Open String Bound States on Non-compact D-branes

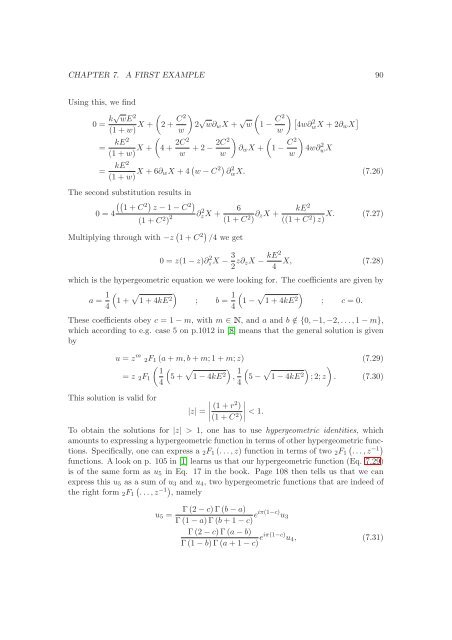
CHAPTER 7. A FIRST EXAMPLE 90Using this, we find0 = k√ wE 2 ((1 + w) X + 2 + C2w)2 √ w∂ w X + √ w(= kE2(1 + w) X + 4 + 2C2w + 2 − 2C2w)∂ w X +) (1 − C2 [4w∂2w X + 2∂ w X ]w(1 − C2w)4w∂ 2 wX= kE2(1 + w) X + 6∂ wX + 4 ( w − C 2) ∂ 2 wX. (7.26)The second substitution results in0 = 4((1 + C2 ) z − 1 − C 2)(1 + C 2 ) 2 ∂ 2 zX +Multiplying through with −z ( 1 + C 2) /4 we get6(1 + C 2 ) ∂ zX +kE 2((1 + C 2 X. (7.27))z)0 = z(1 − z)∂zX 2 − 3 2 z∂ zX − kE2 X, (7.28)4which is the hypergeometric equation we were looking for. The coefficients are given bya = 1 (1 + √ )1 + 4kE42 ; b = 1 (1 − √ )1 + 4kE42 ; c = 0.These coefficients obey c = 1 − m, with m ∈Æ, and a and b /∈ {0, −1, −2, . . .,1 − m},which according to e.g. case 5 on p.1012 in [8] means that the general solution is givenbyThis solution is valid foru = z m 2F 1 (a + m, b + m; 1 + m; z) (7.29)( 1= z 2 F 1(5 + √ )1 − 4kE42 , 1 (5 − √ ) )1 − 4kE42 ; 2;z . (7.30)|z| =(1 + r 2 )∣(1 + C 2 ) ∣ < 1.To obtain the solutions for |z| > 1, one has to use hypergeometric identities, whichamounts to expressing a hypergeometric function in terms <strong>of</strong> other hypergeometric functions.Specifically, one can express a 2 F 1 (. . .,z) function in terms <strong>of</strong> two 2 F 1(. . .,z−1 )functions. A look on p. 105 in [1] learns us that our hypergeometric function (Eq. 7.29)is <strong>of</strong> the same form as u 5 in Eq. 17 in the book. Page 108 then tells us that we canexpress this u 5 as a sum <strong>of</strong> u 3 and u 4 , two hypergeometric functions that are indeed <strong>of</strong>the right form 2 F 1(. . .,z−1 ) , namelyu 5 =Γ (2 − c)Γ(b − a)Γ (1 − a)Γ(b + 1 − c) eiπ(1−c) u 3Γ (2 − c)Γ(a − b)Γ (1 − b) Γ (a + 1 − c) eiπ(1−c) u 4 , (7.31)