MYSTERIES OF THE EQUILATERAL TRIANGLE - HIKARI Ltd
MYSTERIES OF THE EQUILATERAL TRIANGLE - HIKARI Ltd
MYSTERIES OF THE EQUILATERAL TRIANGLE - HIKARI Ltd
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
Mathematical Recreations 111<br />
Recreation 13 (Erdös’ Sphere Coloring Problem [131]). Paul Erdös<br />
proposed the following unsolved problem in graph theory. What is the minimum<br />
number of colors required to paint all of the points on the surface of a unit<br />
sphere so that, no matter how we inscribe an equilateral triangle of side √ 3<br />
(the largest such triangle that can be so inscribed), the triangle will have each<br />
corner on a different color (Figure 4.13(a)) [131]?<br />
E. G. Straus has shown that five colors suffice. In his five-coloring (shown in<br />
Figure 4.13(b)), the north polar region is open with boundary circle of diameter<br />
√ 3. The rest of the sphere is divided into four identical regions, each closed<br />
along its northern and eastern borders, as indicated by the heavy black line on<br />
the dark shaded region. One color is given to the cap and to the south pole.<br />
The remaining four colors are assigned to four quadrant regions. G. J. Simmons<br />
has shown that three colors are not sufficient so that at least four colors are<br />
necessary. It is unknown whether four or five colors are both necessary and<br />
sufficient. The analogous problem for the plane, i.e. the minimum number of<br />
colors which ensures that every equilateral triangle of unit side will have its<br />
corners on different colors, is also open. Indeed, it is equivalent to asking for a<br />
minimal coloring of the plane so that every unit line segment has its endpoints<br />
on different colors, a problem which was discussed at the end of the previous<br />
Recreation. This problem may be recast in terms of the chromatic number of<br />
planar graphs [131].<br />
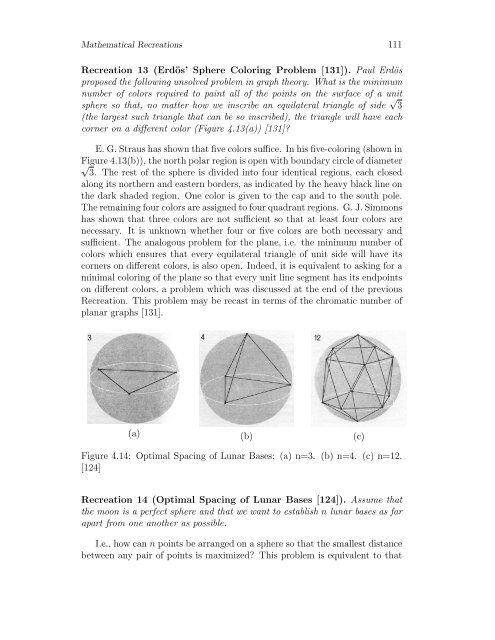
(a) (b) (c)<br />
Figure 4.14: Optimal Spacing of Lunar Bases: (a) n=3. (b) n=4. (c) n=12.<br />
[124]<br />
Recreation 14 (Optimal Spacing of Lunar Bases [124]). Assume that<br />
the moon is a perfect sphere and that we want to establish n lunar bases as far<br />
apart from one another as possible.<br />
I.e., how can n points be arranged on a sphere so that the smallest distance<br />
between any pair of points is maximized? This problem is equivalent to that