MYSTERIES OF THE EQUILATERAL TRIANGLE - HIKARI Ltd

44 Mathematical Properties
be the side of the largest equilateral triangle ∆ z in that fits in T with one side
along side z ∈ {a, b, c} of T, we have that sin = max{s a in, s b in, s c in}. Likewise,
defining sout to be the side of ∆out and s z out to be the side of the smallest
equilateral triangle ∆ z in containing T with one side along side z ∈ {a, b, c} of
T, we have that sout = min{s a out, s b out, s c out}. They establish the surprising fact
that ∆in and ∆out always rest on the same side of T. Curiously, the area of
T is the geometric mean of the areas of ∆ z in and ∆ z out for each side z of T
and thus of the areas of ∆in and ∆out. Figures 2.19(a-c) display the three
possible configurations where z is the longest, shortest and median side of T,
respectively.
They also show that ∆in and ∆out always lie on either the longest or the
shortest side of T. If the median angle of T is at most 60 ◦ then they rest
on the longest side. Otherwise, there is a complicated condition involving the
median angle and its adjacent sides which determines whether they rest on the
longest or the shortest side of T. Along the way, they work out the ordering
relations among s a in, s b in, s c in and s a out, s b out, s c out which then permits the explicit
calculation of sin and sout. It follows that if an equilateral triangle ∆ of side s
and a triangle T are given, then ∆ fits within T precisely when s ≤ sin and T
fits within ∆ precisely when s ≥ sout. Finally, they establish that the isosceles
triangle with apex angle 20 ◦ is the unique nonequilateral triangle for which the
three inner/outer maximal equilateral triangles are congruent (Figure 2.19(d)).
(a)
(b) (c) (d) (e)
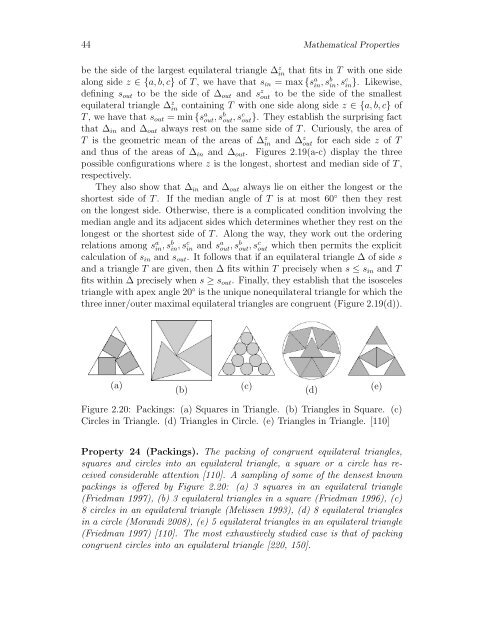
Figure 2.20: Packings: (a) Squares in Triangle. (b) Triangles in Square. (c)
Circles in Triangle. (d) Triangles in Circle. (e) Triangles in Triangle. [110]
Property 24 (Packings). The packing of congruent equilateral triangles,
squares and circles into an equilateral triangle, a square or a circle has received
considerable attention [110]. A sampling of some of the densest known
packings is offered by Figure 2.20: (a) 3 squares in an equilateral triangle
(Friedman 1997), (b) 3 equilateral triangles in a square (Friedman 1996), (c)
8 circles in an equilateral triangle (Melissen 1993), (d) 8 equilateral triangles
in a circle (Morandi 2008), (e) 5 equilateral triangles in an equilateral triangle
(Friedman 1997) [110]. The most exhaustively studied case is that of packing
congruent circles into an equilateral triangle [220, 150].