MYSTERIES OF THE EQUILATERAL TRIANGLE - HIKARI Ltd
MYSTERIES OF THE EQUILATERAL TRIANGLE - HIKARI Ltd
MYSTERIES OF THE EQUILATERAL TRIANGLE - HIKARI Ltd
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
70 Mathematical Properties<br />
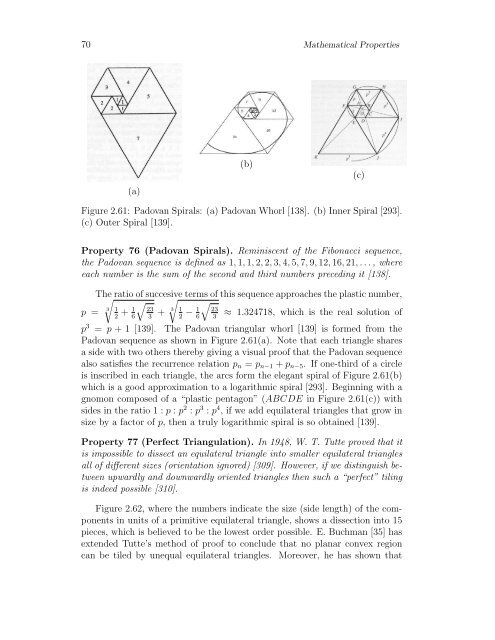
(a)<br />
(b)<br />
Figure 2.61: Padovan Spirals: (a) Padovan Whorl [138]. (b) Inner Spiral [293].<br />
(c) Outer Spiral [139].<br />
Property 76 (Padovan Spirals). Reminiscent of the Fibonacci sequence,<br />
the Padovan sequence is defined as 1, 1, 1, 2, 2, 3, 4, 5, 7, 9, 12, 16, 21, . . ., where<br />
each number is the sum of the second and third numbers preceding it [138].<br />
The �ratio<br />
of succesive � terms of this sequence approaches the plastic number,<br />
p = 3<br />
1<br />
2<br />
+ 1<br />
6<br />
� 23<br />
3<br />
+ 3<br />
1<br />
2<br />
− 1<br />
6<br />
� 23<br />
3<br />
(c)<br />
≈ 1.324718, which is the real solution of<br />
p 3 = p + 1 [139]. The Padovan triangular whorl [139] is formed from the<br />
Padovan sequence as shown in Figure 2.61(a). Note that each triangle shares<br />
a side with two others thereby giving a visual proof that the Padovan sequence<br />
also satisfies the recurrence relation pn = pn−1 + pn−5. If one-third of a circle<br />
is inscribed in each triangle, the arcs form the elegant spiral of Figure 2.61(b)<br />
which is a good approximation to a logarithmic spiral [293]. Beginning with a<br />
gnomon composed of a “plastic pentagon” (ABCDE in Figure 2.61(c)) with<br />
sides in the ratio 1 : p : p 2 : p 3 : p 4 , if we add equilateral triangles that grow in<br />
size by a factor of p, then a truly logarithmic spiral is so obtained [139].<br />
Property 77 (Perfect Triangulation). In 1948, W. T. Tutte proved that it<br />
is impossible to dissect an equilateral triangle into smaller equilateral triangles<br />
all of different sizes (orientation ignored) [309]. However, if we distinguish between<br />
upwardly and downwardly oriented triangles then such a “perfect” tiling<br />
is indeed possible [310].<br />
Figure 2.62, where the numbers indicate the size (side length) of the components<br />
in units of a primitive equilateral triangle, shows a dissection into 15<br />
pieces, which is believed to be the lowest order possible. E. Buchman [35] has<br />
extended Tutte’s method of proof to conclude that no planar convex region<br />
can be tiled by unequal equilateral triangles. Moreover, he has shown that