MYSTERIES OF THE EQUILATERAL TRIANGLE - HIKARI Ltd
MYSTERIES OF THE EQUILATERAL TRIANGLE - HIKARI Ltd
MYSTERIES OF THE EQUILATERAL TRIANGLE - HIKARI Ltd
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
56 Mathematical Properties<br />
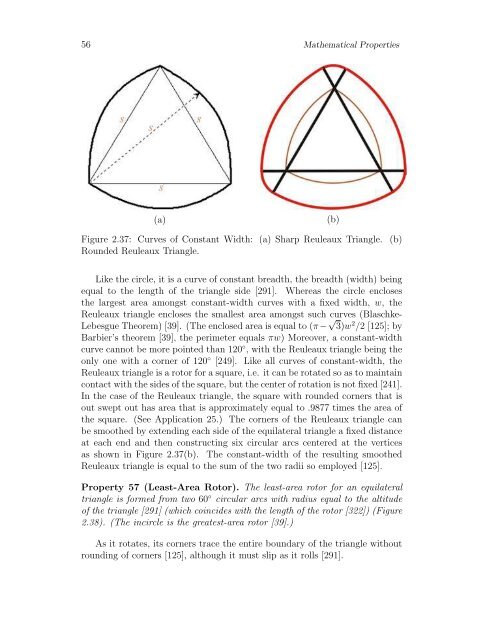
(a) (b)<br />
Figure 2.37: Curves of Constant Width: (a) Sharp Reuleaux Triangle. (b)<br />
Rounded Reuleaux Triangle.<br />
Like the circle, it is a curve of constant breadth, the breadth (width) being<br />
equal to the length of the triangle side [291]. Whereas the circle encloses<br />
the largest area amongst constant-width curves with a fixed width, w, the<br />
Reuleaux triangle encloses the smallest area amongst such curves (Blaschke-<br />
Lebesgue Theorem) [39]. (The enclosed area is equal to (π− √ 3)w 2 /2 [125]; by<br />
Barbier’s theorem [39], the perimeter equals πw) Moreover, a constant-width<br />
curve cannot be more pointed than 120 ◦ , with the Reuleaux triangle being the<br />
only one with a corner of 120 ◦ [249]. Like all curves of constant-width, the<br />
Reuleaux triangle is a rotor for a square, i.e. it can be rotated so as to maintain<br />
contact with the sides of the square, but the center of rotation is not fixed [241].<br />
In the case of the Reuleaux triangle, the square with rounded corners that is<br />
out swept out has area that is approximately equal to .9877 times the area of<br />
the square. (See Application 25.) The corners of the Reuleaux triangle can<br />
be smoothed by extending each side of the equilateral triangle a fixed distance<br />
at each end and then constructing six circular arcs centered at the vertices<br />
as shown in Figure 2.37(b). The constant-width of the resulting smoothed<br />
Reuleaux triangle is equal to the sum of the two radii so employed [125].<br />
Property 57 (Least-Area Rotor). The least-area rotor for an equilateral<br />
triangle is formed from two 60 ◦ circular arcs with radius equal to the altitude<br />
of the triangle [291] (which coincides with the length of the rotor [322]) (Figure<br />
2.38). (The incircle is the greatest-area rotor [39].)<br />
As it rotates, its corners trace the entire boundary of the triangle without<br />
rounding of corners [125], although it must slip as it rolls [291].