MYSTERIES OF THE EQUILATERAL TRIANGLE - HIKARI Ltd

Mathematical Properties 45
(a)
(b)
(c)
(d) (e)
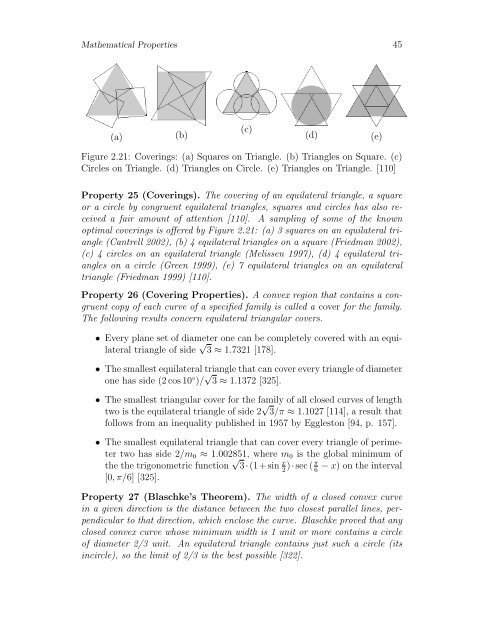
Figure 2.21: Coverings: (a) Squares on Triangle. (b) Triangles on Square. (c)
Circles on Triangle. (d) Triangles on Circle. (e) Triangles on Triangle. [110]
Property 25 (Coverings). The covering of an equilateral triangle, a square
or a circle by congruent equilateral triangles, squares and circles has also received
a fair amount of attention [110]. A sampling of some of the known
optimal coverings is offered by Figure 2.21: (a) 3 squares on an equilateral triangle
(Cantrell 2002), (b) 4 equilateral triangles on a square (Friedman 2002),
(c) 4 circles on an equilateral triangle (Melissen 1997), (d) 4 equilateral triangles
on a circle (Green 1999), (e) 7 equilateral triangles on an equilateral
triangle (Friedman 1999) [110].
Property 26 (Covering Properties). A convex region that contains a congruent
copy of each curve of a specified family is called a cover for the family.
The following results concern equilateral triangular covers.
• Every plane set of diameter one can be completely covered with an equilateral
triangle of side √ 3 ≈ 1.7321 [178].
• The smallest equilateral triangle that can cover every triangle of diameter
one has side (2 cos 10 ◦ )/ √ 3 ≈ 1.1372 [325].
• The smallest triangular cover for the family of all closed curves of length
two is the equilateral triangle of side 2 √ 3/π ≈ 1.1027 [114], a result that
follows from an inequality published in 1957 by Eggleston [94, p. 157].
• The smallest equilateral triangle that can cover every triangle of perimeter
two has side 2/m0 ≈ 1.002851, where m0 is the global minimum of
the the trigonometric function √ 3·(1+sin x)·sec
(π − x) on the interval
2 6
[0, π/6] [325].
Property 27 (Blaschke’s Theorem). The width of a closed convex curve
in a given direction is the distance between the two closest parallel lines, perpendicular
to that direction, which enclose the curve. Blaschke proved that any
closed convex curve whose minimum width is 1 unit or more contains a circle
of diameter 2/3 unit. An equilateral triangle contains just such a circle (its
incircle), so the limit of 2/3 is the best possible [322].