MYSTERIES OF THE EQUILATERAL TRIANGLE - HIKARI Ltd

Mathematical Properties 65
Property 69 (Tetrahedral Geodesics). A geodesic is a generalization of
a straight line which, in the presence of a metric, is defined to be the (locally)
shortest path between two points as measured along the surface [305].
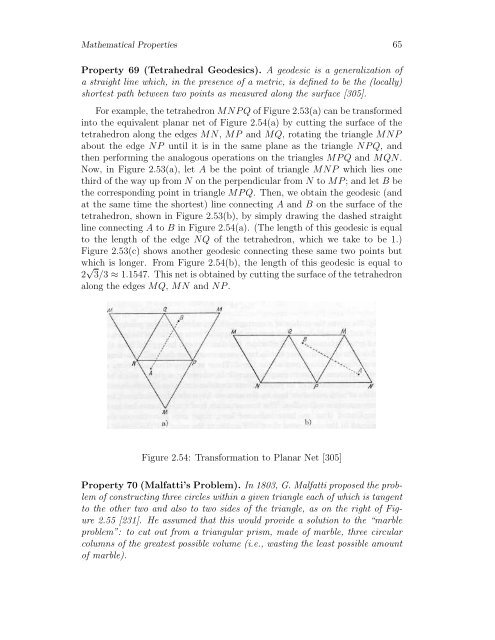
For example, the tetrahedron MNPQ of Figure 2.53(a) can be transformed
into the equivalent planar net of Figure 2.54(a) by cutting the surface of the
tetrahedron along the edges MN, MP and MQ, rotating the triangle MNP
about the edge NP until it is in the same plane as the triangle NPQ, and
then performing the analogous operations on the triangles MPQ and MQN.
Now, in Figure 2.53(a), let A be the point of triangle MNP which lies one
third of the way up from N on the perpendicular from N to MP; and let B be
the corresponding point in triangle MPQ. Then, we obtain the geodesic (and
at the same time the shortest) line connecting A and B on the surface of the
tetrahedron, shown in Figure 2.53(b), by simply drawing the dashed straight
line connecting A to B in Figure 2.54(a). (The length of this geodesic is equal
to the length of the edge NQ of the tetrahedron, which we take to be 1.)
Figure 2.53(c) shows another geodesic connecting these same two points but
which is longer. From Figure 2.54(b), the length of this geodesic is equal to
2 √ 3/3 ≈ 1.1547. This net is obtained by cutting the surface of the tetrahedron
along the edges MQ, MN and NP.
Figure 2.54: Transformation to Planar Net [305]
Property 70 (Malfatti’s Problem). In 1803, G. Malfatti proposed the problem
of constructing three circles within a given triangle each of which is tangent
to the other two and also to two sides of the triangle, as on the right of Figure
2.55 [231]. He assumed that this would provide a solution to the “marble
problem”: to cut out from a triangular prism, made of marble, three circular
columns of the greatest possible volume (i.e., wasting the least possible amount
of marble).