5.1 INTEGRALES DOBLES 5.2 INTEGRALES TRIPLES
5.1 INTEGRALES DOBLES 5.2 INTEGRALES TRIPLES
5.1 INTEGRALES DOBLES 5.2 INTEGRALES TRIPLES
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
MOISES VILLENA Integración Múltiple<br />
∫∫<br />
2<br />
x +<br />
2<br />
y +<br />
x<br />
2<br />
z<br />
h a<br />
∫ ∫<br />
( 2<br />
2<br />
) + ( 2<br />
2 2 ) + 0<br />
R<br />
0 0<br />
h a<br />
F F F x y<br />
S = dydz = 4<br />
dydz<br />
F 2x<br />
= 4<br />
∫∫<br />
0 0<br />
2<br />
2a<br />
a − y<br />
2 2<br />
⎛ y ⎞ h<br />
= 4a<br />
⎜arcsen ⎟ ( z)<br />
0<br />
⎝ a ⎠0<br />
= 4a( arcsen1−arcsen0) h<br />
⎛π⎞ = 4a⎜<br />
⎟h<br />
⎝ 2 ⎠<br />
= 2π<br />
ah<br />
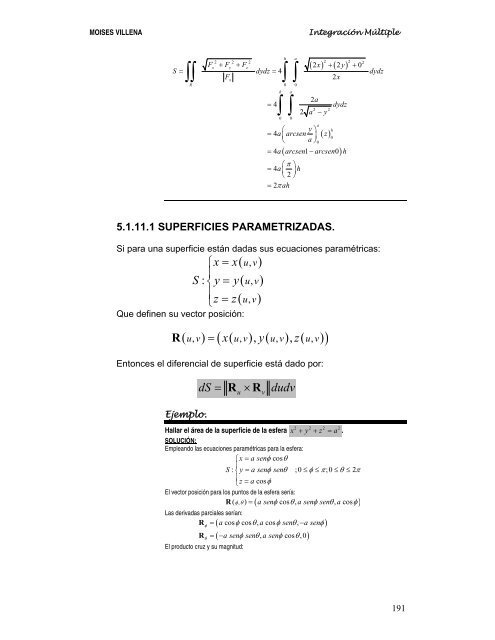
<strong>5.1</strong>.11.1 SUPERFICIES PARAMETRIZADAS.<br />
a<br />
dydz<br />
Si para una superficie están dadas sus ecuaciones paramétricas:<br />
uv ,<br />
⎧x=<br />
x(<br />
)<br />
⎪<br />
S: ⎨y<br />
= y(<br />
uv , )<br />
⎪ z = z(<br />
uv , )<br />
⎩<br />
Que definen su vector posición:<br />
( )<br />
R ( uv , ) = x( uv , ) , y( uv , ) , z(<br />
uv , )<br />
Entonces el diferencial de superficie está dado por:<br />
Ejemplo.<br />
dS = R × R dudv<br />
u v<br />
2 2 2 2<br />
Hallar el área de la superficie de la esfera x + y + z = a .<br />
SOLUCIÓN:<br />
Empleando las ecuaciones paramétricas para la esfera:<br />
⎧x<br />
= a senφ<br />
cosθ<br />
⎪<br />
S : ⎨y<br />
= a senφsenθ ;0 ≤φ ≤π;0≤θ ≤ 2π<br />
⎪<br />
⎩z<br />
= a cosφ<br />
El vector posición para los puntos de la esfera sería:<br />
R ( φθ , ) = ( asenφcos θ, asenφ senθ, acosφ)<br />
Las derivadas parciales serían:<br />
R φ = ( a cosφ cos θ, a cos φ senθ, −asenφ)<br />
( a sen sen , a sen cos ,0)<br />
R θ = − φ θ φ θ<br />
El producto cruz y su magnitud:<br />
191