Projects - Cengage Learning

C-56 Appendix C
y
y
y
1
1
1
-3
-2 -1
1 2 3
x x x
-3 -2 -1 1 2 3 -3 -2 -1 1 2 3
-1
4
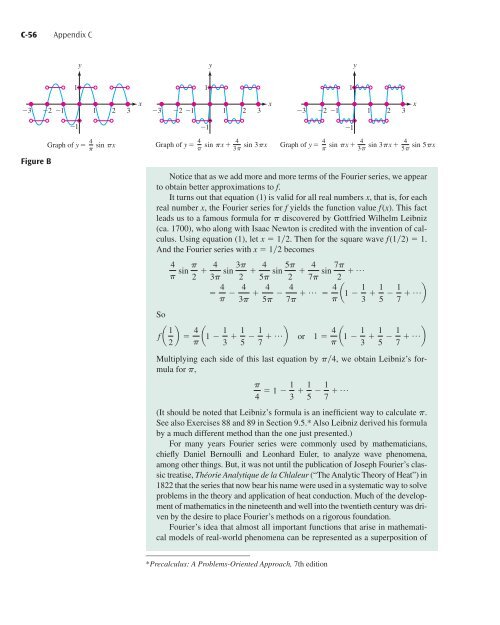
Graph of y= sin πx
π
Figure B
4
4
4
4
4
Graph of y= sin πx+ sin 3πx Graph of y= sin πx+ sin 3πx+ sin 5πx
π 3π
π 3π
5π
Notice that as we add more and more terms of the Fourier series, we appear
to obtain better approximations to f.
It turns out that equation (1) is valid for all real numbers x, that is, for each
real number x, the Fourier series for f yields the function value f(x). This fact
leads us to a famous formula for p discovered by Gottfried Wilhelm Leibniz
(ca. 1700), who along with Isaac Newton is credited with the invention of calculus.
Using equation (1), let x 12. Then for the square wave f(12) 1.
And the Fourier series with x 12 becomes
So
-1
4
p sin p 2 4
3p sin 3p 2 4
5p sin 5p 2 4
7p sin 7p 2 p
4 p 4
3p 4
5p 4
7p p 4 p a1 1 3 1 5 1 7 p b
-1
f a 1 2 b 4 p a1 1 3 1 5 1 7 p b
or
1 4 p a1 1 3 1 5 1 7 p b
Multiplying each side of this last equation by p4, we obtain Leibniz’s formula
for p,
p
4 1 1 3 1 5 1 7 p
(It should be noted that Leibniz’s formula is an inefficient way to calculate p.
See also Exercises 88 and 89 in Section 9.5.* Also Leibniz derived his formula
by a much different method than the one just presented.)
For many years Fourier series were commonly used by mathematicians,
chiefly Daniel Bernoulli and Leonhard Euler, to analyze wave phenomena,
among other things. But, it was not until the publication of Joseph Fourier’s classic
treatise, Théorie Analytique de la Chlaleur (“The Analytic Theory of Heat”) in
1822 that the series that now bear his name were used in a systematic way to solve
problems in the theory and application of heat conduction. Much of the development
of mathematics in the nineteenth and well into the twentieth century was driven
by the desire to place Fourier’s methods on a rigorous foundation.
Fourier’s idea that almost all important functions that arise in mathematical
models of real-world phenomena can be represented as a superposition of
*Precalculus: A Problems-Oriented Approach, 7th edition