Student Seminar: Classical and Quantum Integrable Systems

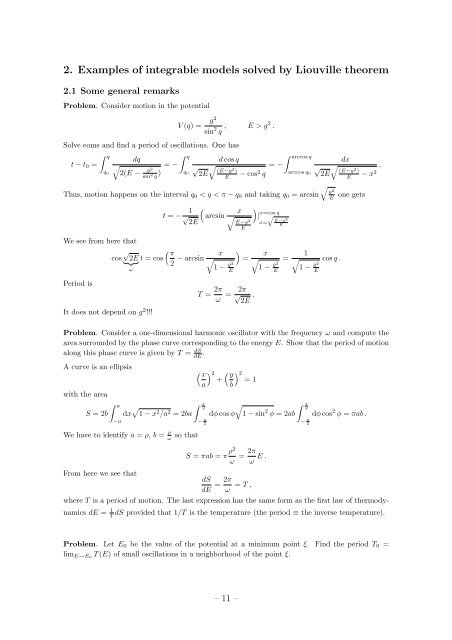
2. Examples of integrable models solved by Liouville theorem
2.1 Some general remarks
Problem. Consider motion in the potential
V (q) =
Solve eoms and find a period of oscillations. One has
t − t 0 =
∫ q
q 0
dq
√
= −
2(E − g2
sin 2 q )
∫ q
q 0
g2
sin 2 q , E > g2 .
d cos q
√ √
2E
(E−g 2 )
E
− cos 2 q
∫ arccos q
= −
arccos q 0
Thus, motion happens on the interval q 0 < q < π − q 0 and taking q 0 = arcsin
We see from here that
Period is
cos √ } {{
2E
}
ω
It does not depend on g 2 !!!
t = − 1 √
2E
(
arcsin
x
√
E−g 2
E
( π
t = cos
2 − arcsin x
)
√ =
1 − g2
E
T = 2π ω =
)
2π √
2E
.
| x=cos q
q
E−g
x=
2
E
x
√
1 − g2
E
dx
√ √ .
2E
(E−g 2 )
E
− x 2
√
g 2
E
= √
1
cos q .
1 − g2
E
one gets
Problem. Consider a one-dimensional harmonic oscillator with the frequency ω and compute the
area surrounded by the phase curve corresponding to the energy E. Show that the period of motion
along this phase curve is given by T = dS
dE .
A curve is an ellipsis
( x
a
) 2
+
( y
b
) 2
= 1
with the area
S = 2b
∫ a
−a
dx √ ∫ π √
1 − x 2 /a 2 2
= 2ba dφ cos φ 1 − sin 2 φ = 2ab
− π 2
∫ π
2
− π 2
dφ cos 2 φ = πab .
We have to identify a = ρ, b = ρ ω
so that
S = πab = π ρ2
ω = 2π ω E .
From here we see that
dS
dE = 2π ω = T ,
where T is a period of motion. The last expression has the same form as the first law of thermodynamics
dE = 1 T
dS provided that 1/T is the temperature (the period ≡ the inverse temperature).
Problem. Let E 0 be the value of the potential at a minimum point ξ. Find the period T 0 =
lim E→E0 T (E) of small oscillations in a neighborhood of the point ξ.
– 11 –