Student Seminar: Classical and Quantum Integrable Systems

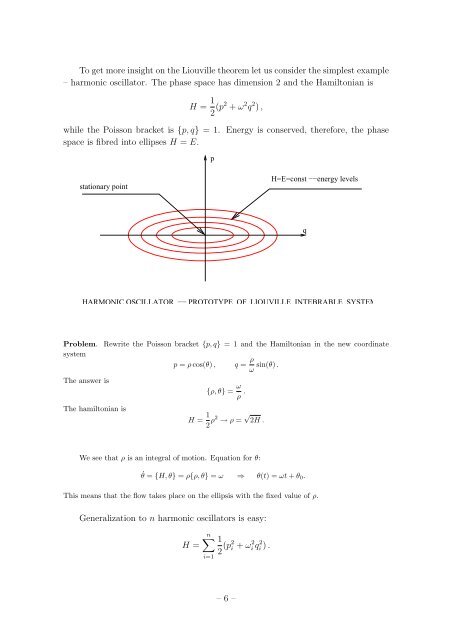
To get more insight on the Liouville theorem let us consider the simplest example
– harmonic oscillator. The phase space has dimension 2 and the Hamiltonian is
H = 1 2 (p2 + ω 2 q 2 ) ,
while the Poisson bracket is {p, q} = 1. Energy is conserved, therefore, the phase
space is fibred into ellipses H = E.
p
stationary point
H=E=const −−energy levels
q
HARMONIC OSCILLATOR −− PROTOTYPE OF LIOUVILLE INTEBRABLE SYSTEMS
Problem.
system
Rewrite the Poisson bracket {p, q} = 1 and the Hamiltonian in the new coordinate
p = ρ cos(θ) , q = ρ ω sin(θ) .
The answer is
The hamiltonian is
{ρ, θ} = ω ρ .
H = 1 2 ρ2 → ρ = √ 2H .
We see that ρ is an integral of motion. Equation for θ:
˙θ = {H, θ} = ρ{ρ, θ} = ω ⇒ θ(t) = ωt + θ 0 .
This means that the flow takes place on the ellipsis with the fixed value of ρ.
Generalization to n harmonic oscillators is easy:
H =
n∑
i=1
1
2 (p2 i + ω 2 i q 2 i ) .
– 6 –