Student Seminar: Classical and Quantum Integrable Systems

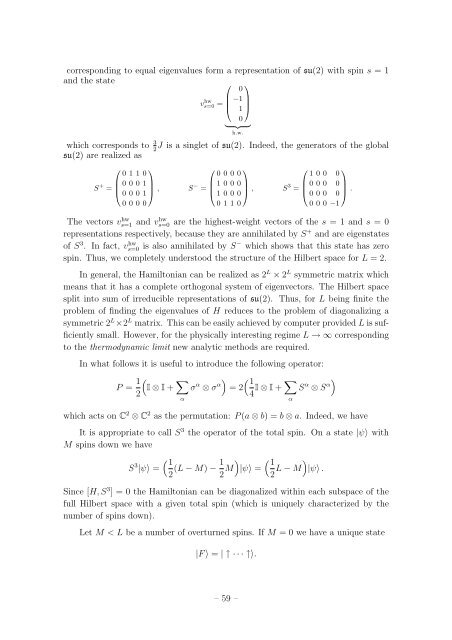
corresponding to equal eigenvalues form a representation of su(2) with spin s = 1
and the state
⎛ ⎞
0
vs=0 hw = ⎜ −1
⎟
⎝ 1 ⎠
}
0
{{ }
h.w.
which corresponds to 3 J is a singlet of su(2). Indeed, the generators of the global
2
su(2) are realized as
⎛ ⎞
⎛ ⎞
⎛ ⎞
0 1 1 0
0 0 0 0
1 0 0 0
S + = ⎜ 0 0 0 1
⎟
⎝ 0 0 0 1 ⎠ , S− = ⎜ 1 0 0 0
⎟
⎝ 1 0 0 0 ⎠ , S3 = ⎜ 0 0 0 0
⎟
⎝ 0 0 0 0 ⎠ .
0 0 0 0
0 1 1 0
0 0 0 −1
The vectors vs=1 hw and vs=0 hw are the highest-weight vectors of the s = 1 and s = 0
representations respectively, because they are annihilated by S + and are eigenstates
of S 3 . In fact, vs=0 hw is also annihilated by S − which shows that this state has zero
spin. Thus, we completely understood the structure of the Hilbert space for L = 2.
In general, the Hamiltonian can be realized as 2 L × 2 L symmetric matrix which
means that it has a complete orthogonal system of eigenvectors. The Hilbert space
split into sum of irreducible representations of su(2). Thus, for L being finite the
problem of finding the eigenvalues of H reduces to the problem of diagonalizing a
symmetric 2 L ×2 L matrix. This can be easily achieved by computer provided L is sufficiently
small. However, for the physically interesting regime L → ∞ corresponding
to the thermodynamic limit new analytic methods are required.
In what follows it is useful to introduce the following operator:
P = 1 2
(
I ⊗ I + ∑ α
) ( 1
σ α ⊗ σ α = 2
4 I ⊗ I + ∑ α
S α ⊗ S α )
which acts on C 2 ⊗ C 2 as the permutation: P (a ⊗ b) = b ⊗ a. Indeed, we have
It is appropriate to call S 3 the operator of the total spin. On a state |ψ〉 with
M spins down we have
S 3 |ψ〉 =
( 1
2 (L − M) − 1 ) ( 1
)
2 M |ψ〉 =
2 L − M |ψ〉 .
Since [H, S 3 ] = 0 the Hamiltonian can be diagonalized within each subspace of the
full Hilbert space with a given total spin (which is uniquely characterized by the
number of spins down).
Let M < L be a number of overturned spins. If M = 0 we have a unique state
|F 〉 = | ↑ · · · ↑〉.
– 59 –