Cryptology - Unofficial St. Mary's College of California Web Site

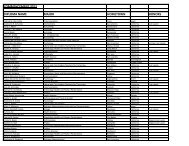
9.3. RECOGNIZING AND BREAKING POLYGRAPHIC CIPHERS 175
Repetition Start Positions Distance Factors
ELX 10 22 2 × 11
XEL 12 60 2 × 2 × 3 × 5
RQLXFKELRK 55 112 2 × 2 × 2 × 2 × 7
LRKQF 62 88 2 × 2 × 2 × 11
RLFER 28 56 2 × 2 × 2 × 7
QXKLK 117 56 2 × 2 × 2 × 7
LKF 120 44 2 × 2 × 11
Figure 9.4: Repetitions in the unknown cipher.
ones. And the large number of repetitions of length 2 at distances divisible by
2 would lead us to digraphic ciphers anyway. So digraphic it is.
Since we’ve recognized it, how do we break it To attack a digraphic cipher
we start as we always did – with a frequency count – except we count pairs of
letters, not singletons. The only pairs appearing more than twice are EL and
LX, 5 times each, and KO, QF and RK, 3 times each.
Now what We will grant ourselves the knowledge that it is a Hill cipher.
In many ways Hill ciphers are a fancy version of the Decimation and Linear
Ciphers from Chapters 3 and 4. They do do a better job of mixing up the
frequencies than those ciphers did, but, just as correctly guessing two letters of
a ciphertext enciphered with a Linear Cipher (and some math) leads to a decryption,
correctly guessing two bigrams (and some math) leads to a decryption
of a Hill cipher. (However, it is generally much harder to guess bigrams than
letters.) Here, the pairs EL and LX must be very common bigrams. If we know
which, we can solve for the enciphering matrix.
For ease, we will make a (miraculously correct) guess, that LX=th and
EL=at. This is, actually, fairly reasonable as th is the most common ( bigraph ) a b
and at is the eleventh most common. So there is some matrix with
c d
( ( ) ( ( ( ) ( a b L t a b E a
= and
= . Substituting the values for
c d)
X h)
c d)
L t)
( ) ( ) ( ) ( ( ) ( a b 12 20 a b 5 1
the letters, and we have
= and
= .
c d 24 8 c d)
12 20)
Multiplying out gives two sets of equations
12a + 24b = 20 and 5c + 12d = 20
12c + 24d = 8 5a + 12b = 1.
These( two sets ) of two equations in two unknowns may now be solved to see
9 5
that
is the original enciphering matrix. From here, the quote may
10 17