Blaga P. Lectures on the differential geometry of - tiera.ru
Blaga P. Lectures on the differential geometry of - tiera.ru
Blaga P. Lectures on the differential geometry of - tiera.ru
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
146 Chapter 4. General <strong>the</strong>ory <strong>of</strong> surfaces<br />
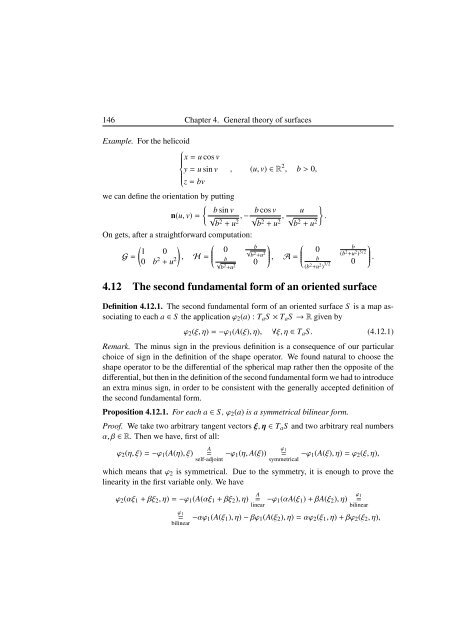
Example. For <strong>the</strong> helicoid<br />
⎧<br />
x = u cos v<br />
⎪⎨<br />
y = u sin v<br />
⎪⎩ z = bv<br />
we can define <strong>the</strong> orientati<strong>on</strong> by putting<br />
n(u, v) =<br />
, (u, v) ∈ R 2 , b > 0,<br />
�<br />
b sin v b cos v<br />
√ , − √<br />
b2 + u2 b2 + u2 ,<br />
On gets, after a straightforward computati<strong>on</strong>:<br />
�<br />
1<br />
G =<br />
0<br />
0<br />
b2 + u2 �<br />
,<br />
⎛<br />
H = ⎜⎝<br />
0 b<br />
0<br />
b<br />
√ b 2 +u 2<br />
√ b 2 +u 2<br />
⎞<br />
⎟⎠ , A =<br />
�<br />
u<br />
√ .<br />
b2 + u2 ⎛<br />
⎜⎝<br />
0<br />
b<br />
(b 2 +u 2 ) 3/2<br />
b<br />
(b2 +u2 ) 3/2<br />
4.12 The sec<strong>on</strong>d fundamental form <strong>of</strong> an oriented surface<br />
Definiti<strong>on</strong> 4.12.1. The sec<strong>on</strong>d fundamental form <strong>of</strong> an oriented surface S is a map associating<br />
to each a ∈ S <strong>the</strong> applicati<strong>on</strong> ϕ2(a) : TaS × TaS → R given by<br />
ϕ2(ξ, η) = −ϕ1(A(ξ), η), ∀ξ, η ∈ TaS. (4.12.1)<br />
Remark. The minus sign in <strong>the</strong> previous definiti<strong>on</strong> is a c<strong>on</strong>sequence <strong>of</strong> our particular<br />
choice <strong>of</strong> sign in <strong>the</strong> definiti<strong>on</strong> <strong>of</strong> <strong>the</strong> shape operator. We found natural to choose <strong>the</strong><br />
shape operator to be <strong>the</strong> <strong>differential</strong> <strong>of</strong> <strong>the</strong> spherical map ra<strong>the</strong>r <strong>the</strong>n <strong>the</strong> opposite <strong>of</strong> <strong>the</strong><br />
<strong>differential</strong>, but <strong>the</strong>n in <strong>the</strong> definiti<strong>on</strong> <strong>of</strong> <strong>the</strong> sec<strong>on</strong>d fundamental form we had to introduce<br />
an extra minus sign, in order to be c<strong>on</strong>sistent with <strong>the</strong> generally accepted definiti<strong>on</strong> <strong>of</strong><br />
<strong>the</strong> sec<strong>on</strong>d fundamental form.<br />
Propositi<strong>on</strong> 4.12.1. For each a ∈ S , ϕ2(a) is a symmetrical bilinear form.<br />
Pro<strong>of</strong>. We take two arbitrary tangent vectors ξ, η ∈ TaS and two arbitrary real numbers<br />
α, β ∈ R. Then we have, first <strong>of</strong> all:<br />
ϕ2(η, ξ) = −ϕ1(A(η), ξ)<br />
A<br />
=<br />
self-adjoint −ϕ1(η,<br />
ϕ1<br />
A(ξ)) =<br />
symmetrical −ϕ1(A(ξ), η) = ϕ2(ξ, η),<br />
which means that ϕ2 is symmetrical. Due to <strong>the</strong> symmetry, it is enough to prove <strong>the</strong><br />
linearity in <strong>the</strong> first variable <strong>on</strong>ly. We have<br />
ϕ2(αξ1 + βξ2, η) = −ϕ1(A(αξ1 + βξ2), η)<br />
A<br />
=<br />
linear −ϕ1(αA(ξ1) + βA(ξ2), η)<br />
0<br />
⎞<br />
ϕ1<br />
=<br />
bilinear<br />
ϕ1<br />
=<br />
bilinear −αϕ1(A(ξ1), η) − βϕ1(A(ξ2), η) = αϕ2(ξ1, η) + βϕ2(ξ2, η),<br />
⎟⎠ .