Blaga P. Lectures on the differential geometry of - tiera.ru

154 Chapter 4. General theory of surfaces
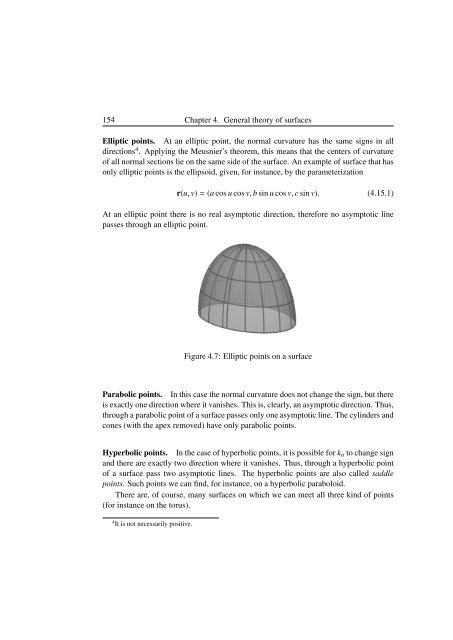
Elliptic points. At an elliptic point, the normal curvature has the same signs in all
directions 4 . Applying the Meusnier’s theorem, this means that the centers of curvature
of all normal sections lie on the same side of the surface. An example of surface that has
only elliptic points is the ellipsoid, given, for instance, by the parameterization
r(u, v) = (a cos u cos v, b sin u cos v, c sin v). (4.15.1)
At an elliptic point there is no real asymptotic direction, therefore no asymptotic line
passes through an elliptic point.
Figure 4.7: Elliptic points on a surface
Parabolic points. In this case the normal curvature does not change the sign, but there
is exactly one direction where it vanishes. This is, clearly, an asymptotic direction. Thus,
through a parabolic point of a surface passes only one asymptotic line. The cylinders and
cones (with the apex removed) have only parabolic points.
Hyperbolic points. In the case of hyperbolic points, it is possible for kn to change sign
and there are exactly two direction where it vanishes. Thus, through a hyperbolic point
of a surface pass two asymptotic lines. The hyperbolic points are also called saddle
points. Such points we can find, for instance, on a hyperbolic paraboloid.
There are, of course, many surfaces on which we can meet all three kind of points
(for instance on the torus).
4 It is not necessarily positive.