Blaga P. Lectures on the differential geometry of - tiera.ru

174 Chapter 4. General theory of surfaces
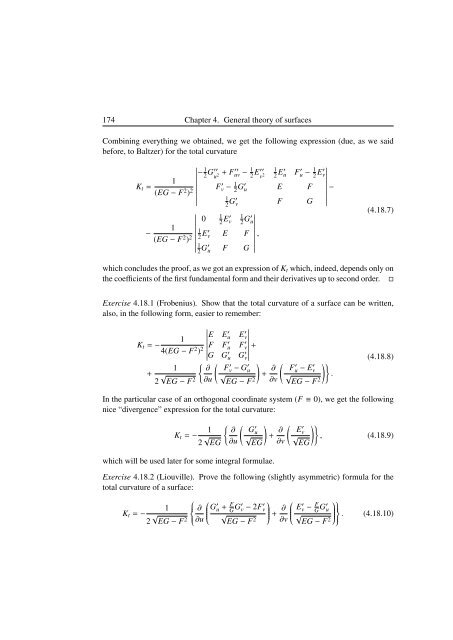
Combining everything we obtained, we get the following expression (due, as we said
before, to Baltzer) for the total curvature
Kt =
−
1
(EG − F2 ) 2
�
�
�
�
�
�
�
�
�
�
�
2G′ v F G
1
(EG − F2 ) 2
�
� 1
�
�
0 2
�
�
�
�
�
�
�
E′ 1
v 2G′ u
1
2 E′ v E F
1
2G′ �
�
�
�
�
�
�
� ,
�
�
u F G �
− 1
2G′′ u2 + F ′′
uv − 1
2 E′′
v2 1
2 E′ u F ′ u − 1
2 E′ v
F ′ v − 1
2G′ u
1
E F
�
�
�
�
�
�
�
� −
�
�
�
(4.18.7)
which concludes the proof, as we got an expression of Kt which, indeed, depends only on
the coefficients of the first fundamental form and their derivatives up to second order. �
Exercise 4.18.1 (Frobenius). Show that the total curvature of a surface can be written,
also, in the following form, easier to remember:
1
Kt = −
4(EG − F2 ) 2
�
�
�
�E
E
�
�
�
�
�
′ u E ′ v
F F ′ u F ′ v
G G ′ u G ′ �
�
�
�
�
�
� +
�
v�
1
+
2 √ EG − F2 � �
∂ F ′
v − G
∂u
′ �
u
√ +
EG − F2 ∂
�
F ′
u − E
∂v
′ ��
v
√ .
EG − F2 (4.18.8)
In the particular case of an orthogonal coordinate system (F ≡ 0), we get the following
nice “divergence” expression for the total curvature:
Kt = − 1
2 √ � �
∂ G ′ �
u
√ +
EG ∂u EG
∂
�
E ′ ��
v
√ , (4.18.9)
∂v EG
which will be used later for some integral formulae.
Exercise 4.18.2 (Liouville). Prove the following (slightly asymmetric) formula for the
total curvature of a surface:
1
Kt = −
2 √ EG − F2 ⎧ ⎛
⎪⎨ ∂ G
⎪⎩
⎜⎝
∂u
′ u + F
GG′ v − 2F ′ ⎞ ⎛
v ∂ E
√ ⎟⎠ + ⎜⎝
EG − F2 ∂v
′ v − F
GG′ ⎞⎫
u ⎪⎬
√ ⎟⎠
EG − F2 ⎪⎭
. (4.18.10)