nr. 477 - 2011 - Institut for Natur, Systemer og Modeller (NSM)

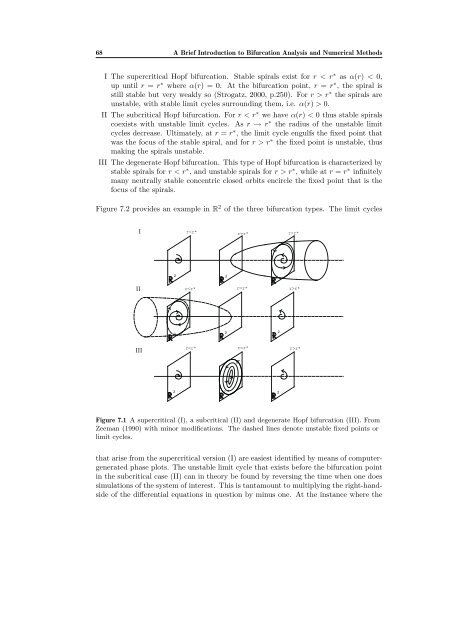
68 A Brief Introduction to Bifurcation Analysis and Numerical Methods
I The supercritical Hopf bifurcation. Stable spirals exist for r < r ∗ as α(r) < 0,
up until r = r ∗ where α(r) = 0. At the bifurcation point, r = r ∗ , the spiral is
still stable but very weakly so (Strogatz, 2000, p.250). For r > r ∗ the spirals are
unstable, with stable limit cycles surrounding them, i.e. α(r) > 0.
II The subcritical Hopf bifurcation. For r < r ∗ we have α(r) < 0 thus stable spirals
coexists with unstable limit cycles. As r → r ∗ the radius of the unstable limit
cycles decrease. Ultimately, at r = r ∗ , the limit cycle engulfs the fixed point that
was the focus of the stable spiral, and for r > r ∗ the fixed point is unstable, thus
making the spirals unstable.
III The degenerate Hopf bifurcation. This type of Hopf bifurcation is characterized by
stable spirals for r < r ∗ , and unstable spirals for r > r ∗ , while at r = r ∗ infinitely
many neutrally stable concentric closed orbits encircle the fixed point that is the
focus of the spirals.
Figure 7.2 provides an example in R 2 of the three bifurcation types. The limit cycles
I
II
III
2
rr*
2 2 2
rr*
2 2
2
rr*
Figure 7.1 A supercritical (I), a subcritical (II) and degenerate Hopf bifurcation (III). From
Zeeman (1990) with minor modifications. The dashed lines denote unstable fixed points or
limit cycles.
that arise from the supercritical version (I) are easiest identified by means of computergenerated
phase plots. The unstable limit cycle that exists before the bifurcation point
in the subcritical case (II) can in theory be found by reversing the time when one does
simulations of the system of interest. This is tantamount to multiplying the right-handside
of the differential equations in question by minus one. At the instance where the
2
2