Edge-connectivity of undirected and directed hypergraphs
Edge-connectivity of undirected and directed hypergraphs
Edge-connectivity of undirected and directed hypergraphs
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
Section 7.2. Augmentation to meet orientability requirements 121<br />
<br />
Z∈F2<br />
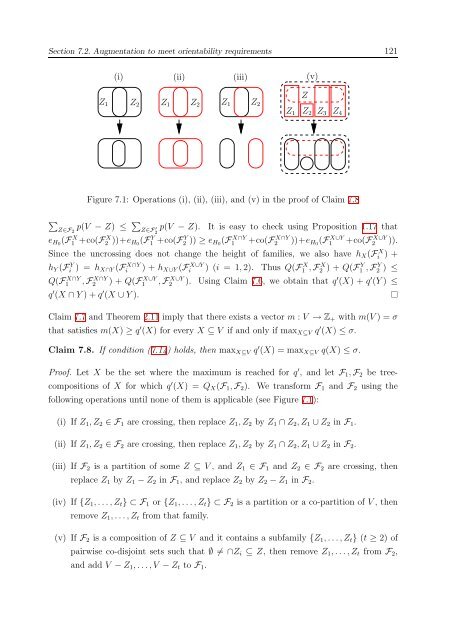
(i) (ii) (iii) (v)<br />
Z1 Z2 Z1 Z2 Z1 Z2<br />
Z<br />
Z1 Z2 Z3 Z4<br />
Figure 7.1: Operations (i), (ii), (iii), <strong>and</strong> (v) in the pro<strong>of</strong> <strong>of</strong> Claim 7.8<br />
<br />
p(V − Z) ≤ Z∈F ′ p(V − Z). It is easy to check using Proposition 1.17 that<br />
2<br />
eH0(F X 1 +co(F X 2 ))+eH0(F Y 1 +co(F Y 2 )) ≥ eH0(F X∩Y<br />
1<br />
+co(F X∩Y<br />
2 ))+eH0(F X∪Y<br />
1<br />
+co(F X∪Y<br />
2 )).<br />
Since the uncrossing does not change the height <strong>of</strong> families, we also have hX(F X i ) +<br />
hY (F Y i ) = hX∩Y (F X∩Y<br />
i ) + hX∪Y (F X∪Y<br />
i ) (i = 1, 2). Thus Q(F X 1 , F X 2 ) + Q(F Y 1 , F Y 2 ) ≤<br />
Q(F X∩Y<br />
1<br />
, F X∩Y<br />
2<br />
) + Q(F X∪Y<br />
1<br />
q ′ (X ∩ Y ) + q ′ (X ∪ Y ).<br />
, F X∪Y<br />
2 ). Using Claim 7.6, we obtain that q ′ (X) + q ′ (Y ) ≤<br />
Claim 7.7 <strong>and</strong> Theorem 2.11 imply that there exists a vector m : V → Z+ with m(V ) = σ<br />
that satisfies m(X) ≥ q ′ (X) for every X ⊆ V if <strong>and</strong> only if maxX⊆V q ′ (X) ≤ σ.<br />
Claim 7.8. If condition (7.14) holds, then maxX⊆V q ′ (X) = maxX⊆V q(X) ≤ σ.<br />
Pro<strong>of</strong>. Let X be the set where the maximum is reached for q ′ , <strong>and</strong> let F1, F2 be tree-<br />
compositions <strong>of</strong> X for which q ′ (X) = QX(F1, F2). We transform F1 <strong>and</strong> F2 using the<br />
following operations until none <strong>of</strong> them is applicable (see Figure 7.1):<br />
(i) If Z1, Z2 ∈ F1 are crossing, then replace Z1, Z2 by Z1 ∩ Z2, Z1 ∪ Z2 in F1.<br />
(ii) If Z1, Z2 ∈ F2 are crossing, then replace Z1, Z2 by Z1 ∩ Z2, Z1 ∪ Z2 in F2.<br />
(iii) If F2 is a partition <strong>of</strong> some Z ⊆ V , <strong>and</strong> Z1 ∈ F1 <strong>and</strong> Z2 ∈ F2 are crossing, then<br />
replace Z1 by Z1 − Z2 in F1, <strong>and</strong> replace Z2 by Z2 − Z1 in F2.<br />
(iv) If {Z1, . . . , Zt} ⊂ F1 or {Z1, . . . , Zt} ⊂ F2 is a partition or a co-partition <strong>of</strong> V , then<br />
remove Z1, . . . , Zt from that family.<br />
(v) If F2 is a composition <strong>of</strong> Z ⊆ V <strong>and</strong> it contains a subfamily {Z1, . . . , Zt} (t ≥ 2) <strong>of</strong><br />
pairwise co-disjoint sets such that ∅ = ∩Zi ⊆ Z, then remove Z1, . . . , Zt from F2,<br />
<strong>and</strong> add V − Z1, . . . , V − Zt to F1.