Edge-connectivity of undirected and directed hypergraphs

86 Chapter 5. Partition-connectivity of hypergraphs
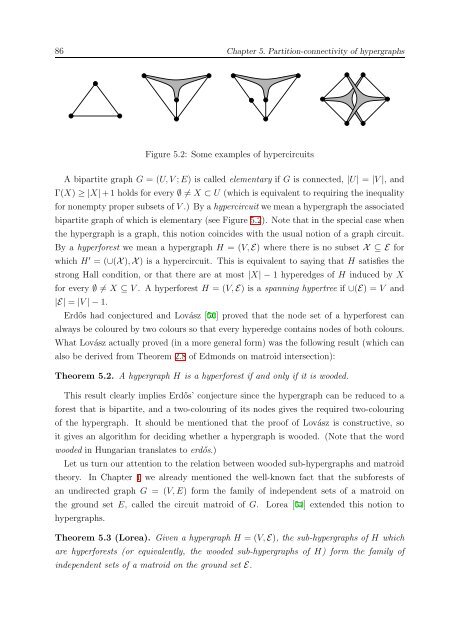
Figure 5.2: Some examples of hypercircuits
A bipartite graph G = (U, V ; E) is called elementary if G is connected, |U| = |V |, and
Γ(X) ≥ |X| + 1 holds for every ∅ = X ⊂ U (which is equivalent to requiring the inequality
for nonempty proper subsets of V .) By a hypercircuit we mean a hypergraph the associated
bipartite graph of which is elementary (see Figure 5.2). Note that in the special case when
the hypergraph is a graph, this notion coincides with the usual notion of a graph circuit.
By a hyperforest we mean a hypergraph H = (V, E) where there is no subset X ⊆ E for
which H ′ = (∪(X ), X ) is a hypercircuit. This is equivalent to saying that H satisfies the
strong Hall condition, or that there are at most |X| − 1 hyperedges of H induced by X
for every ∅ = X ⊆ V . A hyperforest H = (V, E) is a spanning hypertree if ∪(E) = V and
|E| = |V | − 1.
Erdős had conjectured and Lovász [56] proved that the node set of a hyperforest can
always be coloured by two colours so that every hyperedge contains nodes of both colours.
What Lovász actually proved (in a more general form) was the following result (which can
also be derived from Theorem 2.8 of Edmonds on matroid intersection):
Theorem 5.2. A hypergraph H is a hyperforest if and only if it is wooded.
This result clearly implies Erdős’ conjecture since the hypergraph can be reduced to a
forest that is bipartite, and a two-colouring of its nodes gives the required two-colouring
of the hypergraph. It should be mentioned that the proof of Lovász is constructive, so
it gives an algorithm for deciding whether a hypergraph is wooded. (Note that the word
wooded in Hungarian translates to erdős.)
Let us turn our attention to the relation between wooded sub-hypergraphs and matroid
theory. In Chapter 1 we already mentioned the well-known fact that the subforests of
an undirected graph G = (V, E) form the family of independent sets of a matroid on
the ground set E, called the circuit matroid of G. Lorea [54] extended this notion to
hypergraphs.
Theorem 5.3 (Lorea). Given a hypergraph H = (V, E), the sub-hypergraphs of H which
are hyperforests (or equivalently, the wooded sub-hypergraphs of H) form the family of
independent sets of a matroid on the ground set E.