Edge-connectivity of undirected and directed hypergraphs

Section 5.2. Tree-reducible hypergraphs 85
(a)
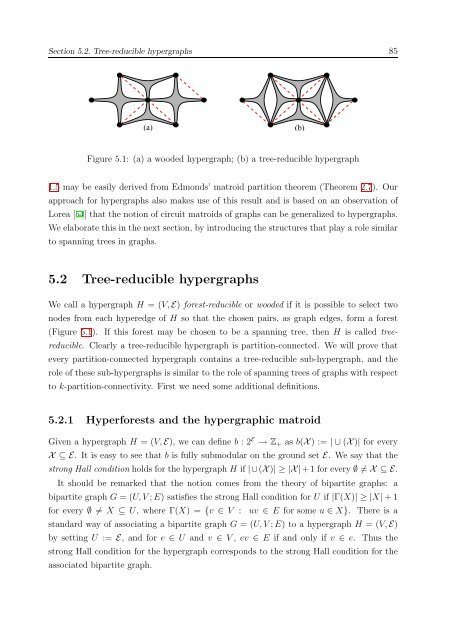
Figure 5.1: (a) a wooded hypergraph; (b) a tree-reducible hypergraph
1.5 may be easily derived from Edmonds’ matroid partition theorem (Theorem 2.7). Our
approach for hypergraphs also makes use of this result and is based on an observation of
Lorea [54] that the notion of circuit matroids of graphs can be generalized to hypergraphs.
We elaborate this in the next section, by introducing the structures that play a role similar
to spanning trees in graphs.
5.2 Tree-reducible hypergraphs
We call a hypergraph H = (V, E) forest-reducible or wooded if it is possible to select two
nodes from each hyperedge of H so that the chosen pairs, as graph edges, form a forest
(Figure 5.1). If this forest may be chosen to be a spanning tree, then H is called tree-
reducible. Clearly a tree-reducible hypergraph is partition-connected. We will prove that
every partition-connected hypergraph contains a tree-reducible sub-hypergraph, and the
role of these sub-hypergraphs is similar to the role of spanning trees of graphs with respect
to k-partition-connectivity. First we need some additional definitions.
5.2.1 Hyperforests and the hypergraphic matroid
Given a hypergraph H = (V, E), we can define b : 2 E → Z+ as b(X ) := | ∪ (X )| for every
X ⊆ E. It is easy to see that b is fully submodular on the ground set E. We say that the
strong Hall condition holds for the hypergraph H if |∪(X )| ≥ |X |+1 for every ∅ = X ⊆ E.
It should be remarked that the notion comes from the theory of bipartite graphs: a
bipartite graph G = (U, V ; E) satisfies the strong Hall condition for U if |Γ(X)| ≥ |X| + 1
for every ∅ = X ⊆ U, where Γ(X) = {v ∈ V : uv ∈ E for some u ∈ X}. There is a
standard way of associating a bipartite graph G = (U, V ; E) to a hypergraph H = (V, E)
by setting U := E, and for e ∈ U and v ∈ V , ev ∈ E if and only if v ∈ e. Thus the
strong Hall condition for the hypergraph corresponds to the strong Hall condition for the
associated bipartite graph.
(b)