Edge-connectivity of undirected and directed hypergraphs

More documents

Recommendations

Info

54 Chapter 3. Edge-connectivity augmentation of hypergraphs of adding ν-hyperedges (for a fixed ν) to a (k − 1)-edge-connected hypergraph to make it k-edge-connected. A hypergraph H covers a family C if dH(X) > 0 for every X ∈ C. A family C of sets is crossing if for any two crossing sets X, Y ∈ C, X ∩ Y and X ∪ Y are in C. Note that if C is also symmetric, then X − Y and Y − X are in C as well. If we define p(X) := 1 if X ∈ C and p(X) := −∞ otherwise, then p is crossing supermodular. If a hypergraph H0 is (k − 1)-edge-connected, then the sets ∅ = X ⊂ V for which k − dH0(X) = 1 form a symmetric crossing family, and a hypergraph H covers this family if and only if H0 + H is k-edge-connected. A partition F = {V1, . . . , Vl} is called a full partition if ∪i∈IVi ∈ C for every ∅ = I ⊂ {1, . . . , l}. In other words, it is full if it is p-full for the set function p defined above. Fleiner and Jordán proved the following theorem: Theorem 3.13 (Fleiner, Jordán [20]). Let C be a symmetric crossing family on the ground set V , and ν ≥ 2 an integer. Then C can be covered by γ ν-hyperedges if and only if and νγ ≥ max{|F| : F ⊆ C, F is a subpartititon of V }, (ν − 1)γ ≥ max{|F| − 1 : F is a full partititon}. Note that the covering by ν-hyperedges is equivalent to the problem of covering by hyperedges of size at most ν, since one can always take hyperedges of maximal size. The proof of Fleiner and Jordán is different from the proofs of the results cited previously, in that it does not solve a degree-specified result using some splitting-off technique, instead it depends on an analysis of the structure of symmetric crossing families. 3.4.2 Covering symmetric supermodular functions by uniform hypergraphs The main result of this chapter is a common generalization of the above mentioned results in [9] and [20] (Theorems 3.12 and 3.13), based on the approach of Benczúr and Frank. We give a min-max formula on the minimum number of ν-hyperedges that can cover a given symmetric, positively crossing supermodular set function. As in [9], the substantial part of the proof is a solution of the degree-specified problem (i.e. when the degree of each node v ∈ V is a prescribed value m(v)), which then easily leads to a min-max formula on the minimum number of new hyperedges needed.
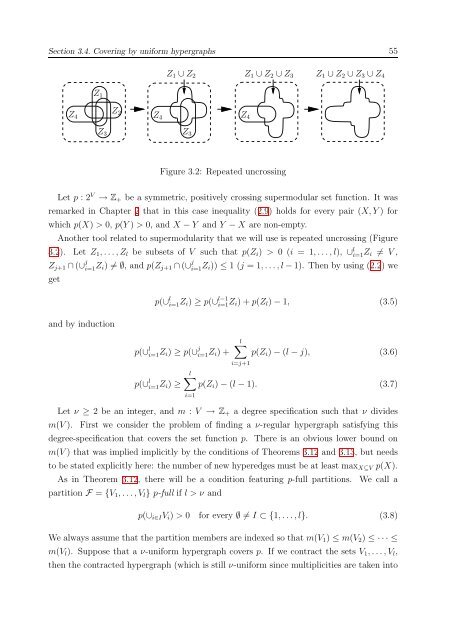
Section 3.4. Covering by uniform hypergraphs 55 Z4 Z1 Z3 Z2 Z4 Z1 ∪ Z2 Z3 Z1 ∪ Z2 ∪ Z3 Z4 Figure 3.2: Repeated uncrossing Z1 ∪ Z2 ∪ Z3 ∪ Z4 Let p : 2 V → Z+ be a symmetric, positively crossing supermodular set function. It was remarked in Chapter 2 that in this case inequality (2.9) holds for every pair (X, Y ) for which p(X) > 0, p(Y ) > 0, and X − Y and Y − X are non-empty. Another tool related to supermodularity that we will use is repeated uncrossing (Figure 3.2). Let Z1, . . . , Zl be subsets of V such that p(Zi) > 0 (i = 1, . . . , l), ∪l i=1Zi = V , Zj+1 ∩ (∪ j i=1Zi) = ∅, and p(Zj+1 ∩ (∪ j i=1Zi)) ≤ 1 (j = 1, . . . , l − 1). Then by using (2.2) we get and by induction p(∪ l i=1Zi) ≥ p(∪ l−1 i=1 Zi) + p(Zl) − 1, (3.5) p(∪ l i=1Zi) ≥ p(∪ j i=1 Zi) + p(∪ l i=1Zi) ≥ l i=j+1 p(Zi) − (l − j), (3.6) l p(Zi) − (l − 1). (3.7) i=1 Let ν ≥ 2 be an integer, and m : V → Z+ a degree specification such that ν divides m(V ). First we consider the problem of finding a ν-regular hypergraph satisfying this degree-specification that covers the set function p. There is an obvious lower bound on m(V ) that was implied implicitly by the conditions of Theorems 3.12 and 3.13, but needs to be stated explicitly here: the number of new hyperedges must be at least maxX⊆V p(X). As in Theorem 3.12, there will be a condition featuring p-full partitions. We call a partition F = {V1, . . . , Vl} p-full if l > ν and p(∪i∈IVi) > 0 for every ∅ = I ⊂ {1, . . . , l}. (3.8) We always assume that the partition members are indexed so that m(V1) ≤ m(V2) ≤ · · · ≤ m(Vl). Suppose that a ν-uniform hypergraph covers p. If we contract the sets V1, . . . , Vl, then the contracted hypergraph (which is still ν-uniform since multiplicities are taken into
Page 1:
Edge-connectivity of undirected and
Page 4 and 5: 4 Contents 6 Hypergraph orientation
Page 6 and 7: 6 Contents
Page 8 and 9: 8 Chapter 1. Introduction and preli
Page 10 and 11: 10 Chapter 1. Introduction and prel
Page 28 and 29: 28 Chapter 2. Submodular functions
Page 48 and 49: 48 Chapter 3. Edge-connectivity aug
Page 70 and 71: 70 Chapter 4. Connectivity augmenta
Page 84 and 85: 84 Chapter 5. Partition-connectivit
Page 96 and 97: 96 Chapter 6. Hypergraph orientatio
Page 98 and 99: 98 Chapter 6. Hypergraph orientatio
Page 100 and 101: 100 Chapter 6. Hypergraph orientati
Page 102 and 103: 102 Chapter 6. Hypergraph orientati
Page 104 and 105:
104 Chapter 6. Hypergraph orientati
Page 106 and 107:
Page 108 and 109:
Page 110 and 111:
Page 112 and 113:
Page 114 and 115:
Page 116 and 117:
116 Chapter 7. Combined augmentatio
Page 118 and 119:
Page 120 and 121:
Page 122 and 123:
Page 124 and 125:
Page 126 and 127:
Page 128 and 129:
Page 130 and 131:
130 Chapter 8. Concluding remarks w
Page 132 and 133:
132 Bibliography [11] E. Cheng, Edg
Page 134 and 135:
134 Bibliography [38] S. Fujishige,
Page 136 and 137:
136 Bibliography [66] A. Schrijver,
Page 138 and 139:
138 Index in-degree of node, 19 ind
Page 140 and 141:
140 Index h(a) the head node of the
Page 142 and 143:
142 Index
show all

Edge-connectivity of undirected and directed hypergraphs

Create successful ePaper yourself

Delete template?

Save as template?