Edge-connectivity of undirected and directed hypergraphs

72 Chapter 4. Connectivity augmentation of directed hypergraphs
(1, 0)
V V
V
(0, 1)
(0, 1)
z z
z
(0, 1) (0, 1)
(0, 1)
(1, 0) (1, 0)
(0, 1)
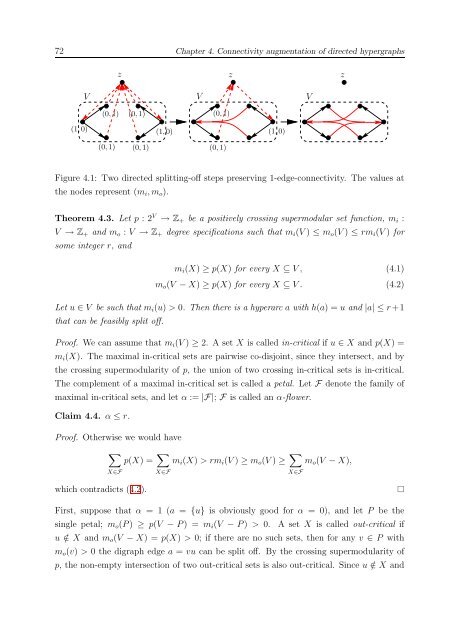
Figure 4.1: Two directed splitting-off steps preserving 1-edge-connectivity. The values at
the nodes represent (mi, mo).
Theorem 4.3. Let p : 2 V → Z+ be a positively crossing supermodular set function, mi :
V → Z+ and mo : V → Z+ degree specifications such that mi(V ) ≤ mo(V ) ≤ rmi(V ) for
some integer r, and
mi(X) ≥ p(X) for every X ⊆ V , (4.1)
mo(V − X) ≥ p(X) for every X ⊆ V . (4.2)
Let u ∈ V be such that mi(u) > 0. Then there is a hyperarc a with h(a) = u and |a| ≤ r +1
that can be feasibly split off.
Proof. We can assume that mi(V ) ≥ 2. A set X is called in-critical if u ∈ X and p(X) =
mi(X). The maximal in-critical sets are pairwise co-disjoint, since they intersect, and by
the crossing supermodularity of p, the union of two crossing in-critical sets is in-critical.
The complement of a maximal in-critical set is called a petal. Let F denote the family of
maximal in-critical sets, and let α := |F|; F is called an α-flower.
Claim 4.4. α ≤ r.
Proof. Otherwise we would have
p(X) =
mi(X) > rmi(V ) ≥ mo(V ) ≥
mo(V − X),
X∈F
which contradicts (4.2).
X∈F
First, suppose that α = 1 (a = {u} is obviously good for α = 0), and let P be the
single petal; mo(P ) ≥ p(V − P ) = mi(V − P ) > 0. A set X is called out-critical if
u /∈ X and mo(V − X) = p(X) > 0; if there are no such sets, then for any v ∈ P with
mo(v) > 0 the digraph edge a = vu can be split off. By the crossing supermodularity of
p, the non-empty intersection of two out-critical sets is also out-critical. Since u /∈ X and
X∈F