PHYS08200604018 Shamik Banerjee - Homi Bhabha National ...

36 CHAPTER 4. GENERALITIES OF QUARTER BPS DYON PARTITION FUNCTION
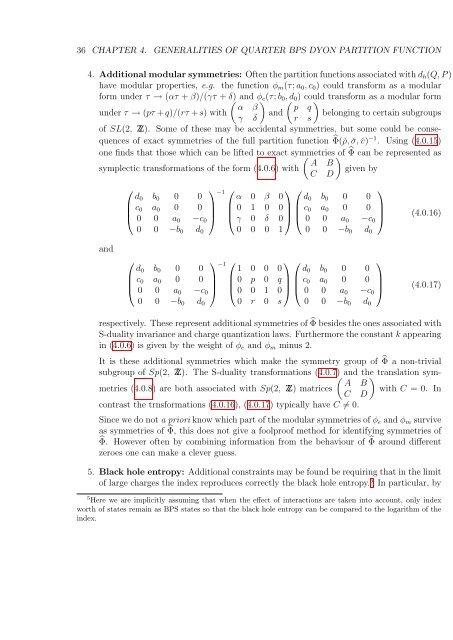
4. Additional modular symmetries: Often the partition functions associated with d h (Q, P )
have modular properties, e.g. the function φ m (τ; a 0 , c 0 ) could transform as a modular
form under τ → (ατ + β)/(γτ + δ) ( and φ)
e (τ; b 0 ,( d 0 ) could ) transform as a modular form
α β p q
under τ → (pτ + q)/(rτ + s) with and belonging to certain subgroups
γ δ r s
of SL(2, Z). Some of these may be accidental symmetries, but some could be consequences
of exact symmetries of the full partition function ̂Φ(ˇρ, ˇσ, ˇv) −1 . Using (4.0.15)
one finds that those which can be lifted to exact symmetries ( ) of ̂Φ can be represented as
A B
symplectic transformations of the form (4.0.6) with
given by
C D
⎛
⎞
d 0 b 0 0 0
⎜ c 0 a 0 0 0
⎟
⎝ 0 0 a 0 −c 0
⎠
0 0 −b 0 d 0
⎞ ⎛
⎞
α 0 β 0 d 0 b 0 0 0
⎜ 0 1 0 0
⎟ ⎜ c 0 a 0 0 0
⎟
⎝ γ 0 δ 0 ⎠ ⎝ 0 0 a 0 −c 0
⎠ (4.0.16)
0 0 0 1 0 0 −b 0 d 0
−1 ⎛
and
⎛
⎞
d 0 b 0 0 0
⎜ c 0 a 0 0 0
⎟
⎝ 0 0 a 0 −c 0
⎠
0 0 −b 0 d 0
⎞ ⎛
⎞
1 0 0 0 d 0 b 0 0 0
⎜ 0 p 0 q
⎟ ⎜ c 0 a 0 0 0
⎟
⎝ 0 0 1 0 ⎠ ⎝ 0 0 a 0 −c 0
⎠ (4.0.17)
0 r 0 s 0 0 −b 0 d 0
−1 ⎛
respectively. These represent additional symmetries of ̂Φ besides the ones associated with
S-duality invariance and charge quantization laws. Furthermore the constant k appearing
in (4.0.6) is given by the weight of φ e and φ m minus 2.
It is these additional symmetries which make the symmetry group of ̂Φ a non-trivial
subgroup of Sp(2, Z). The S-duality transformations (4.0.7) ( and the ) translation symmetries
(4.0.8) are both associated with Sp(2, Z) matrices
with C = 0. In
A B
C D
contrast the trnsformations (4.0.16), (4.0.17) typically have C ≠ 0.
Since we do not a priori know which part of the modular symmetries of φ e and φ m survive
as symmetries of ̂Φ, this does not give a foolproof method for identifying symmetries of
̂Φ. However often by combining information from the behaviour of ̂Φ around different
zeroes one can make a clever guess.
5. Black hole entropy: Additional constraints may be found be requiring that in the limit
of large charges the index reproduces correctly the black hole entropy. 5 In particular, by
5 Here we are implicitly assuming that when the effect of interactions are taken into account, only index
worth of states remain as BPS states so that the black hole entropy can be compared to the logarithm of the
index.