PHYS08200604018 Shamik Banerjee - Homi Bhabha National ...

72 CHAPTER 4. GENERALITIES OF QUARTER BPS DYON PARTITION FUNCTION
⎛
⎞
−1
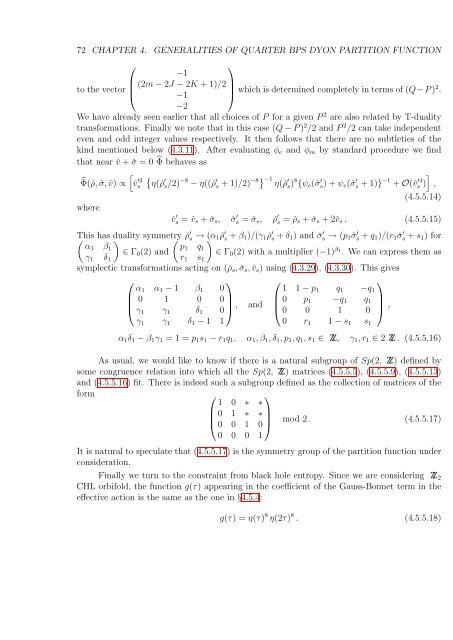
to the vector ⎜ (2m − 2J − 2K + 1)/2
⎟
⎝ −1 ⎠ which is determined completely in terms of (Q−P )2 .
−2
We have already seen earlier that all choices of P for a given P 2 are also related by T-duality
transformations. Finally we note that in this case (Q − P ) 2 /2 and P 2 /2 can take independent
even and odd integer values respectively. It then follows that there are no subtleties of the
kind mentioned below (4.3.11). After evaluating φ e and φ m by standard procedure we find
that near ˇv + ˇσ = 0 ̂Φ behaves as
[ {
̂Φ(ˇρ, ˇσ, ˇv) ∝ ˇv s
′2 η(ˇρ
′
s /2) −8 − η((ˇρ ′ s + 1)/2) −8} ]
−1
η(ˇρ
′
s ) 8 {ψ e (ˇσ s) ′ + ψ e (ˇσ s ′ + 1)} −1 + O(ˇv s ′4 ) ,
(4.5.5.14)
where
ˇv s ′ = ˇv s + ˇσ s , ˇσ s ′ = ˇσ s , ˇρ ′ s = ˇρ s + ˇσ s + 2ˇv s . (4.5.5.15)
( This has duality ) symmetry ( ˇρ ′ s → )(α 1 ˇρ ′ s + β 1 )/(γ 1 ˇρ ′ s + δ 1 ) and ˇσ s ′ → (p 1ˇσ s ′ + q 1 )/(r 1ˇσ s ′ + s 1 ) for
α1 β 1
p1 q
∈ Γ
γ 1 δ 0 (2) and
1
∈ Γ
1 r 1 s 0 (2) with a multiplier (−1) β 1
. We can express them as
1
symplectic transformations acting on (ˇρ s , ˇσ s , ˇv s ) using (4.3.29), (4.3.30). This gives
⎛
⎞ ⎛
⎞
α 1 α 1 − 1 β 1 0
1 1 − p 1 q 1 −q 1
⎜ 0 1 0 0
⎟
⎝ γ 1 γ 1 δ 1 0 ⎠ , and ⎜ 0 p 1 −q 1 q 1
⎟
⎝ 0 0 1 0 ⎠ ,
γ 1 γ 1 δ 1 − 1 1
0 r 1 1 − s 1 s 1
α 1 δ 1 − β 1 γ 1 = 1 = p 1 s 1 − r 1 q 1 , α 1 , β 1 , δ 1 , p 1 , q 1 , s 1 ∈ Z, γ 1 , r 1 ∈ 2 Z . (4.5.5.16)
As usual, we would like to know if there is a natural subgroup of Sp(2, Z) defined by
some congruence relation into which all the Sp(2, Z) matrices (4.5.5.5), (4.5.5.9), (4.5.5.13)
and (4.5.5.16) fit. There is indeed such a subgroup defined as the collection of matrices of the
form
⎛
⎞
1 0 ∗ ∗
⎜ 0 1 ∗ ∗
⎟
⎝ 0 0 1 0 ⎠ mod 2 . (4.5.5.17)
0 0 0 1
It is natural to speculate that (4.5.5.17) is the symmetry group of the partition function under
consideration.
Finally we turn to the constraint from black hole entropy. Since we are considering Z 2
CHL orbifold, the function g(τ) appearing in the coefficient of the Gauss-Bonnet term in the
effective action is the same as the one in §4.5.4:
g(τ) = η(τ) 8 η(2τ) 8 . (4.5.5.18)