PHYS08200604018 Shamik Banerjee - Homi Bhabha National ...

4.5. EXAMPLES 53
Next we turn to the constraints from the wall crossing formula. In this case all the
walls are related by S-duality transformation to the wall corresponding to the decay (Q, P ) →
(Q, 0) + (0, P ). So it is sufficient to study the consequences of the wall crossing formula at this
wall. Clearly Q 2 and P 2 given in (4.5.1.4) are uncorrelated. Furthermore in heterotic string
theory on T 6 all Q’s with a given Q 2 are related by T -duality transformation [41]. The same
is true for P . Thus the subtleties mentioned below eq.(4.3.11) are absent, and the behaviour
of ̂Φ(ˇρ, ˇσ, ˇv) near ˇv = 0 is expected to be given by (4.3.9). In this case both the electric and
the magnetic half-BPS partition functions are given by η(τ) −24 where η denotes the Dedekind
function. Thus we have, as a consequence of the wall crossing formula,
̂Φ(ˇρ, ˇσ, ˇv) ∝ {ˇv 2 (η(ˇρ)) 24 (η(ˇσ)) 24 + O(ˇv 4 )} . (4.5.1.7)
η(τ) 24 transforms as a modular form of weight 12 under an SL(2, Z) transformation. From
eqs.(4.3.26) it follows that these SL(2, Z) transformations may be regarded as the following
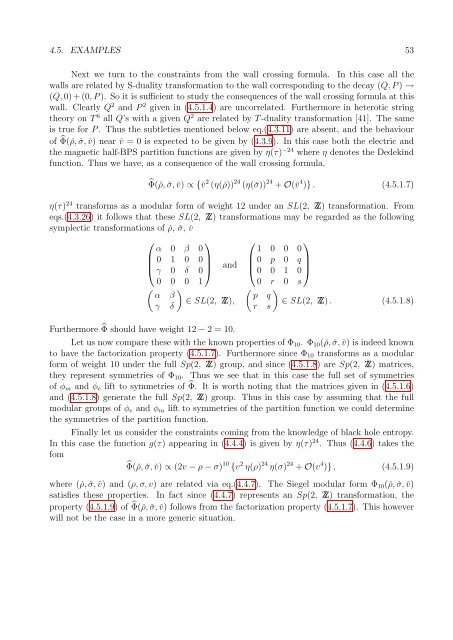
symplectic transformations of ˇρ, ˇσ, ˇv
Furthermore ̂Φ should have weight 12 − 2 = 10.
⎛
⎞ ⎛
⎞
α 0 β 0
1 0 0 0
⎜ 0 1 0 0
⎟
⎝ γ 0 δ 0 ⎠ and ⎜ 0 p 0 q
⎟
⎝ 0 0 1 0 ⎠
0 0 0 1
0 r 0 s
( )
( )
α β
p q
∈ SL(2, Z),
∈ SL(2, Z) . (4.5.1.8)
γ δ
r s
Let us now compare these with the known properties of Φ 10 . Φ 10 (ˇρ, ˇσ, ˇv) is indeed known
to have the factorization property (4.5.1.7). Furthermore since Φ 10 transforms as a modular
form of weight 10 under the full Sp(2, Z) group, and since (4.5.1.8) are Sp(2, Z) matrices,
they represent symmetries of Φ 10 . Thus we see that in this case the full set of symmetries
of φ m and φ e lift to symmetries of ̂Φ. It is worth noting that the matrices given in (4.5.1.6)
and (4.5.1.8) generate the full Sp(2, Z) group. Thus in this case by assuming that the full
modular groups of φ e and φ m lift to symmetries of the partition function we could determine
the symmetries of the partition function.
Finally let us consider the constraints coming from the knowledge of black hole entropy.
In this case the function g(τ) appearing in (4.4.4) is given by η(τ) 24 . Thus (4.4.6) takes the
fom
̂Φ(ˇρ, ˇσ, ˇv) ∝ (2v − ρ − σ) 10 {v 2 η(ρ) 24 η(σ) 24 + O(v 4 )} , (4.5.1.9)
where (ˇρ, ˇσ, ˇv) and (ρ, σ, v) are related via eq.(4.4.7). The Siegel modular form Φ 10 (ˇρ, ˇσ, ˇv)
satisfies these properties. In fact since (4.4.7) represents an Sp(2, Z) transformation, the
property (4.5.1.9) of ̂Φ(ˇρ, ˇσ, ˇv) follows from the factorization property (4.5.1.7). This however
will not be the case in a more generic situation.