PHYS08200604018 Shamik Banerjee - Homi Bhabha National ...

More documents

Recommendations

Info

54 CHAPTER 4. GENERALITIES OF QUARTER BPS DYON PARTITION FUNCTION 4.5.2 Dyons with unit torsion and even Q 2 /2 in heterotic on T 6 We now consider again heterotic string theory on T 6 , but choose the set A to be collection of (Q, P ) of the form: ⎛ ⎞ ⎛ ⎞ 0 K Q = ⎜ 2m ⎟ ⎝ 0 ⎠ , P = ⎜ J ⎟ ⎝ 1 ⎠ , m, K, J ∈ Z . (4.5.2.1) −1 0 This has Q 2 = −4m, P 2 = 2K, Q · P = −J . (4.5.2.2) We note that all the charge vectors have Q 2 /2 even. Since Q 2 is T-duality invariant, any other charge vector which can be obtained from this one by a T-duality transformation has Q 2 /2 even. Thus the set B now consists of charge vectors which have even Q 2 /2 and arbitrary integer values of P 2 /2 and Q · P . Since this set B is a subset of charges for which the spectrum was analyzed in §4.5.1 we do not expect to derive any new results. Nevertheless we have chosen this example as this will serve as a useful guide to our analysis in later sections. We first note that the quantization conditions of Q 2 , P 2 and Q · P imply the following periods of the partition function: (ˇρ, ˇσ, ˇv) → (ˇρ + a 1 , ˇσ + a 2 , ˇv + a 3 ), a 1 ∈ Z, a 2 ∈ 1 2 Z, a 3 ∈ Z . (4.5.2.3) The period along ˇσ is not an integer. We can remedy this by defining Q s = Q/2 , ˇσ s = 4ˇσ, ˇv s = 2ˇv . (4.5.2.4) so that Q 2 s/2 and Q s · P are now quantized in half integer units. The periods (ã 1 , ã 2 , ã 3 ) of the variables (ˇρ, ˇσ s , ˇv s ) conjugate to (P 2 s /2, Q 2 s/2, Q s · P s ) are now integers, given by, ã 1 ∈ Z, ã 2 ∈ 2 Z, ã 3 ∈ 2 Z . (4.5.2.5) The dyon partition function in this case can be easily calculated from the one for §4.5.1 by taking into account the evenness of Q 2 /2. This amounts to adding to the original partition function another term where ˇσ is shifted by 1/2. Thus we have 1 ̂Φ(ˇρ, ˇσ, ˇv) = 1 2 ( ) 1 Φ 10 (ˇρ, ˇσ, ˇv) + 1 Φ 10 (ˇρ, ˇσ + 1, ˇv) , (4.5.2.6) 2 or, in terms of the rescaled variables, 1 ̂Φ(ˇρ, ˇσ, ˇv) = 1 ( ) 1 2 Φ 10 (ˇρ, 1 ˇσ 4 s, 1 ˇv 2 s) + 1 Φ 10 (ˇρ, 1 ˇσ 4 s + 1, 1 ˇv . (4.5.2.7) 2 2 s)
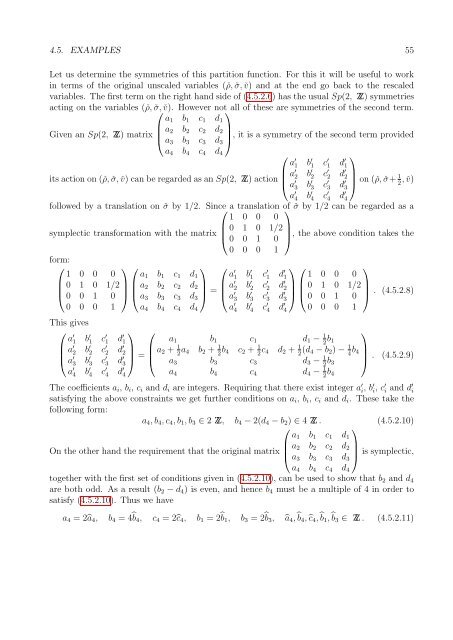
4.5. EXAMPLES 55 Let us determine the symmetries of this partition function. For this it will be useful to work in terms of the original unscaled variables (ˇρ, ˇσ, ˇv) and at the end go back to the rescaled variables. The first term on the right hand side of (4.5.2.6) has the usual Sp(2, Z) symmetries acting on the variables (ˇρ, ˇσ, ⎛ˇv). However not⎞ all of these are symmetries of the second term. a 1 b 1 c 1 d 1 Given an Sp(2, Z) matrix ⎜ a 2 b 2 c 2 d 2 ⎟ ⎝ a 3 b 3 c 3 d 3 ⎠ , it is a symmetry of the second term provided a 4 b 4 c 4 d 4 ⎛ ⎞ a ′ 1 b ′ 1 c ′ 1 d ′ 1 its action on (ˇρ, ˇσ, ˇv) can be regarded as an Sp(2, Z) action ⎜ a ′ 2 b ′ 2 c ′ 2 d ′ 2 ⎟ ⎝ a ′ 3 b ′ 3 c ′ 3 d ′ ⎠ on (ˇρ, ˇσ+ 1, ˇv) 2 3 a ′ 4 b ′ 4 c ′ 4 d ′ 4 followed by a translation on ˇσ by 1/2. Since ⎛ a translation of ⎞ ˇσ by 1/2 can be regarded as a 1 0 0 0 symplectic transformation with the matrix ⎜ 0 1 0 1/2 ⎟ ⎝ 0 0 1 0 ⎠ , the above condition takes the 0 0 0 1 form: ⎛ ⎞ ⎛ ⎞ ⎛ ⎞ ⎛ ⎞ 1 0 0 0 a 1 b 1 c 1 d 1 a ′ 1 b ′ 1 c ′ 1 d ′ 1 1 0 0 0 ⎜ 0 1 0 1/2 ⎟ ⎜ a 2 b 2 c 2 d 2 ⎟ ⎝ 0 0 1 0 ⎠ ⎝ a 3 b 3 c 3 d 3 ⎠ = ⎜ a ′ 2 b ′ 2 c ′ 2 d ′ 2 ⎟ ⎜ 0 1 0 1/2 ⎟ ⎝ a ′ 3 b ′ 3 c ′ 3 d ′ ⎠ ⎝ 3 0 0 1 0 ⎠ . (4.5.2.8) 0 0 0 1 a 4 b 4 c 4 d 4 a ′ 4 b ′ 4 c ′ 4 d ′ 4 0 0 0 1 This gives ⎛ ⎞ ⎛ a ′ 1 b ′ 1 c ′ 1 d ′ 1 a 1 b 1 c 1 d 1 − 1 ⎜ a ′ 2 b ′ 2 c ′ 2 d ′ 2 ⎟ ⎝ a ′ 3 b ′ 3 c ′ 3 d ′ ⎠ = b 2 1 ⎜ a 2 + 1 ⎝ a 2 4 b 2 + 1b 2 4 c 2 + 1c 2 4 d 2 + 1(d 2 4 − b 2 ) − 1b 4 4 3 a 3 b 3 c 3 d 3 − 1b a ′ 4 b ′ 4 c ′ 4 d ′ 2 3 4 a 4 b 4 c 4 d 4 − 1b 2 4 ⎞ ⎟ ⎠ . (4.5.2.9) The coefficients a i , b i , c i and d i are integers. Requiring that there exist integer a ′ i, b ′ i, c ′ i and d ′ i satisfying the above constraints we get further conditions on a i , b i , c i and d i . These take the following form: a 4 , b 4 , c 4 , b 1 , b 3 ∈ 2 Z, b 4 − 2(d 4 − b 2 ) ∈ 4 Z . (4.5.2.10) ⎛ a 1 b 1 c 1 d 1 On the other hand the requirement that the original matrix ⎜ a 2 b 2 c 2 d 2 ⎟ ⎝ a 3 b 3 c 3 d 3 ⎠ is symplectic, a 4 b 4 c 4 d 4 ⎞ together with the first set of conditions given in (4.5.2.10), can be used to show that b 2 and d 4 are both odd. As a result (b 2 − d 4 ) is even, and hence b 4 must be a multiple of 4 in order to satisfy (4.5.2.10). Thus we have a 4 = 2â 4 , b 4 = 4̂b 4 , c 4 = 2ĉ 4 , b 1 = 2̂b 1 , b 3 = 2̂b 3 , â 4 ,̂b 4 , ĉ 4 ,̂b 1 ,̂b 3 ∈ Z . (4.5.2.11)
Page 1:
Counting Microscopic Degeneracy of
Page 5:
Declaration This thesis is a presen
Page 9:
Acknowledgments Firstly I would lik
Page 13 and 14:
Synopsis Black Holes are classical
Page 15:
proposed formula reproduces the kno
Page 19 and 20:
Contents 1 Introduction 1 1.1 Broad
Page 23 and 24:
Chapter 1 Introduction Einstein pro
Page 25 and 26: 1.2. D BRANES 3 specific class of s
Page 27 and 28: 1.3. BRIEF INTRODUCTION TO E 8 × E
Page 29 and 30: 1.4. DEGENERACY OF QUARTER-BPS DYON
Page 31 and 32: 1.5. MOTIVATION AND A BRIEF REVIEW
Page 33 and 34: Chapter 2 Duality orbits, dyon spec
Page 35 and 36: 2.2. T-DUALITY ORBITS OF DYON CHARG
Page 41 and 42: 2.3. AN ALTERNATIVE PROOF 19 Q and
Page 43 and 44: 2.4. PREDICTIONS FOR GAUGE THEORY 2
Page 45 and 46: 2.4. PREDICTIONS FOR GAUGE THEORY 2
Page 47 and 48: Chapter 3 S-duality Action on Discr
Page 49 and 50: This proves the desired result. Nex
Page 51 and 52: For appropriate choice of (α, β,
Page 53 and 54: Chapter 4 Generalities of Quarter B
Page 55 and 56: may not be convergent in another re
Page 57 and 58: 35 where Λ is a large positive num
Page 59 and 60: equiring that we reproduce the blac
Page 61 and 62: 4.1. THE DYON PARTITION FUNCTION 39
Page 63 and 64: 4.2. CONSEQUENCES OF S-DUALITY SYMM
Page 65 and 66: 4.3. CONSTRAINTS FROM WALL CROSSING
Page 73 and 74: 4.5. EXAMPLES 51 Here k is the same
Page 75: 4.5. EXAMPLES 53 Next we turn to th
Page 79 and 80: 4.5. EXAMPLES 57 We shall now set a
Page 81 and 82: 4.5. EXAMPLES 59 uncorrelated and c
Page 83 and 84: 4.5. EXAMPLES 61 is an integer. Sin
Page 85 and 86: 4.5. EXAMPLES 63 1 ((Q−P 2 )/2)2
Page 87 and 88: 4.5. EXAMPLES 65 Finally we turn to
Page 89 and 90: 4.5. EXAMPLES 67 IIB description of
Page 91 and 92: 4.5. EXAMPLES 69 Note that Q and P
Page 93 and 94: 4.5. EXAMPLES 71 On the other hand
Page 95 and 96: 4.6. A PROPOSAL FOR THE PARTITION F
Page 103 and 104: 4.7. REVERSE APPLICATIONS 81 Thus i
Page 105 and 106: 4.7. REVERSE APPLICATIONS 83 For th
Page 107 and 108: Chapter 5 Partition Functions of To
Page 109 and 110: crossing formula for decay into a p
Page 111 and 112: 89 As a consequence of (5.0.19) and
Page 113 and 114: 91 In the primed variables the desi
Page 115 and 116: Furthermore, in order to reproduce
Page 117 and 118: The spectrum in gauge theory contai
Page 119 and 120: Chapter 6 Concluding Remarks Comple
Page 121 and 122: Bibliography [1] J.Polchinski ”Di
Page 123 and 124: BIBLIOGRAPHY 101 [30] M. Stern and
Page 125 and 126: BIBLIOGRAPHY 103 [63] S. Banerjee a
show all

PHYS08200604018 Shamik Banerjee - Homi Bhabha National ...

Create successful ePaper yourself

Delete template?

Save as template?