PHYS08200604018 Shamik Banerjee - Homi Bhabha National ...

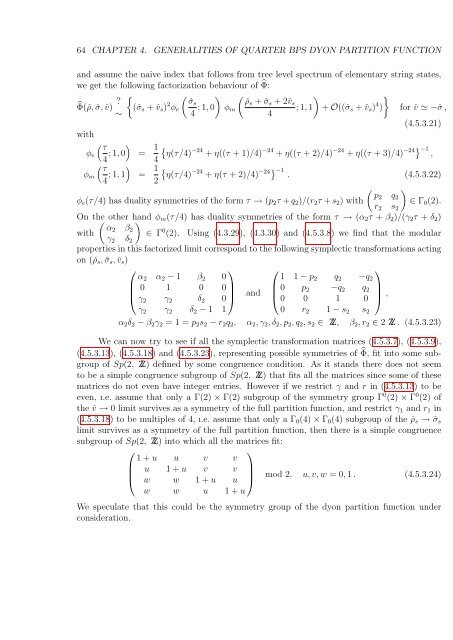
64 CHAPTER 4. GENERALITIES OF QUARTER BPS DYON PARTITION FUNCTION
and assume the naive index that follows from tree level spectrum of elementary string states,
we get the following factorization behaviour of ̂Φ:
̂Φ(ˇρ, ˇσ, ˇv) ? {
( ) ( )
}
(ˇσ s + ˇv s ) 2 ˇσs
φ e
∼
4 ; 1, 0 ˇρs + ˇσ s + 2ˇv s
φ m ; 1, 1 + O((ˇσ s + ˇv s ) 4 ) for ˇv ≃ −ˇσ ,
4
(4.5.3.21)
with
( τ
)
φ e
4 ; 1, 0 = 1 {
η(τ/4) −24 + η((τ + 1)/4) −24 + η((τ + 2)/4) −24 + η((τ + 3)/4) −24} −1
,
4
( τ
)
φ m
4 ; 1, 1 = 1 {
η(τ/4) −24 + η(τ + 2)/4) −24} −1
. (4.5.3.22)
2
( )
p2 q
φ e (τ/4) has duality symmetries of the form τ → (p 2 τ + q 2 )/(r 2 τ + s 2 ) with
2
∈ Γ
r 2 s 0 (2).
2
On the ( other hand ) φ m (τ/4) has duality symmetries of the form τ → (α 2 τ + β 2 )/(γ 2 τ + δ 2 )
α2 β
with
2
∈ Γ
γ 2 δ 0 (2). Using (4.3.29), (4.3.30) and (4.5.3.8) we find that the modular
2
properties in this factorized limit correspond to the following symplectic transformations acting
on (ˇρ s , ˇσ s , ˇv s )
⎛
⎞ ⎛
⎞
α 2 α 2 − 1 β 2 0
1 1 − p 2 q 2 −q 2
⎜ 0 1 0 0
⎟
⎝ γ 2 γ 2 δ 2 0 ⎠ and ⎜ 0 p 2 −q 2 q 2
⎟
⎝ 0 0 1 0 ⎠ ,
γ 2 γ 2 δ 2 − 1 1
0 r 2 1 − s 2 s 2
α 2 δ 2 − β 2 γ 2 = 1 = p 2 s 2 − r 2 q 2 , α 2 , γ 2 , δ 2 , p 2 , q 2 , s 2 ∈ Z, β 2 , r 2 ∈ 2 Z . (4.5.3.23)
We can now try to see if all the symplectic transformation matrices (4.5.3.7), (4.5.3.9),
(4.5.3.13), (4.5.3.18) and (4.5.3.23), representing possible symmetries of ̂Φ, fit into some subgroup
of Sp(2, Z) defined by some congruence condition. As it stands there does not seem
to be a simple congruence subgroup of Sp(2, Z) that fits all the matrices since some of these
matrices do not even have integer entries. However if we restrict γ and r in (4.5.3.13) to be
even, ı.e. assume that only a Γ(2) × Γ(2) subgroup of the symmetry group Γ 0 (2) × Γ 0 (2) of
the ˇv → 0 limit survives as a symmetry of the full partition function, and restrict γ 1 and r 1 in
(4.5.3.18) to be multiples of 4, ı.e. assume that only a Γ 0 (4) × Γ 0 (4) subgroup of the ˇρ s → ˇσ s
limit survives as a symmetry of the full partition function, then there is a simple congruence
subgroup of Sp(2, Z) into which all the matrices fit:
⎛
⎜
⎝
1 + u u v v
u 1 + u v v
w w 1 + u u
w w u 1 + u
⎞
⎟
⎠
mod 2, u, v, w = 0, 1 . (4.5.3.24)
We speculate that this could be the symmetry group of the dyon partition function under
consideration.