PHYS08200604018 Shamik Banerjee - Homi Bhabha National ...

More documents

Recommendations

Info

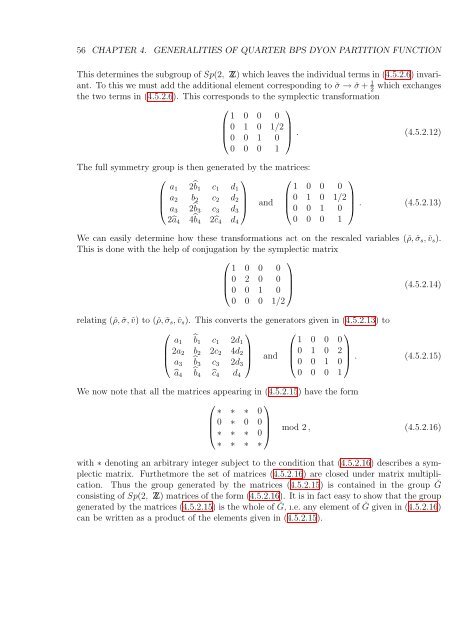
56 CHAPTER 4. GENERALITIES OF QUARTER BPS DYON PARTITION FUNCTION This determines the subgroup of Sp(2, Z) which leaves the individual terms in (4.5.2.6) invariant. To this we must add the additional element corresponding to ˇσ → ˇσ + 1 which exchanges 2 the two terms in (4.5.2.6). This corresponds to the symplectic transformation ⎛ ⎞ 1 0 0 0 ⎜ 0 1 0 1/2 ⎟ ⎝ 0 0 1 0 ⎠ . (4.5.2.12) 0 0 0 1 The full symmetry group is then generated by the matrices: ⎛ ⎞ ⎛ ⎞ a 1 2̂b 1 c 1 d 1 1 0 0 0 ⎜ a 2 b 2 c 2 d 2 ⎟ ⎝ a 3 2̂b 3 c 3 d 3 ⎠ and ⎜ 0 1 0 1/2 ⎟ ⎝ 0 0 1 0 ⎠ . (4.5.2.13) 2â 4 4̂b 4 2ĉ 4 d 4 0 0 0 1 We can easily determine how these transformations act on the rescaled variables (ˇρ, ˇσ s , ˇv s ). This is done with the help of conjugation by the symplectic matrix ⎛ ⎞ 1 0 0 0 ⎜ 0 2 0 0 ⎟ ⎝ 0 0 1 0 ⎠ (4.5.2.14) 0 0 0 1/2 relating (ˇρ, ˇσ, ˇv) to (ˇρ, ˇσ s , ˇv s ). This converts the generators given in (4.5.2.13) to ⎛ ⎞ ⎛ ⎞ a 1 ̂b1 c 1 2d 1 1 0 0 0 ⎜ 2a 2 b 2 2c 2 4d 2 ⎟ ⎝ a 3 ̂b3 c 3 2d 3 ⎠ and ⎜ 0 1 0 2 ⎟ ⎝ 0 0 1 0 ⎠ . (4.5.2.15) â 4 ̂b4 ĉ 4 d 4 0 0 0 1 We now note that all the matrices appearing in (4.5.2.15) have the form ⎛ ⎞ ∗ ∗ ∗ 0 ⎜ 0 ∗ 0 0 ⎟ ⎝ ∗ ∗ ∗ 0 ⎠ mod 2 , (4.5.2.16) ∗ ∗ ∗ ∗ with ∗ denoting an arbitrary integer subject to the condition that (4.5.2.16) describes a symplectic matrix. Furthetmore the set of matrices (4.5.2.16) are closed under matrix multiplication. Thus the group generated by the matrices (4.5.2.15) is contained in the group Ǧ consisting of Sp(2, Z) matrices of the form (4.5.2.16). It is in fact easy to show that the group generated by the matrices (4.5.2.15) is the whole of Ǧ, ı.e. any element of Ǧ given in (4.5.2.16) can be written as a product of the elements given in (4.5.2.15).
4.5. EXAMPLES 57 We shall now set aside this result for a while and study the implications of S-duality symmetry and the wall crossing formula on the partition function. The eventual goal is to test the conclusions drawn from the general arguments along the lines of §4.2 and §4.3 against the known results for ̂Φ given above. It follows ( from ) (4.2.2) and (4.5.2.2) that in order that a b an S-duality transformation generated by takes an arbitrary element of the set B c d to another element of the set B we must have b even. Thus S-duality transformations which preserve the set B take the form: Q → Q ′′ = aQ+bP, P → P ′′ = cQ+dP, a, c, d ∈ Z, b ∈ 2 Z, ad−bc = 1 . (4.5.2.17) On the original variables (ˇρ, ˇσ, ˇv) the associated transformation can be represented by the symplectic matrix (4.2.14). After conjugation by the matrix (4.5.2.14) we get the symplectic matrix acting on the rescaled variables (ˇρ, ˇσ s , ˇv s ): ⎛ d ˜b ⎞ 0 0 ⎜ ˜c a 0 0 ⎟ ⎝ 0 0 a −˜c ⎠ , a,˜b ≡ b/2, d ∈ Z, ˜c ≡ 2c ∈ 2 Z . (4.5.2.18) 0 0 −˜b d This clearly has the form given in (4.5.2.16). Also the periodicities along the (ˇρ, ˇσ s , ˇv s ) directions, as given in (4.5.2.5), are represented by the symplectic transformation ⎛ ⎞ 1 0 ã 1 ã 3 ⎜ 0 1 ã 3 ã 2 ⎟ ⎝ 0 0 1 0 ⎠ , ã 1 ∈ Z, ã 2 , ã 3 ∈ 2 Z . (4.5.2.19) 0 0 0 1 These also are of the form given in (4.5.2.16). Next we turn to the information obtained from the wall crossing relations. Consider first the wall associated with decay (Q, P ) → (Q, 0) + (0, P ), – this controls the behaviour of ̂Φ near ˇv = 0 via eq.(4.3.9). Since Q 2 = −4m and P 2 = 2K can vary independently inside the set A, and since any two charge vectors of the same norm can be related by a T-duality transformation [41], there is no subtlety of the type described below (4.3.11). The inverse of the magnetic partition function φ m entering (4.3.9) is the same as the one that appeared in (4.5.1.7): φ m (ˇρ) = (η(ˇρ)) 24 . (4.5.2.20) The electric partition function gets modified from the corresponding expression given in (4.5.1.7) due to the fact that ( we are only including even Q 2 /2 states. As a result the partition function now becomes 1 η(ˇσ) −24 + η (ˇσ ) ) + 1 −24 . Replacing ˇσ by ˇσ 2 2 s /4 we get φ e (ˇσ) −1 = 1 ( ( )) −24 ˇσs η + 1 ( ( ˇσs η 2 4 2 4 + 1 )) −24 . (4.5.2.21) 2
Page 1:
Counting Microscopic Degeneracy of
Page 5:
Declaration This thesis is a presen
Page 9:
Acknowledgments Firstly I would lik
Page 13 and 14:
Synopsis Black Holes are classical
Page 15:
proposed formula reproduces the kno
Page 19 and 20:
Contents 1 Introduction 1 1.1 Broad
Page 23 and 24:
Chapter 1 Introduction Einstein pro
Page 25 and 26:
1.2. D BRANES 3 specific class of s
Page 27 and 28: 1.3. BRIEF INTRODUCTION TO E 8 × E
Page 29 and 30: 1.4. DEGENERACY OF QUARTER-BPS DYON
Page 31 and 32: 1.5. MOTIVATION AND A BRIEF REVIEW
Page 33 and 34: Chapter 2 Duality orbits, dyon spec
Page 35 and 36: 2.2. T-DUALITY ORBITS OF DYON CHARG
Page 41 and 42: 2.3. AN ALTERNATIVE PROOF 19 Q and
Page 43 and 44: 2.4. PREDICTIONS FOR GAUGE THEORY 2
Page 45 and 46: 2.4. PREDICTIONS FOR GAUGE THEORY 2
Page 47 and 48: Chapter 3 S-duality Action on Discr
Page 49 and 50: This proves the desired result. Nex
Page 51 and 52: For appropriate choice of (α, β,
Page 53 and 54: Chapter 4 Generalities of Quarter B
Page 55 and 56: may not be convergent in another re
Page 57 and 58: 35 where Λ is a large positive num
Page 59 and 60: equiring that we reproduce the blac
Page 61 and 62: 4.1. THE DYON PARTITION FUNCTION 39
Page 63 and 64: 4.2. CONSEQUENCES OF S-DUALITY SYMM
Page 65 and 66: 4.3. CONSTRAINTS FROM WALL CROSSING
Page 73 and 74: 4.5. EXAMPLES 51 Here k is the same
Page 75 and 76: 4.5. EXAMPLES 53 Next we turn to th
Page 77: 4.5. EXAMPLES 55 Let us determine t
Page 81 and 82: 4.5. EXAMPLES 59 uncorrelated and c
Page 83 and 84: 4.5. EXAMPLES 61 is an integer. Sin
Page 85 and 86: 4.5. EXAMPLES 63 1 ((Q−P 2 )/2)2
Page 87 and 88: 4.5. EXAMPLES 65 Finally we turn to
Page 89 and 90: 4.5. EXAMPLES 67 IIB description of
Page 91 and 92: 4.5. EXAMPLES 69 Note that Q and P
Page 93 and 94: 4.5. EXAMPLES 71 On the other hand
Page 95 and 96: 4.6. A PROPOSAL FOR THE PARTITION F
Page 103 and 104: 4.7. REVERSE APPLICATIONS 81 Thus i
Page 105 and 106: 4.7. REVERSE APPLICATIONS 83 For th
Page 107 and 108: Chapter 5 Partition Functions of To
Page 109 and 110: crossing formula for decay into a p
Page 111 and 112: 89 As a consequence of (5.0.19) and
Page 113 and 114: 91 In the primed variables the desi
Page 115 and 116: Furthermore, in order to reproduce
Page 117 and 118: The spectrum in gauge theory contai
Page 119 and 120: Chapter 6 Concluding Remarks Comple
Page 121 and 122: Bibliography [1] J.Polchinski ”Di
Page 123 and 124: BIBLIOGRAPHY 101 [30] M. Stern and
Page 125 and 126: BIBLIOGRAPHY 103 [63] S. Banerjee a
show all

PHYS08200604018 Shamik Banerjee - Homi Bhabha National ...

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?