Lecture Notes for 120 - UCLA Department of Mathematics

More documents

Recommendations

Info

$Math 33B/1 S00 K. Solutions to Midterm Exam G1, Version B If you ...$

$Math 3C Homework 3 Solutions$

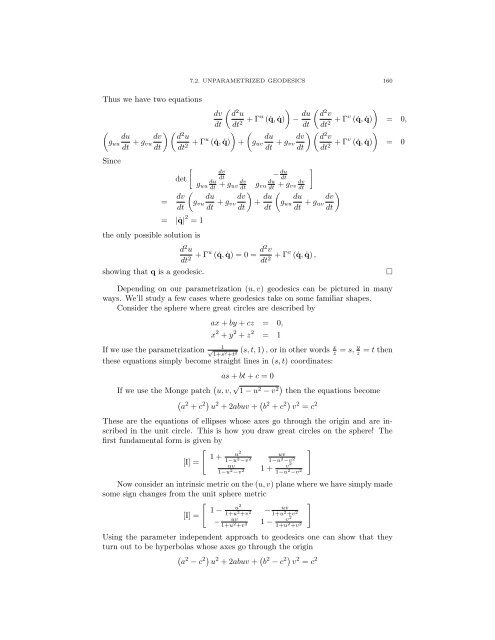
7.2. UNPARAMETRIZED GEODESICS 160 Thus we have two equations ✓ du g uu dt + g vu Since dv dt ◆✓ d 2 u dt 2 + apple det ✓ dv d 2 u dt dt 2 + ◆ u ( ˙q, ˙q) g uu du dv dt = dv ✓ du g vu dt dt + g vv = | ˙q| 2 =1 the only possible solution is d 2 u dt 2 + showing that q is a geodesic. + dt + g uv dv dt dv dt ◆ u ( ˙q, ˙q) ✓ g uv du dt + g vv g vu du ◆ + du dt du dt ✓ du d 2 v dt dt 2 + ◆✓ d 2 v dt 2 + dv dt dt + g vv dv dt ✓ du g uu dt + g uv u ( ˙q, ˙q) =0= d2 v dt 2 + v ( ˙q, ˙q) , ◆ dv dt ◆ v ( ˙q, ˙q) ◆ v ( ˙q, ˙q) = 0, = 0 ⇤ Depending on our parametrization (u, v) geodesics can be pictured in many ways. We’ll study a few cases where geodesics take on some familiar shapes. Consider the sphere where great circles are described by ax + by + cz = 0, x 2 + y 2 + z 2 = 1 If we use the parametrization 1 p 1+s2 +t 2 (s, t, 1) , or in other words x z = s, y z = t then these equations simply become straight lines in (s, t) coordinates: as + bt + c =0 If we use the Monge patch u, v, p 1 u 2 v 2 then the equations become a 2 + c 2 u 2 +2abuv + b 2 + c 2 v 2 = c 2 These are the equations of ellipses whose axes go through the origin and are inscribed in the unit circle. This is how you draw great circles on the sphere! The first fundamental form is given by " u 1+ 2 [I] = # uv 1 u 2 v 2 1 u 2 v 2 1 u 2 v 2 uv v 1 u 2 v 1+ 2 2 Now consider an intrinsic metric on the (u, v) plane where we have simply made some sign changes from the unit sphere metric " [I] = # u 1 2 uv 1+u 2 +v 2 1+u 2 +v 2 uv v 1+u 2 +v 1 2 2 1+u 2 +v 2 Using the parameter independent approach to geodesics one can show that they turn out to be hyperbolas whose axes go through the origin a 2 c 2 u 2 +2abuv + b 2 c 2 v 2 = c 2
7.4. CONSTANT GAUSS CURVATURE 161 This metric can also be reparametrized to have its geodesics be straight lines. The later reparametrization is: u s = p 1+u2 + v 2 t = v p 1+u2 + v 2 and the geodesics given by as + bt + c =0. We shall later explicitly find the geodesics on the upper half plane using the equations developed here. Exercises. (1) Show that geodesics satisfy a second order equation of the type d 2 ✓ ◆ 3 ✓ ◆ 2 v dv dv du 2 = A + B + C dv du du du + D and calculate the functions A, B, C, D in terms of the appropriate Christoffel symbols and metric coefficients. 7.3. Shortest Curves The goal is to show that the shortest curves are geodesics, and conversely that sufficiently short geodesics are minimal in length. For the latter using geodesic coordinates makes an argument that is similar to the Euclidean version using a unit gradient field. There should be an easy extrinsic proof that the shortest curves are geodesics 7.4. Constant Gauss Curvature The goal will be to give a canonical local structure for surfaces with constant Gauss curvature. Given the plethora of surfaces with constant Gauss curvature we seek for the moment only canonical coordinates. Minding was the first person to give the classification of the first fundamental form we obtain below. Riemann extended this result to higher dimensions. We start by studying the case of vanishing Gauss curvature. Theorem 7.4.1. If a surface has zero Gauss curvature, then it admits Cartesian coordinates. Proof. We shall assume that we have geodesic coordinates along a unit speed geodesic. Thus the v-curve described by u =0is a geodesic, and by definition of geodesic coordinates all of the u-curves are unit speed geodesics. The first fundamental form is apple 1 0 [I] = 0 r 2 Assuming K =0, we immediately obtain r (u, v) =r (0,v)+u @r @u (0,v) But r (0,v)= @q @v =1
Page 1:
Classical Differential Geometry Pet
Page 4 and 5:
CONTENTS 4 Chapter 7. Other Topics
Page 6 and 7:
1.1. CURVES 6 measurements are need
Page 8 and 9:
1.1. CURVES 8 as we then get q (✓
Page 10 and 11:
1.1. CURVES 10 these derivatives ar
Page 12 and 13:
1.1. CURVES 12 (a) Show that if r (
Page 14 and 15:
1.2. ARCLENGTH AND LINEAR MOTION 14
Page 16 and 17:
Page 18 and 19:
Page 20 and 21:
1.3. CURVATURE 20 relates the side
Page 22 and 23:
1.3. CURVATURE 22 of a that is norm
Page 24 and 25:
1.3. CURVATURE 24 As the piece of t
Page 26 and 27:
1.4. INTEGRAL CURVES 26 (14) Let q
Page 28 and 29:
1.4. INTEGRAL CURVES 28 So at q 0 =
Page 30 and 31:
CHAPTER 2 Planar Curves 2.1. Genera
Page 32 and 33:
2.2. THE FUNDAMENTAL EQUATIONS 32 M
Page 34 and 35:
2.2. THE FUNDAMENTAL EQUATIONS 34 (
Page 36 and 37:
2.3. LENGTH AND AREA 36 (22) (Newto
Page 38 and 39:
As ( 2.3. LENGTH AND AREA 38 sin
Page 40 and 41:
2.3. LENGTH AND AREA 40 The choice
Page 42 and 43:
2.4. THE ROTATION INDEX 42 the hypo
Page 44 and 45:
2.4. THE ROTATION INDEX 44 where an
Page 46 and 47:
2.4. THE ROTATION INDEX 46 Exercise
Page 48 and 49:
2.5. TWO SURPRISING RESULTS 48 unit
Page 50 and 51:
2.6. CONVEX CURVES 50 Exercises. Po
Page 52 and 53:
2.6. CONVEX CURVES 52 Proof. Any cu
Page 54 and 55:
2.6. CONVEX CURVES 54 (9) Give an e
Page 56 and 57:
3.1. THE FUNDAMENTAL EQUATIONS 56 T
Page 58 and 59:
3.1. THE FUNDAMENTAL EQUATIONS 58 B
Page 60 and 61:
3.1. THE FUNDAMENTAL EQUATIONS 60 (
Page 62 and 63:
3.2. CHARACTERIZATIONS OF SPACE CUR
Page 64 and 65:
3.2. CHARACTERIZATIONS OF SPACE CUR
Page 66 and 67:
3.3. CLOSED SPACE CURVES 66 (12) Pr
Page 68 and 69:
3.3. CLOSED SPACE CURVES 68 Thus th
Page 70 and 71:
3.3. CLOSED SPACE CURVES 70 (b) Sho
Page 72 and 73:
CHAPTER 4 Basic Surface Theory 4.1.
Page 74 and 75:
4.1. SURFACES 74 Definition 4.1.6.
Page 76 and 77:
4.1. SURFACES 76 (6) Many classical
Page 78 and 79:
4.2. TANGENT SPACES AND MAPS 78 Thi
Page 80 and 81:
4.2. TANGENT SPACES AND MAPS 80 We
Page 82 and 83:
4.2. TANGENT SPACES AND MAPS 82 Def
Page 84 and 85:
4.3. THE ABSTRACT FRAMEWORK 84 Befo
Page 86 and 87:
4.3. THE ABSTRACT FRAMEWORK 86 in o
Page 88 and 89:
4.4. THE FIRST FUNDAMENTAL FORM 88
Page 90 and 91:
Page 92 and 93:
Page 94 and 95:
4.5. SPECIAL MAPS AND PARAMETRIZATI
Page 96 and 97:
4.5. SPECIAL MAPS AND PARAMETRIZATI
Page 98 and 99:
4.6. THE GAUSS FORMULAS 98 and then
Page 100 and 101:
CHAPTER 5 The Second Fundamental Fo
Page 102 and 103:
5.1. CURVES ON SURFACES 102 This sh
Page 104 and 105:
5.1. CURVES ON SURFACES 104 We also
Page 106 and 107:
5.1. CURVES ON SURFACES 106 Exercis
Page 108 and 109:
5.2. THE GAUSS AND WEINGARTEN MAPS
Page 110 and 111: 5.2. THE GAUSS AND WEINGARTEN MAPS
Page 112 and 113: 5.3. THE GAUSS AND MEAN CURVATURES
Page 118 and 119: 5.4. PRINCIPAL CURVATURES 118 The f
Page 120 and 121: 5.4. PRINCIPAL CURVATURES 120 By le
Page 122 and 123: 5.5. RULED SURFACES 122 (16) Let q
Page 124 and 125: 5.5. RULED SURFACES 124 Definition
Page 126 and 127: i.e., d dv and X are parallel. In p
Page 128 and 129: 5.5. RULED SURFACES 128 This shows
Page 130 and 131: 5.5. RULED SURFACES 130 (b) Show th
Page 132 and 133: 5.5. RULED SURFACES 132 (e) Show th
Page 134 and 135: 6.1. CALCULATING CHRISTOFFEL SYMBOL
Page 136 and 137: 6.1. CALCULATING CHRISTOFFEL SYMBOL
Page 138 and 139: 6.2. GENERALIZED AND ABSTRACT SURFA
Page 140 and 141: 6.3. ACCELERATION 140 Exercises. (1
Page 142 and 143: 6.3. ACCELERATION 142 Alternately t
Page 144 and 145: 6.4. THE GAUSS AND CODAZZI EQUATION
Page 150 and 151: 6.5. LOCAL GAUSS-BONNET 150 (b) Use
Page 152 and 153: 6.5. LOCAL GAUSS-BONNET 152 We furt
Page 154 and 155: 6.5. LOCAL GAUSS-BONNET 154 clockwi
Page 156 and 157: 6.5. LOCAL GAUSS-BONNET 156 where t
Page 158 and 159: 7.1. GEODESICS 158 we must solve a
Page 162 and 163: 7.5. ISOMETRIES 162 since the v-cur
Page 164 and 165: 7.6. THE UPPER HALF PLANE 164 Proof
Page 166 and 167: 7.6. THE UPPER HALF PLANE 166 and t
Page 168 and 169: 7.6. THE UPPER HALF PLANE 168 so so
Page 170 and 171: 8.2. RIEMANNIAN GEOMETRY 170 The ke
Page 172 and 173: APPENDIX A Vector Calculus A.1. Vec
Page 174 and 175: A.2. GEOMETRY 174 the characteristi
Page 176 and 177: A.4. DIFFERENTIAL EQUATIONS 176 The
Page 178 and 179: A.4. DIFFERENTIAL EQUATIONS 178 Nex
Page 180 and 181: APPENDIX B Special Coordinate Repre
Page 182 and 183: This leaves us with finding L s s.
Page 184 and 185: B.3. MONGE PATCHES 184 So we immedi
Page 186 and 187: B.4. SURFACES GIVEN BY AN EQUATION
Page 188 and 189: B.6. CHEBYSHEV NETS 188 (1) Show th
Page 190: B.7. ISOTHERMAL COORDINATES 190 K =
show all

Lecture Notes for 120 - UCLA Department of Mathematics

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?